Non-closed geodesics on a convex polyhedron in $\mathbb{R}^3$

Question

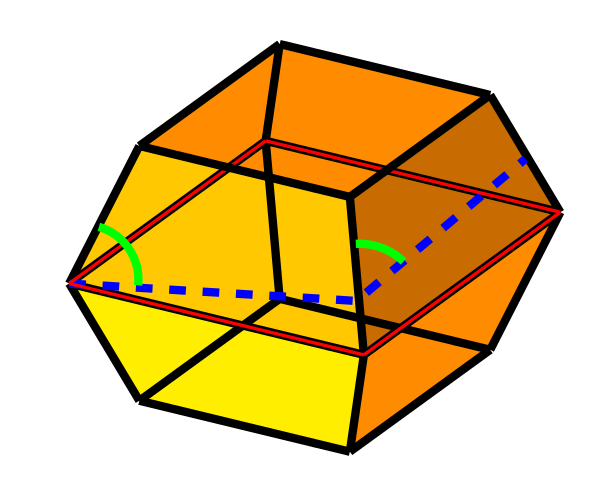
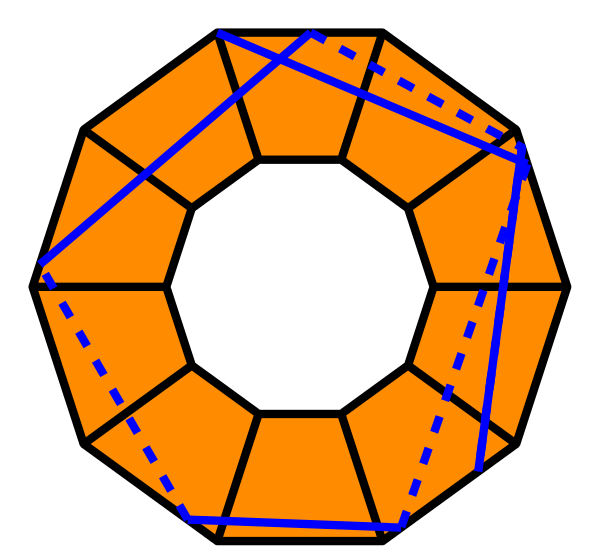
Let $P$ be the surface of a closed convex polyhedron in $\mathbb{R}^3$.

Q. Does every non-closed geodesic $\gamma$ fill $P$ densely?

Of course $\gamma$ cannot pass through a vertex of $P$, but it could come arbitrarily close.

Here is an informal way to phrase the question. One can have non-closed geodesics on smooth convex surfaces in $\mathbb{R}^3$ that do not fill the surface. E.g., on ellipsoids, as in the MO question "Surfaces filled densely by a geodesic":

---

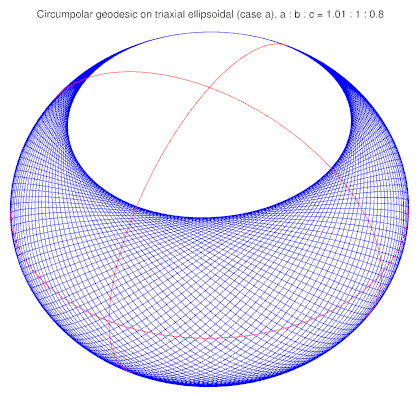
            ^{(Image from GeographicLib.)}

---

So: Is there a polyhedral analog?

(One could ask the same question for nonconvex polyhedra.)

Answer 1

A simple triangulation of the previous example will work.

Suppose we have a geodesic like the blue one. The key point is to look at the green angles (better the complementary ones, those respect to the horizontal plane). Each time the geodesic crosses a black edge, this angle decreases by a fixed (this is essential!) amount, namely the angle formed by two black vertical edges if extended. If the initial angle is small enough, then we may make sure that the geodesic will cross so many black edges as we want before it touches the top square, so in fact it is not touched at all. The blue geodesic goes down and the process goes on forever.

One could answer: what happens if, when coming down, the angle is very large? For this not to happen, the polyhedron must be pointed enough. This is always feasible, as shown by this picture (which represents a periodic plane representation of the lateral faces):

Of course non-periodicity is a generally satisfied.