6
$\begingroup$

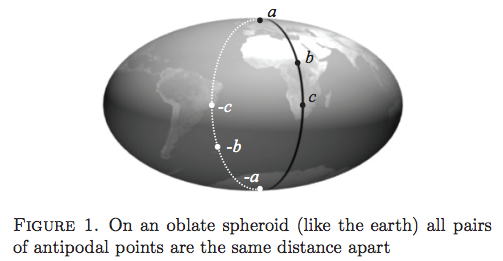
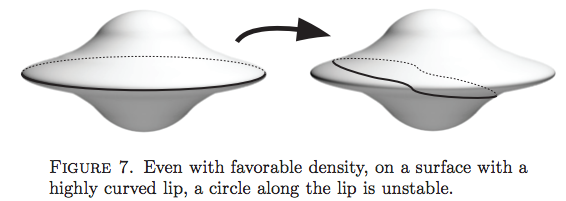
Let $S$ be a smooth convex surface in $\mathbb{R}^3$ (although my question may as well be asked for the surface of a polyhedron). Say that $\gamma$ is a shortest halving curve if (a) it partitions the surface area of $S$ into two equal-area halves, and (b) it is the shortest such curve (under the Euclidean metric).

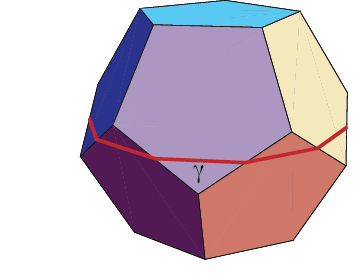
  DodecaGeodesic

Q. Is $\gamma$ necessarily a simple (non-self-intersecting) closed geodesic on $S$?

On a polyhedral surface, the equivalent would be a simple closed quasigeodesic (in Alexandrov's sense).

Addendum. Douglas Zare's counterexample to that vain hope:
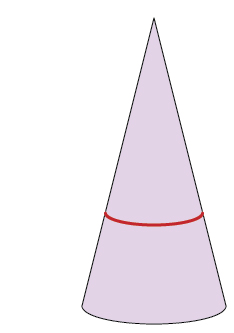
BisectCone
                      (Not metrically accurate.)
The red circle seems to be a shortest halving curve, but it is not a geodesic.

Improve this question
$\endgroup$
3
  • 4
    $\begingroup$ If you smooth out a tall cone, I think you get a counterexample. $\endgroup$
    – Douglas Zare
    Commented Jul 27, 2014 at 1:13
  • 4
    $\begingroup$ Poincare remarked that if you take a shortest curve that halves the curvatura integra in a convex surface you do get a closed geodesic. The full details were worked out much later, but I forget by who! It'll come back ... $\endgroup$
    – alvarezpaiva
    Commented Jul 27, 2014 at 12:07
  • $\begingroup$ @alvarezpaiva: Relatedly, the Gauss-Bonnet theorem implies that on a genus-zero surface, a closed geodesic must partition the curvature $2\pi + 2\pi$. $\endgroup$
    – Joseph O'Rourke
    Commented Jul 27, 2014 at 13:31

2 Answers 2

Reset to default
4
$\begingroup$

Look at it from a calculus of variations perspective. A geodesic is locally a distance-minimizing curve - that is, an infinitesimal change in the curve between two points, which keeps those two points constant, cannot decrease the length.

Your curve is globally a distance minimizing curve among curves with a fixed area on one side. Using Lagrange multipliers, we can see that the curve minimizes the sum of the length plus some constant multiple of the area on one side. If that constant multiple is zero, you obtain a geodesic. If not, you don't.

Solving the minimization problem for a given constant gives a differential equation. I haven't done the computation, but I would guess that, if a geoedesic represents travelling in a straight line on the surface, then this equation represents travelling with a constant rate of turning.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Another example that may be helpful is to round out a flat disc, then enlarge a small circle on one side of the disc to a bubble, increasing the area on that side. Then the length-minimizing curve that divides the area evenly will be a length-minimizing curve in flat space containing a given area - that is, a circle. $\endgroup$
    – Will Sawin
    Commented Jul 27, 2014 at 2:08
  • 1
    $\begingroup$ I think this calculus of variations perspective makes clear that the more natural question is about curves with a fixed area to one side, where the area on the other side may be different (and even infinite). $\endgroup$
    – Allen Knutson
    Commented Jul 27, 2014 at 3:25
  • $\begingroup$ @AllenKnutson Yes, and that's a more elegant way of connecting this problem to the isoperimetric inequality than my example. $\endgroup$
    – Will Sawin
    Commented Jul 27, 2014 at 20:30
3
$\begingroup$

I just located this relevant reference:

Engelstein, Max, Anthony Marcuccio, Quinn Maurmann, and Taryn Pritchard. "Isoperimetric problems on the sphere and on surfaces with density." New York J. Math 15 (2009): 97-123. (PDF download link)

"the short equator gives a least perimeter partition of the ellipsoid into two regions of equal area"

  EllipsoidHalved
Note the similarity in Fig.7 below to Will Sawin's disc-bubble (although making a different point):
      FlyingSaucer

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .