Which smooth, closed surfaces $S \subset \mathbb{R}^3$ have no single geodesic $\gamma$ that fills $S$ densely?
Say a geodesic $\gamma$ "fills $S$ densely" if the closure of the set of points through which $\gamma$ passes equals $S$. Some examples:
- A sphere: every geodesic is a great circle.
- Zoll surfaces, as discussed here: "Surfaces all of whose geodesics are both closed and simple."
- An ellipsoid.
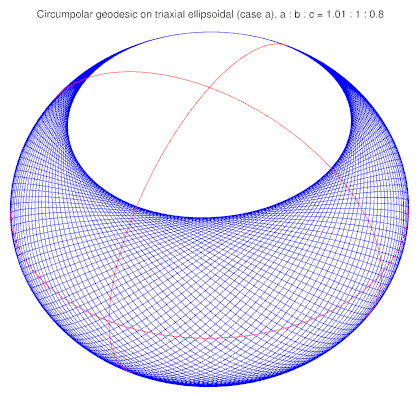
(Image from GeographicLib.)
-
A torus generally has many geodesics that fill the surface.
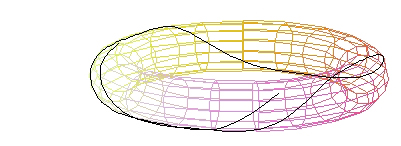
(Image by John Oprea)
My assumption is that almost all surfaces have geodesics that fill them. Is this known, under any interpretation of "almost all"? I would also be interested in extending the list of exceptional surfaces beyond {sphere, Zoll, ellipsoid}. Thanks for pointers!
Answers Summary (18Apr2013):
- (Robert Bryant, Mikhail Katz) Any surface of revolution with poles has no dense geodesic. This holds for convex or nonconvex surfaces of revolution.
- (Robert Bryant) There are generalizations of Liouville surfaces (due to Goryachev-Chaplygin and to Dullin-Matveev) that have no dense geodesic.
- (Misha Kapovich) Every surface may be perturbed by gluing on "focusing caps" so that it has dense geodesics.
- (Keith Burns) Guess: There is always a dense geodesic on a closed Riemannian surface of genus $\ge 2$.
