Generalizing Bottema's theorem

Question

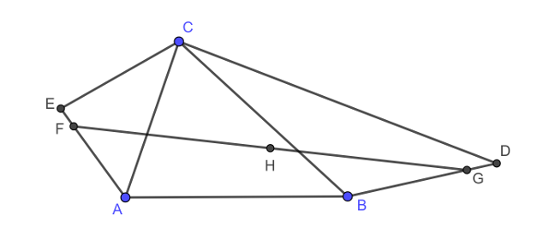
Can you provide another proof for the claim given below?

Claim. In any triangle $\triangle ABC$ construct triangles $\triangle ACE$ and $\triangle BDC$ on sides $AC$ and $BC$ such that $\frac{AE}{AC}=\frac{BD}{BC}$ and $\angle EAC+\angle CBD=180^{\circ}$ hold true. Let points $F$ and $G$ divide sides $AE$ and $BD$ respectively in the same arbitrary ratio . The midpoint $H$ of the line segment that connects points $F$ and $G$ is independent of the location of $C$ .

Proof.(Complex numbers) Consider $A$, $B$ , $C$ as complex numbers and choose a $\lambda \in \mathbb{R}$. Denote $\angle EAC=\alpha$ , $\angle CBD=\beta$ and $\frac{AE}{AC}=\frac{BD}{BC}=k$ . Then, $$F=A+\lambda(E-A)=A+\lambda \cdot k(\cos \alpha +i \sin \alpha)(C-A)$$ $$G=B+\lambda(D-B)=B+\lambda \cdot k(\cos (-\beta) +i \sin (-\beta))(C-B)$$ $$H=\frac{1}{2}(F+G)=\frac{1}{2}(A+\lambda \cdot k(\cos \alpha +i \sin \alpha)(C-A)+$$$$B+\lambda \cdot k(-\cos \alpha -i \sin \alpha)(C-B))=$$ $$\frac{1}{2}(A+\lambda \cdot k(\cos \alpha + i\sin \alpha)C-\lambda \cdot k(\cos \alpha + i\sin \alpha)A+$$$$B-\lambda \cdot k(\cos \alpha + i\sin \alpha)C+\lambda \cdot k(\cos \alpha + i\sin \alpha)B)=$$ $$\frac{1}{2}(A(1-\lambda \cdot k(\cos \alpha + i\sin \alpha))+B(1+\lambda \cdot k(\cos \alpha + i\sin \alpha)))$$ This shows that $H$ is independent of the location of $C$.

Q.E.D.

The GeoGebra applet that demonstrates this claim can be found here.

Answer 1

This is essentially the same proof but it is a bit simplified and provides a more precise statement. We suppose that $A=0$, $B=1$, $C=z$. Then $E=\rho e^{i\alpha} z$ and $D=1+\rho e^{i\beta}(z-1)$ (so we are making no a priori assumptions about the swing angles). Then $F=\lambda \rho z e^{i\alpha}$ and $G=\lambda \rho ze^{-i\beta}$ plus a term which is constant (i.e., independent of $z$) and so irrelevant in this context. By the way, replacing $E$ and $D$ by $F$ and $G$ is a red herring.

From this we immediately get the following refined version. The midpoint $H$ is independent of $z$ if and only if the swing angles are related as in the OP. One can also extend to the case where $H$ is a suitable point on the perpendicular bisector of $FG$ and this version is sharp (i.e., it only holds for such points on the bisector).