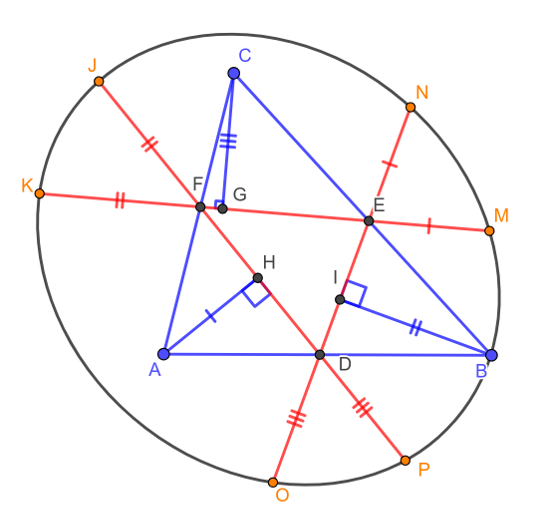
Can you prove the following proposition:
Proposition. Given an arbitrary triangle $\triangle ABC$. Let $D,E,F$ be the points on the sides $AB$,$BC$ and $AC$ respectively , such that $\frac{AB}{DA}=\frac{BC}{EB}=\frac{AC}{FC}=k$, where $k$ is an arbitrary ratio. Let $G,H,I$ be the points on the line segments $EF$,$DF$ and $DE$ respectively such that $CG \perp EF$ , $AH \perp DF$ and $BI \perp DE$. Now let $M$ be the point on the extension of the segment $EF$ beyond $E$ such that $EM=AH$. Similarly, define the points $N,J,K,O,P$ so that the point $N$ lies on the extended segment $DE$ and $EN=AH$ , the point $J$ lies on the extended segment $DF$ and $FJ=BI$ , the point $K$ lies on the extended segment $EF$ and $FK=BI$ , the point $O$ lies on the extended segment $DE$ and $DO=CG$ and the point $P$ lies on the extended segment $DF$ and $DP=CG$ . I claim that the points $J,K,M,N,O,P$ lie on an ellipse.
The GeoGebra applet that demonstrates this proposition can be found here.