A generalization of the law of tangents

Question

The law of tangents is a statement about the relationship between the tangents of two angles of a triangle and the lengths of the opposing sides.

Let $a$, $b$, and $c$ be the lengths of the three sides of a triangle, and $\alpha$, $\beta$ and $\gamma$ be the angles opposite those three respective sides. The law of tangents states that

$$\frac{\tan\frac12(\alpha-\beta)}{\tan\frac12(\alpha+\beta)}=\frac{a-b}{a+b}.\tag{1}\label{1}$$

The law of tangents can be used in any case where two sides and the included angle, or two angles and a side, are known.

Although Viète gave us the modern version of the law of tangents, it was Fincke who stated the law of tangents for the first time and also demonstrated its application by solving a triangle when two sides and the included angle are given (see Wu - The Story of Mollweide and Some Trigonometric Identities).

A proof of the law of tangents is provided by Wikipedia (see here).

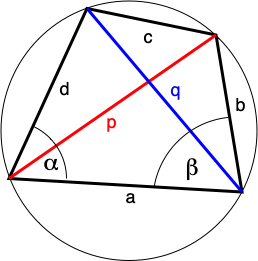
Generalization. Let $a$, $b$, $c$ and $d$ be the sides of a cyclic convex quadrilateral. Let $\angle{DAB}=\alpha$ and $\angle{ABC}=\beta$, then the following identity holds

$$\frac{\tan\frac12(\alpha-\beta)}{\tan\frac12(\alpha+\beta)}=\frac{(a-c)(b-d)}{(a+c)(b+d)}.\tag{2}\label{2}$$

Proof. Using the sum-to-product formulas we can rewrite the left-hand side of $(2)$ as follows

$$\frac{\tan\frac12(\alpha-\beta)}{\tan\frac12(\alpha+\beta)}=\frac{\sin\frac12(\alpha-\beta)\cos\frac12(\alpha+\beta)}{\cos\frac12(\alpha-\beta)\sin\frac12(\alpha+\beta)}=\frac{\sin{\alpha}-\sin{\beta}}{\sin{\alpha}+\sin{\beta}}.$$

The area of a cyclic quadrilateral can be expressed as $\Delta=\frac12(ad+bc)\sin{\alpha}$ (see $(12)$ at Killing three birds with one stone) and similarly for the other angles. Then substituting, simplifying and factorizing we have

$$\begin{align*}\frac{\tan\frac12(\alpha-\beta)}{\tan\frac12(\alpha+\beta)}&=\frac{\frac{2\Delta}{ad+bc}-\frac{2\Delta}{ab+cd}}{\frac{2\Delta}{ad+bc}+\frac{2\Delta}{ab+cd}}=\frac{ab-ad+cd-bc}{ab+ad+cd+bc}=\frac{(a-c)(b-d)}{(a+c)(b+d)}\end{align*}.$$

$\square$

The formula \eqref{2} reduces to the law of tangents for a triangle when $c=0$.

A related result can be found at A generalization of Mollweide's Formula (rather Newton's).

Crossposted at MathSE.

Question: Is this generalization known?

A historical correction: Fincke was not the first to publish the law of tangents. It was actually Ibn Muadh who first described it in the 11th century. See the discussion at HSMSE.

Answer 1

To place the formula of the OP into context, it is helpful to note the identity (from Josefsson - More characterizations of cyclic quadrilaterals)

$$\frac{q-p}{q+p}=\frac{(a-c)(b-d)}{(a+c)(b+d)}.\tag{$*$}\label{star}$$ Here $q$ and $p$ are the lengths of the blue and red diagonals, with opposite angles $\alpha$ and $\beta$.

The quadrilateral tangent formula can thus be rewritten in a form that looks more like the triangle tangent formula, $$\frac{\tan\frac12(\alpha-\beta)}{\tan\frac12(\alpha+\beta)}=\frac{q-p}{q+p}.$$

Q: Is it obvious that the law of tangents applies to the blue and red diagonals?

---

_{The formula \eqref{star} is equivalent (upon rearrangement of the terms) to what is known as Ptolemy’s second theorem $$\frac{p}{q}=\frac{ad+bc}{ab+cd}.$$}

Answer 2

This is a comment but will be too long. Your formulae are examples of the generalised Heron principle—every triangle quantity is expressible in terms of the side lengths $a,b,c$. I won’t give a formal definition of atriangle function—essentially one defined on the triple $A,B,C$ of the vertices which is invariant under congruence and can be computed from their coordinates.

This fact is easily deduced by the $p,q$ method. We choose coordinates so that $A=(0,0)$, $B=(c,0)$ and $C=(p,q)$. We then use the equations $a^2=(p-c)^2+q^2$, $b^2=p^2+q^2$ to express $p$ and $q$ in terms of the three side lengths. This can easily be done by hand. One gets two solutions, corresponding to the fact that the side lengths fail to determine orientation. For a given triangle quantity, one then computes it for the above triangle in terms of $p$, $q$ and $c$ and then applies this substitution (for example $A=\frac 1 2 q c$ give the original Heron formula).

I have used this method to obtain your results. I hope this unified approach to them and many others will be of interest.

Edit in respose to a negative comment. Obviously, the same method can be applied to quadrilaterals. One assumes that the vertices are $(0,0)$, $(a,0)$, $(p_1,q_1)$ and $(p_2,q_2)$ (I am using the natural notion for the side lengths going round cyclically). One can then express every quadrilateral quantity in terms of $a$ and the $p$’s and $q$’s and use this to check the identities (since quadrilaterals are not rigid, one cannot use the side lengths as parameters).

Danke für die Belehrung but I am perfectly aware of the rules of the site. Since it is highly unlikely that I will ever gain enough points to post a comment, I have the options of remaining silent or acting as I did. As I tried to point out, I have chosen the latter course because I had hoped that my “comment” might be of interest to the OP.