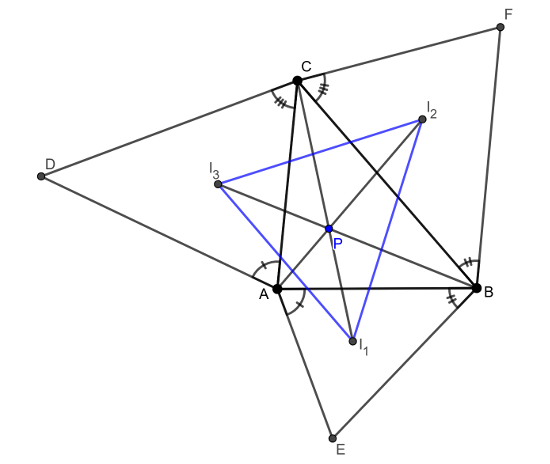
Can you provide a proof for the following proposition?
Proposition. Given an arbitrary $\triangle ABC$. The $\triangle AEB$, $\triangle BFC$ and $\triangle CDA$ are constructed on the sides of the $\triangle ABC$ either all outward or all inward, such that $\angle DAC=\angle BAE$, $\angle EBA= \angle CBF$, $\angle FCB= \angle ACD$ and $\angle DAC+\angle EBA+\angle FCB=180^{\circ}$. Let $I_1$, $I_2$, $I_3$ be the incenters of the $\triangle AEB$, $\triangle BFC$, $\triangle CDA$, respectively. Then the angles of the $\triangle I_1I_2I_3$ are equal to $\angle I_3I_1I_2=(\angle BAE+\angle EBA)/2$, $\angle I_1I_2I_3=(\angle CBF+\angle FCB)/2$ and $\angle I_2I_3I_1=(\angle ACD+ \angle DAC)/2$.
GeoGebra applet that demonstrates this proposition can be found here.