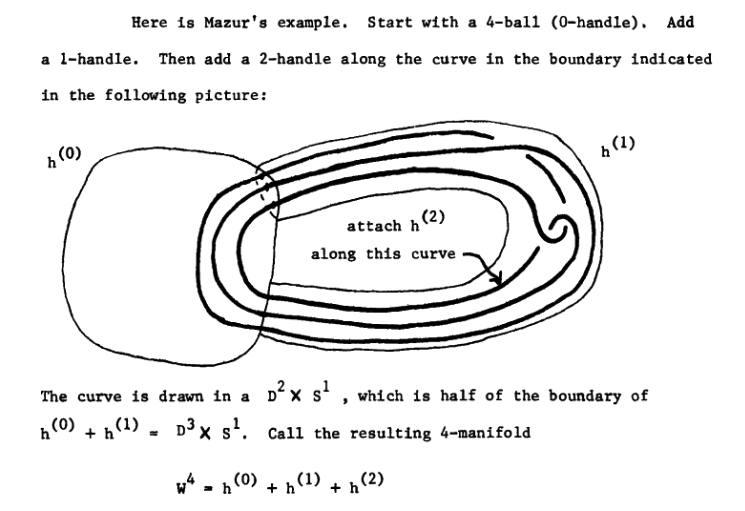
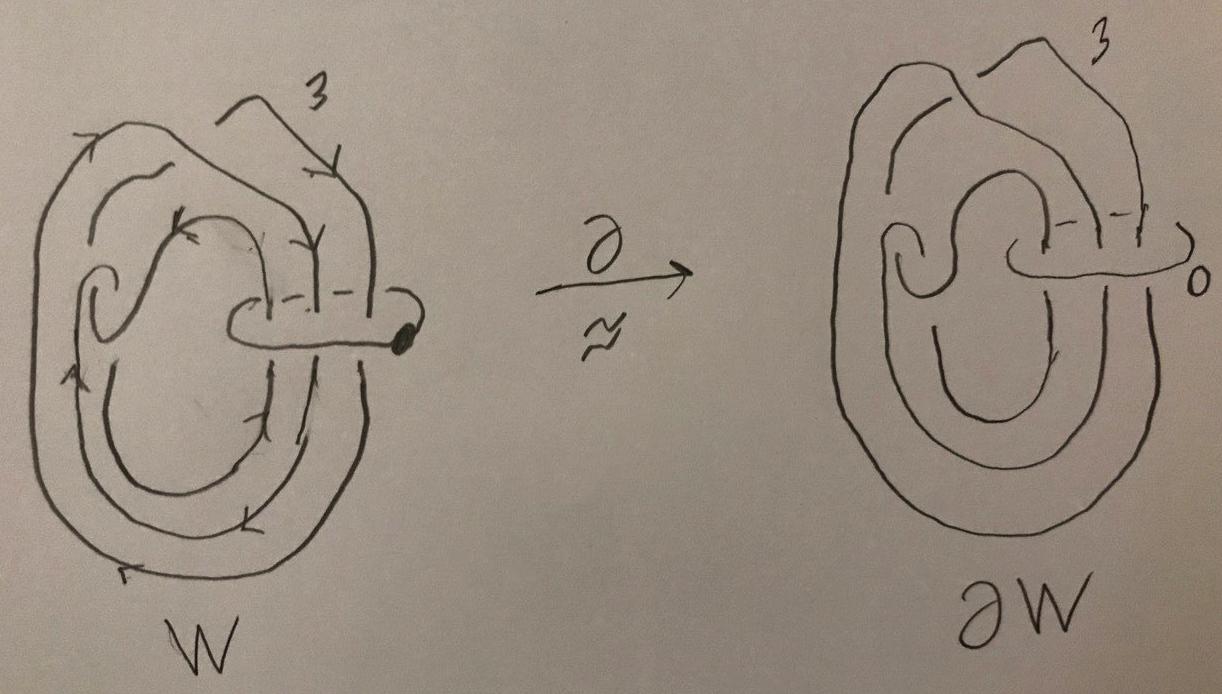6
$\begingroup$

If we attach a $4$-dimensional $1$-handle $D^1 \times D^3$ to a $4$-dimensional $0$-handle $B^4$, we obtain $S^1 \times D^3$. The null homologous knot in $S^1 \times S^2$ indicated in the picture lives in a solid torus/attaching region $S^1 \times D^2$ and $$ S^1 \times D^2 \hookrightarrow S^1 \times S^2 \hookrightarrow S^1 \times D^3.$$

In pg. 356 of his book Knots and Links, Dale Rolfsen notes that

  1. $\pi_1(W) = 1$,
  2. $\pi_1(\partial W) = \langle x,y \ \vert \ y^5 = x^7, y^4 = x^2 y x^2 \rangle \neq 1$.

How we compute the relevant fundamental groups using the following diagram? What is the strategy?

enter image description here

Improve this question
$\endgroup$
6
  • 5
    $\begingroup$ Handle attachments are basically "structured" cw-complexes. There is a standard process to compute the fundamental group of CW-complexes described in algebraic topology textbooks. For the boundary there are a few ways. One would be to start with the Wirthinger presentation of the link exterior, then attach the appropriate relators as one fills. I think Rolfsen explains this, a little earlier in his book. $\endgroup$
    – Ryan Budney
    Commented Feb 19, 2022 at 0:19
  • 2
    $\begingroup$ I don't understand. We can apply the Wirtinger's method to the knots in $S^3$. In OP, knots live in $S^1 \times S^2$. Is there a way generalize this process to another $3$-manifolds? $\endgroup$
    – Max Schumann
    Commented Feb 19, 2022 at 10:46
  • 1
    $\begingroup$ Yes, $S^1 \times S^2$ you can obtain as $0$-surgury on the unknot, so your surgury diagram for $\partial W$ will consist of a union of the surgery curves in your diagram with a $0$-labelled unknot. Going around the $S^1$ factor of $S^1 \times S^2$ corresponds to linking the unknot. $\endgroup$
    – Ryan Budney
    Commented Feb 19, 2022 at 19:57
  • $\begingroup$ Great! To be hundred percent sure, would you sketch a diagram, please? $\endgroup$
    – Max Schumann
    Commented Feb 19, 2022 at 23:01
  • $\begingroup$ Plus, do we generalize your technique to any surgered $3$-manifold, or $S^1 \times S^2$ is special here? Do you have a reference (providing proofs) for generalizing process of Wirtinger presentations? $\endgroup$
    – Max Schumann
    Commented Feb 19, 2022 at 23:03

1 Answer 1

Reset to default
7
$\begingroup$

Thanks to the dotted circle notation of Professor Akbulut, we can easily (compare with your picture) draw the handle diagram of a typical Mazur manifold as follows:

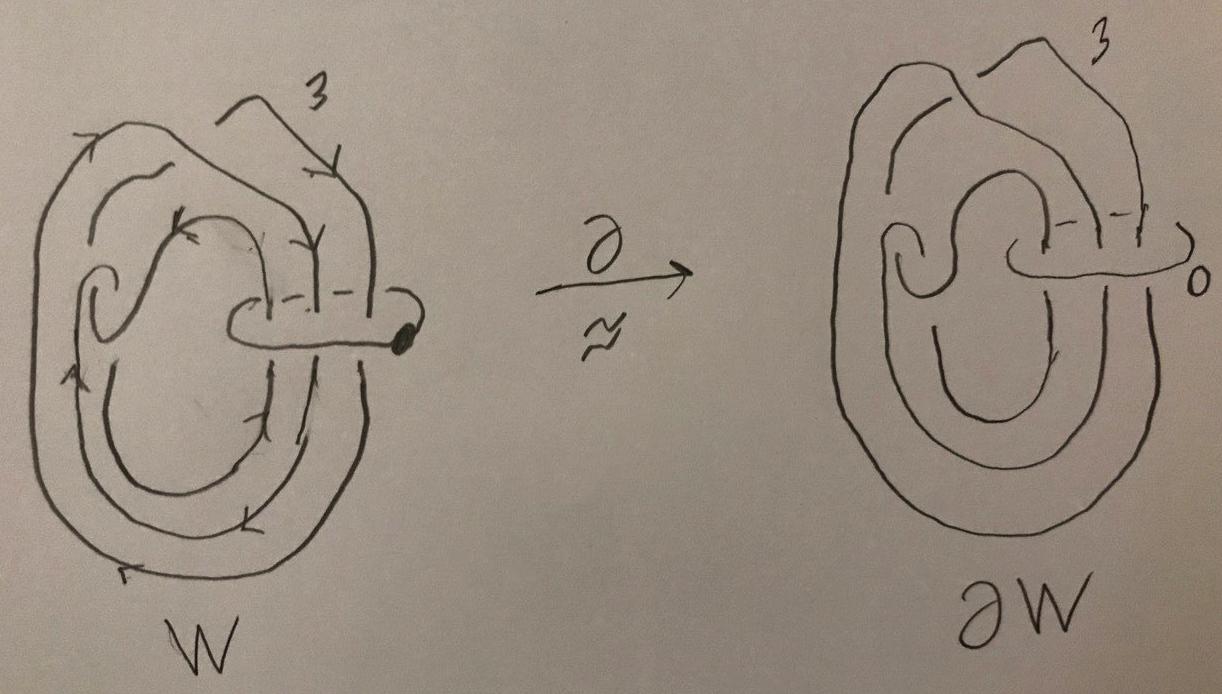
Mazur

Since $1$-handles and $2$-handles respectively give the generators and relations of the fundamental group of a $4$-manifold, we have (for my $W$): $$\pi_1(W) = \langle \alpha \ \vert \ \alpha^2 \alpha^{-1} \rangle = 1.$$

The boundary $3$-manifold $\partial W$ is already in the picture. Further, we can compute $\pi_1(\partial W)$ from the diagram by using Wirtinger's presentation.

Because today is Sunday (which makes me so lazy) and Eylem already did such a computation (another purpose but for a Mazur manifold) in Example 1 in [Yıldız, 2018], the rest is for you, mimic the argument by keeping Ryan Budney's comments in your mind:

Eylem

Bonus: In particular, $W$ is a contractible $4$-manifold: By using Mayer-Vietoris sequences, observe that $W$ is a homology $4$-ball, i.e., we have $H_*(W, \mathbb{Z}) = H_*(B^4, \mathbb{Z})$. Then apply the classical theorems of Hurewicz and Whitehead.

Bonus 2: Since $W$ is contractible, $\partial W$ must be a homology $3$-sphere, i.e., $H_*(\partial W, \mathbb{Z}) = H_*(S^3, \mathbb{Z})$. Using Kirby calculus, show that $\partial W \approx \Sigma(2,5,7)$ where $\Sigma(p,q,r)$ denotes the Brieskorn sphere given coprime positive integers $p,q$ and $r$.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .