arc length of a knot in the solid torus

Question

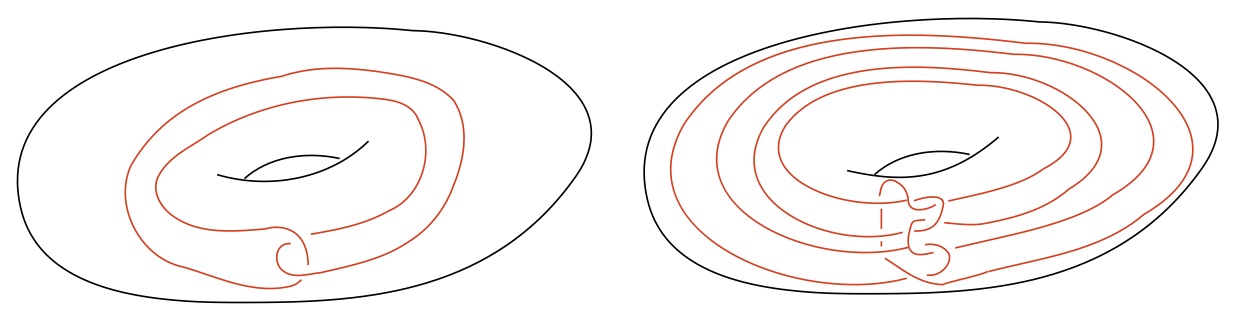
As motivation, consider the knot in the solid torus in the first (left) picture below. Put a metric on the torus -- for concreteness, let's assume it's induced by the standard euclidean metric on $[0,1]^3$ (identify $\{0\} \times [0,1]^2$ and $\{1\} \times [0,1]^2$ to build the torus), so that any non-isotopically trivial curve has length at least 1. It seems pretty clear to me that the infimal arc length of a curve that represents this knot is 2.

The second picture is a more complicated example, where the infimal length is (maybe?) 4. (if I've goofed up on the drawing, hopefully you believe there is a way to make such an example)

Is there an invariant (perhaps coming from thinking of these as links in $S^3$ rather than knots in the torus) that gives a lower bound on length?

There is an obvious way to give a lower bound on length of a curve in the solid torus by considering it as an element of $\pi_1$ of the solid torus. However, both of these examples are trivial in $\pi_1$, so I'm looking for something that sees the knotting!

Ideally, we'll get a lower bound on length, and an easy-to-describe family of knots that are trivial in $\pi_1$, but where infimal knot length goes to infinity.

Answer 1

I think this is just the minimal geometric intersection number with a meridian disk.

Suppose one has a meridian disk $D$ intersecting the knot in $k$ points. Then one can find an infimum of the knot lengths approaching $k$. Just shrink the knot down into a small cylindrical torus, and make $1-\epsilon$ of the knot $k$ straight arcs, so that all of the knotting occurs in an $\epsilon$-long cylinder. By piecewise affine deformations, one can make the length approach $k$ as $\epsilon \to 0$.

Conversely, suppose the length of the knot is $< k$. Then there is a meridian disk meeting the knot in $<k$ points, since the integral of the intersection number with the family of meridian disks $\{t\}\times [0,1]$ gives a lower bound on the length.