7
$\begingroup$

I'm trying to learn more about hyperbolic 3-manifolds, in particular the geometric implications of doing hyperbolic Dehn surgery to suitable knot complements.

Following this paper by Christian Millichap ( https://arxiv.org/abs/1209.1042 ), we can construct a hyperbolic Montesinos knot $K$ with several tangle regions (see p. 8). We then mutate these tangle regions along a suitable Conway sphere, before doing hyperbolic Dehn surgery to fill up the knot complements. Because of how we constructed the hyperbolic Montesinos knot $K$ (in particular, the relevant pair of $S^0$'s are unlinked on $K$, see What does it mean exactly for a pair of $S^0$'s to be unlinked on a knot $K$?), it can be shown that $K$ and all its mutations yield a hyperbolic 3-manifold with the same volume. Further, by an argument in this paper I don't fully understand (for details, see the proof of Theorem 3 on pg. 11), we can pick appropriate coefficients for the Dehn surgery so that the hyperbolic manifolds resulting from $K$ and its mutations are all non-homeomorphic.

I want to have a more precise (and concrete) understanding of how "different" these hyperbolic 3-manifolds are, beyond the fact that they are non-homeomorphic. In particular, suppose we have a hyperbolic knot $K$ and its mutant $K'$ and we know that they yield non-homeomorphic hyperbolic 3-manifolds (call them $M_{K}$ and $M_{K'}$) with the same finite volume via Dehn surgery.

My question is: since mutations of knots involve "cutting up" a certain region of a knot and rotating it appropriately to get a different knot, is it possible for us to cut out an analogous region of $M_{K}$ and (via some isometry in $H^3$) "rotate" the region to obtain $M_{K'}$?

In particular, I don't understand the geometric aspects of hyperbolic Dehn surgery well enough to know if filling in the knot complement with a solid torus presents an obstruction to this. All I know is that hyperbolic Dehn surgery involves picking a suitable pair of integers $(p,q)$ and choosing a basis $(m,l)$ for the fundamental group of the torus boundary of the knot complement (where m and l corresponds to the meridional and longitudinal side of the torus respectively). We may interpret the knot complement as a hyperbolic 3-manifold with a cusp corresponding to the torus boundary; via hyperbolic Dehn surgery, we can cut off the cusp and glue in a solid torus by mapping the boundary of the meridian disc of the torus to $s=pm+ql$ where $s$ is a slope on the torus boundary of the knot complement. (I am following p. 7 of the Millichap paper here). From a geometric standpoint, all I take from this is that there is a very specific way of gluing the solid torus along the torus boundary of the knot complement, and this might give us problems if we were to "cut and paste" the region in order to get a new hyperbolic 3-manifold. Am I right about this?

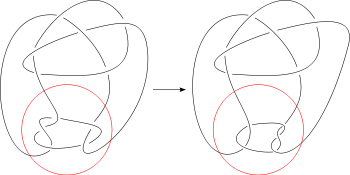
If it helps to have something more visual, consider the following two knots, which are the prime Kinoshita-Teraska knot (LH) and the prime Conway knot (RH). This diagram shows how the two are related by a mutation along the Conway sphere (marked in red) by rotation about the y-axis.

The prime Kinoshita–Terasaka knot (11n42) and the prime Conway knot (11n34) respectively, and how they are related by mutation.

As Ian Agol mentions below, it can be verified that this example of knot mutation satisfies the unlinking condition mentioned above, hence Dehn surgery on the knot complements of these two knots yield hyperbolic 3-manifolds with equal volume. Now, suppose we have filled the knot complements out via Dehn surgery and gotten two non-homeomorphic 3-manifolds with the same volume - can we then cut and rotate whatever is analogous to the red region in the hyperbolic 3-manifold (derived from the LH knot) to get the hyperbolic 3-manifold derived from the RH knot?

Improve this question
$\endgroup$
1
  • $\begingroup$ The name is Montesinos. $\endgroup$
    – Igor Rivin
    Commented Jan 4, 2018 at 17:46

1 Answer 1

Reset to default
8
$\begingroup$

Look at the proof of Theorem 5.5 in Ruberman's paper. The way that he proves that the Dehn fillings on mutants have the same volume is to show that there is a closed genus $\leq 2$ surface on which one may perform mutation, which preserves volume. This surface is obtained from the 4-punctured sphere by tubing along annuli which are tubular neighborhoods of the arcs of the knot lying to one side and whose endpoints are flipped by the mutation. The mutation on the 4-punctured sphere is compatible with mutation on the tubed genus 2 surface, in the sense that mutation along the 4-punctured sphere gives the same manifold as mutation along the genus 2 surface. Hence after Dehn filling, there will still be a mutation along a genus 2 surface which relates the two Dehn fillings.

The examples in your picture do satisfy the unlinking condition (Definition 5.4) which is needed in the hypothesis of Theorem 5.5. Here's a picture of the tubed genus 2 surface:

enter image description here

Improve this answer
$\endgroup$
11
  • $\begingroup$ Thanks for replying - I really appreciate it! I'm aware of Ruberman's paper and of the Theorem, but I'm not sure how the proof relates to the last sentence of your first paragraph. Could you say a little more? I think I understand what you mean by mutation on the 4-punctured sphere being compatible with mutation on a tubed genus 2 surface, but I don't see how this implies that mutation "commutes" with Dehn filling, i.e. mutating the knot $K$ and performing Dehn surgery gives us the same manifold $M$ as performing Dehn surgery on $K$ and "mutating" (?) the hyperbolic 3-manifold. $\endgroup$
    – asldjk
    Commented Jan 5, 2018 at 9:46
  • $\begingroup$ In order for these operations to "commute", mutation has to be well-defined on the 3-manifold and I'm not sure what operation you are referring to here. Also, I'm not sure why exchanging mutation and Dehn filling would yield the same manifold. In particular, note that mutating a knot just involves changing a particular region of the (2-dimensional) knot, whereas mutation of the hyperbolic 3-manifold would affect not only the torus boundary of the knot complement (which is defined by the knot) but also the solid torus that has been filled inside this knot complement via Dehn surgery $\endgroup$
    – asldjk
    Commented Jan 5, 2018 at 9:47
  • $\begingroup$ My concern is that while I can see how by naive rotation, one may move from one 3-manifold to another so that the torus boundary defined by the knot "lines up", I'm not sure if the solid torus filled inside via Dehn surgery will "line up", especially if Dehn surgery depends on the orientation of the knot. $\endgroup$
    – asldjk
    Commented Jan 5, 2018 at 9:48
  • 1
    $\begingroup$ Sorry, I think I see the issue - the automorphism of the closed surface is not mutation (ie not the central involution). Nevertheless, it should preserve the geometric structure. I'll modify my answer later. $\endgroup$
    – Ian Agol
    Commented Jan 5, 2018 at 16:41
  • 1
    $\begingroup$ @asldjk: I don't quite understand your comments. Maybe think about it like this: we have two knots that differ by a mutation on a closed genus 2 surface. Then Dehn fillings will give manifolds that differ by mutation on a genus 2 closed surface. I formulated this originally as something like filling disjoint manifolds is a commutative operation, but I guess you didn't understand that. $\endgroup$
    – Ian Agol
    Commented Jan 5, 2018 at 21:59

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .