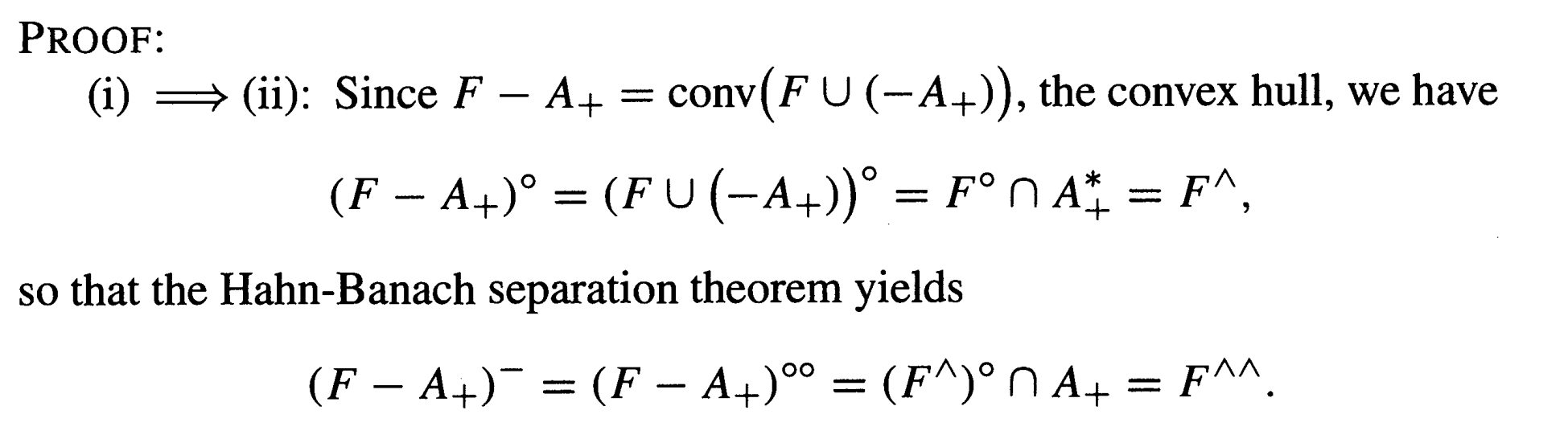$\newcommand{\con}{\operatorname{conv}}$[Edit: This has turned out to be more involved than I thought, and my argument is now somewhat different from the book.] Firstly, some facts about $A_+$. Let $a,b\in A_+$ so $a,b\geq0$ and so $a\geq 0 \implies a+b\geq b \implies a+b\geq 0$. Also $t\in\mathbb R$ with $t\geq 0$ implies that $tA_+ \subseteq A_+$. It follows that $A_+$ is convex, and so also $-A_+$ is convex.
We are proving (i)$\implies$(ii) so by assumption $F$ is a hereditary convex closed set. Set $F_0 = \{ tf : 0\leq t<1, f\in F \}$. As $F$ is hereditary, if $F$ is non-empty there is $f\in F$ so $0\leq0\leq f\implies 0\in F$. Thus $F_0\subseteq F$, and notice that given any $f\in F_0$ there is $0<t_0<1$ and $f_0\in F_0$ with $f = t_0f_0$. $F_0$ is convex.
By definition, $F-A_+ = \{ f-a : f\in F, a\in A_+\}$. As $F_0$ and $A_+$ are convex,
\begin{align*}
\con(F_0\cup(-A_+)) &= \{ tf + s(-a) : f\in F_0,a\in A_+, s,t\geq0, s+t=1\} \\
&= \{ tf - a : f\in F_0, a\in A_+, 0\leq t<1 \} \cup F_0,
\end{align*}
as $A_+$ is invariant under scaling by a positive number. We union with $F_0$ to correspond to the case $t=1$, but as $0\in A_+$, we can write $F_0\ni f = f + 0$. Given $f-a\in F_0-A_+$, pick $t_0,f_0$ with $f = t_0 f_0$ so $f-a = t_0f_0 + (1-t_0)(-(1-t_0)^{-1}a)$. Hence
$$ \con(F_0\cup(-A_+)) = F_0 - A_+. $$
For any subsets $X,Y$ we have $(X\cup Y)^\circ = X^\circ \cap Y^\circ$, just from the definition. So
$$ (F_0 - A_+)^\circ = (F_0\cup(-A_+))^\circ = F_0^\circ \cap (-A_+)^\circ. $$
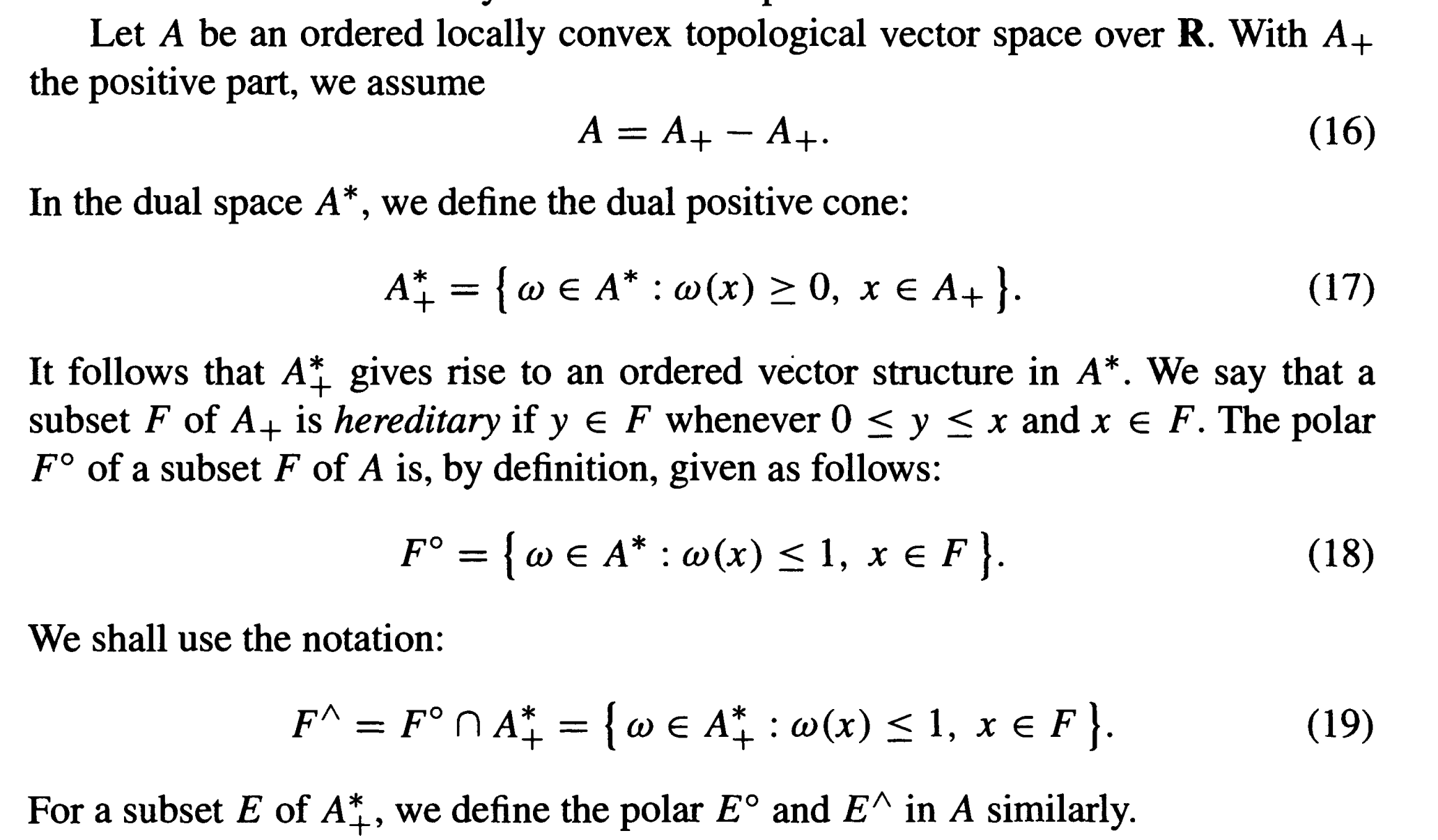
We first show that $(-A_+)^\circ = A_+^*$. If $\omega\in (-A_+)^\circ$ then $\omega(-ta) \leq 1$ for all $t\geq0,a\in A_+$ so $\omega(a)\geq -1/t$ for all $t>0$ so $\omega(a)\geq 0$. Conversely, if $\omega(A_+)\subseteq[0,\infty)$ then $\omega(-a)\leq 0\leq 1$ for all $a\in A_+$. Now consider
\begin{align*}
F_0^\circ &= \{ \omega : t\omega(f)\leq 1 \ (0\leq t<1, f\in F) \} \\
&= \{ \omega : \omega(f)\leq 1 \ (f\in F) \} = F^\circ.
\end{align*}
So $(F_0 - A_+)^\circ = F^\circ \cap A_+^* = F^\wedge$.
We now continue; we finally get to use condition (i)! Hahn-Banach shows that $X^{\circ\circ}$ is the closed convex hull of $X$, for any $X$ which contains $0$. So
$$ (F_0 - A_+)^{\bar{}} = (F_0 - A_+)^{\circ\circ} = (F^\wedge)^\circ. $$
As $F_0 \subseteq F$ and is dense, we have $(F - A_+)^{\bar{}} \subseteq (F_0 - A_+)^{\bar{}} \subseteq (F - A_+)^{\bar{}}$ and so we have equality. Thus $(F - A_+)^{\bar{}} = (F^\wedge)^\circ$. Now use that (i) holds, so $F = (F-A_+)^{\bar{}} \cap A_+$ so $F = (F^\wedge)^\circ \cap A_+ = F^{\wedge\wedge}$, that is, (ii).