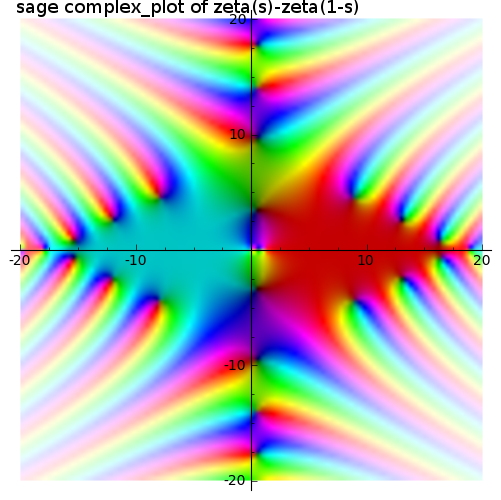
We now have strong results from GH for $\Gamma(s) \pm \Gamma(1-s)$ and $\zeta(s) \pm \zeta(1-s)$ (when both are nonzero), as well as a more generalized claim outlined in this article http://arxiv.org/abs/0712.1266, that zeros for $f(s) = h(s) - h(2a-s)$, with $h$ being a meromorphic function satisfying appropriate growth conditions, are all inclined to lie on a critical line $a$, with only a finite number of exceptions.
However, despite the encouraging outcomes that all zeros are on the critical line and only a finite number of exceptions residing outside the critical strip, there doesn't seem to be any way to extrapolate these results to the non trivial zeros ($\rho$). No new constraints seem to be imposed on their location and they really seem to originate "from deep down the unaccessible cave" of $\zeta(s)$ itself. They therefore could still reside anywhere on the critical strip.
Just to share some 'futile attempts' I made to make a link. Firstly I had hoped that somehow the results would force the following outcome for all $\Re s$ in the strip:
$\lim_{s\to\rho}\left|\frac{\zeta(s)}{\zeta(1-s)}\right|=1$
and thereby seriously limit the possible values $\Re(\rho)$ could assume. But they don't.
In an attempt to find another relation between $\zeta(s)2$ and $\zeta(1-s)$, I converted both of them to the alternating $\eta(s)$ function and then paired up the individual terms for each $n$ (this is allowed since $\eta(s)$ is valid for $\Re s>0$). This gives:
$\displaystyle \sum _{n=1}^{\infty } \left({\frac { \left( -1 \right) ^{n-1}}{(1-{2}^{1-s}) {n}^{s}}} \pm{\frac { \left( -1 \right) ^{n-1}}{ (1-{2}^{s}) {n}^{1-s}}}\right)$
For each individual $n$, this yields a wave with a fixed frequency and amplitude, that only has zeros when $\Re s=1/2$. These waves nicely sum up to a curve that produces all the 'non & semi' trivial zeros from the OP. However, the $\rho$s obviously do arise from summing up the individual terms as well and I could not find a meaningful way to smartly swap out left and right terms, so that maybe new information about the non trivial zeros would be revealed.
As a last attempt, I also experimented with the PrimeZeta function $P(s)$.
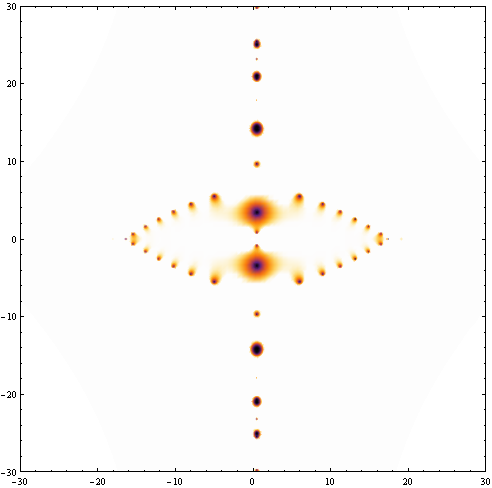
For $P(s)-P(1-s)$ I found:
$\displaystyle \sum _{k=1}^{\infty } \frac{\mu \left( k \right)}{k} \ln \left( {\frac {\zeta \left( ks \right) }{\zeta \left( k \left( 1-s \right) \right) }} \right)$
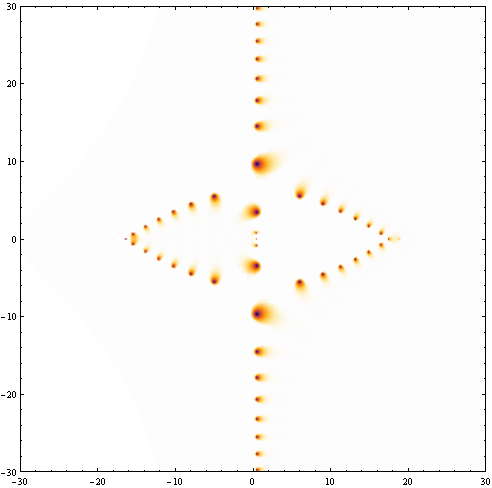
and for $P(s)+P(1-s)$:
$\displaystyle \sum _{k=1}^{\infty } \frac{\mu \left( k \right)}{k} \ln \left( {\zeta \left( ks \right) \zeta \left( k \left( 1-s \right) \right) } \right)$
For both functions, all zeros appear to lie on the critical line $\Re s=\frac12$, but for the first function the non-trivial zeros have now turned into poles. Interesting to note that the Wolfram site on the PrimeZeta states: "According to Fröberg (1968), very little is known about the roots", so maybe there is something new here to proof for $P(s) \pm P(1-s)$... :-)