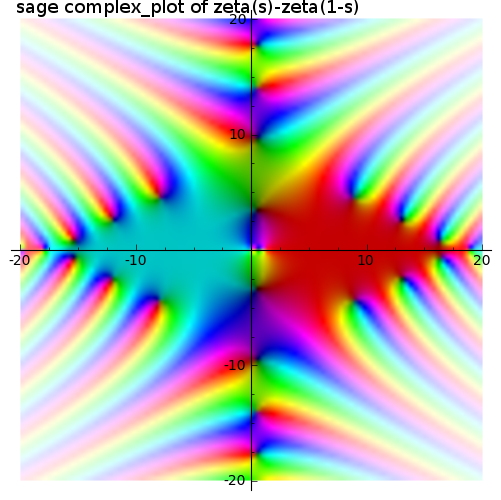
This might be a counter example to $\zeta(s)-\zeta(1-s)$.
Newton's method starting from $13 + 3i$ converges to $s \approx 13.16278786499+2.580464971850i$ which appears a zero.
[Added] A counterexample for $\zeta(s)+\zeta(1-s)$ appears $$ s \approx 14.870309115978233377 + 1.6450192905454179639i $$
[Added later] Per Agno's request the additional zeros found so far for $\zeta(s)-\zeta(1-s)$ are $16.478090665944547285 \pm 0.67940600947784773819i$ and $8.9909145336149198065 \pm 4.5105941406991465448i$
[Added later 2] The additional zeros of $\zeta(s)+\zeta(1-s)$ are $6.0002215061605926659 \pm 5.5128690434285266557$ and $11.248198934515946877 \pm 3.5349340823965337997$.
In all cases if $s$ is a zero so is $1-s$.
Here is a pari session with big precision:
\p 120
a=13.1627878649910358141631713461028903502674045760701701738298467140072119876746309296270651170803651644473445990732853741 + 2.58046497185066957108186074406714794119615081581034778843315640867385970134939303047779039837771630019890293928089781416*I
zeta(a)-zeta(1-a)
%2 = 3.667106168519458724 E-119 - 5.4882932400387681018240947190706996903 E-119*I
The starting value was opportunistically chosen from this plot. The colors are explained here
http://drememi.ludost.net/zeta1.png