In analogy with the lonely runners conjecture, imagine "globe trotters" each traveling on a longitudinal great circle at different (constant, positive) speeds. Each "trotter" behaves just like a runner in the lonely runners situation. Suppose there are $n$ equally spaced longitudinal circles, and $n$ trotters on each circle. So $n^2$ trotters all over the globe.
Each $n$ trotters at one longitude start at the (green) equator and then advance northward (initially), as crudely animated below. Each trotter remains on its longitudinal circle throughout.
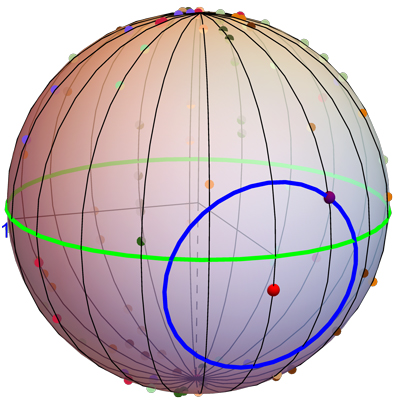
As with the lonely runners conjecture, the question is what radius of a geodesic disk can be guaranteed to be (eventually) empty of all trotters, whether or not on the same longitudinal circle. In the image below, the blue disk centered on the red trotter is the largest empty disk at that time snapshot, with the disk radius the geodesic distance to the purple trotter on the adjacent longitude.
My question is: Is there a positive lower bound on the radius of an empty geodesic disk centered on a trotter, and empty of all other $n^2-1$ trotters, that will occur eventually? Assume the globe/sphere has radius $1$.
Many variations are possible (more or fewer great circles than trotters, constraints on the speeds, trotters switching longitude circles at the north pole, etc.)