Maximum thickness of three linked Euclidean solid tori

Question

Consider three circles of radius $1$ in $\mathbb{R}^3$, linked with each other in the same arrangement as three fibers of the Hopf fibration. Now thicken the circles up into non-overlapping standard round Euclidean solid tori of equal thickness. Allowing the tori to move, there will be some maximum thickness (distance from the core circle to the boundary of the solid torus) before the tori must overlap each other.

I'm interested in the case that the three tori have $3$-fold rotational symmetry, as in the first image here (I think it might be possible to do better with a less symmetrical configuration).

(source)

If realised as a physical object this should have the interesting properly that the only way in which the tori can move is rotation along their axes.

I have a numerical approximation to the best arrangement of the tori, given as follows:

Set up a coordinate system as in the second image, with one circle (of radius $1$) centered on the $x$-axis, at distance $r$ from the origin, and rotated by angles $\theta$ and $\omega$ from the axes. The vectors $U$ and $V$ give the orientation of the circle, and are given by $$U = (\cos(\omega), \sin(\omega), 0), \qquad V=(-\sin(\omega)\sin(\theta), \cos(\omega)\sin(\theta), \cos(\theta)).$$

The other two circles are copies of this one, rotated by $2\pi/3$ and $4\pi/3$ about the $z$-axis. The approximation I have is $r=0.4950, \omega = 0.0000, \theta = -0.8561$, with resulting distance between circles of $0.64576$ (and so torus thickness is $0.32884$). These are accurate to around $4$ decimal places. The arrangement in the picture is this approximation.

Questions:

1. Why does it appear that $\omega=0$? It doesn't seem obvious to me that this should be true. Is there a symmetry argument?
2. Is there a closed form solution?

A closed form solution is probably too much to hope for. In particular, this paper: Finding the distance between two circles in three-dimensional space shows that there is no closed form solution for the distance between arbitrary pairs of circles in $\mathbb{R}^3$. But maybe there is some symmetry argument that helps?

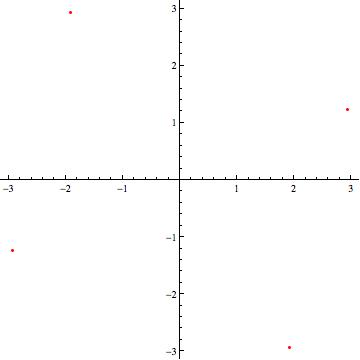
Edit: Better estimates: $r=0.4950197, \theta=-0.8560281$. By Ian Agol's answer, $w=0$. Here is another mysterious symmetry in the numerical solution. Set up a coordinate system on the surface of each torus. Each torus is parameterised by $\alpha, \beta\in[-\pi,\pi)$. The parameter $\alpha$ is in the longitude direction, with 0 at the vector $U$ and $V$ nearest in the positive direction. The parameter $\beta$ is in the meridian direction, with 0 at the biggest longitude (i.e. on the outside of the torus), and the direction $U\times V$ closest in the positive direction. With these coordinates, we can plot the points of contact with the other two tori ($\alpha$ on the x-axis, $\beta$ on the y-axis):

(source)

The (numerically approximated) positions of the points are:

$(-2.941921822296, -1.2298655866392636),$
$(-1.9117269877782, 2.941921878383725),$
$(1.9117269877782, -2.941921878383725),$
$(2.941921822296, 1.2298655866392636)$

Why does the number $2.941921$ appear in both $\alpha$ and $\beta$ coordinates?

Answer 1

I think the minimizer should have dihedral symmetry. I'll give a heuristic explanation for this suggestion.

Consider two linked solid tori of the same shape, such that the outer diameter is less than twice the inner diameter of the hole. If two such tori are tangent at a single point, then one may separate them by a translating each torus in the direction of a vector pointing to one side of the tangent plane at the tangency point.

Now assume that the two linked tori are related by a rotation of order three, rotating one torus to the other. If there is only one tangency point, then one may choose one vector pointing to one side of the tangency plane, which is rotated to the other side of the tangency plane by the rotation. Move each torus by a small translation in the direction of its vector, then by the above observation, they will be separated. A third torus obtained by taking the inverse rotation will also be separated, by symmetry.

Thus, a tight configuration must have two tangencies between each solid torus. What I believe is that two tangent linked isometric tori with two tangency points should be related by an involution rotation exchanging the two (and exchanging the tangency points). If this is true, then in a tight configuration, there should be an extra dihedral symmetry, which would imply $\omega=0$.

Answer 2

You might look at the work of Jason Cantarella, Robert B. Kusner, and John Sullivan, particularly their paper, "On the Minimum Ropelength of Knots and Links" (Inventiones Mathematicae, Vol. 150, 2001). They have methods for constructing thick knots and links, which led to the tight configuration of the Borromean rings adopted by the International Mathematical Union as their logo. Perhaps their optimization methods could be adapted under the additional constraint that the rings must be geometric tori.

(source)

For information on Cantarella's optimization software, see the references in this earlier MO question: "Is it possible to reliably generate a particular approximation of an ideal knot via a simulated annealing approach?"