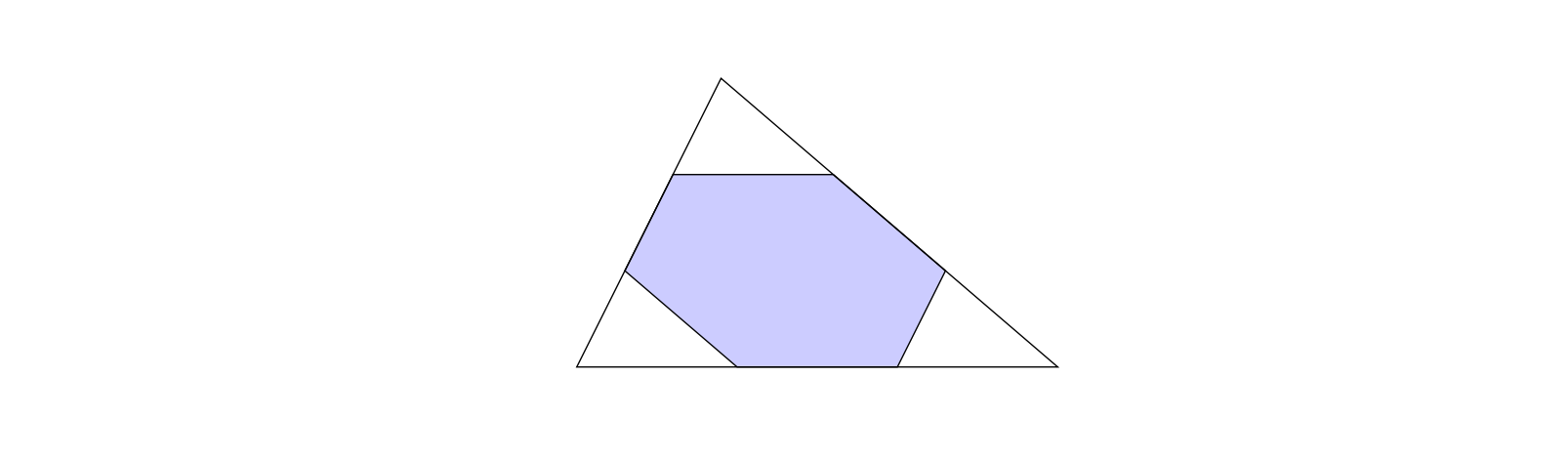
The Kovner-Besicovich theorem states that every convex set $S$ in the plane contains a centrally symmetric subset $C$ of at least $2/3$ the area of $S$, and that this bound is sharp for triangular $S$. In the triangular case, $C$ is given by a hexagon whose vertices are $1/3$ of the way across each side:
I am curious whether we can always use a hexagonal $C$ in this setting, or if there are unit-area $S$ such that the largest centrally symmetric hexagon in $S$ has area less than $2/3$, and if so what the true lower bound is. (It is certainly at least $1/2$, since all convex planar sets contain a parallelogram of at least half their area.)