Finding the smallest centrally symmetric region that contains a convex planar region

Question

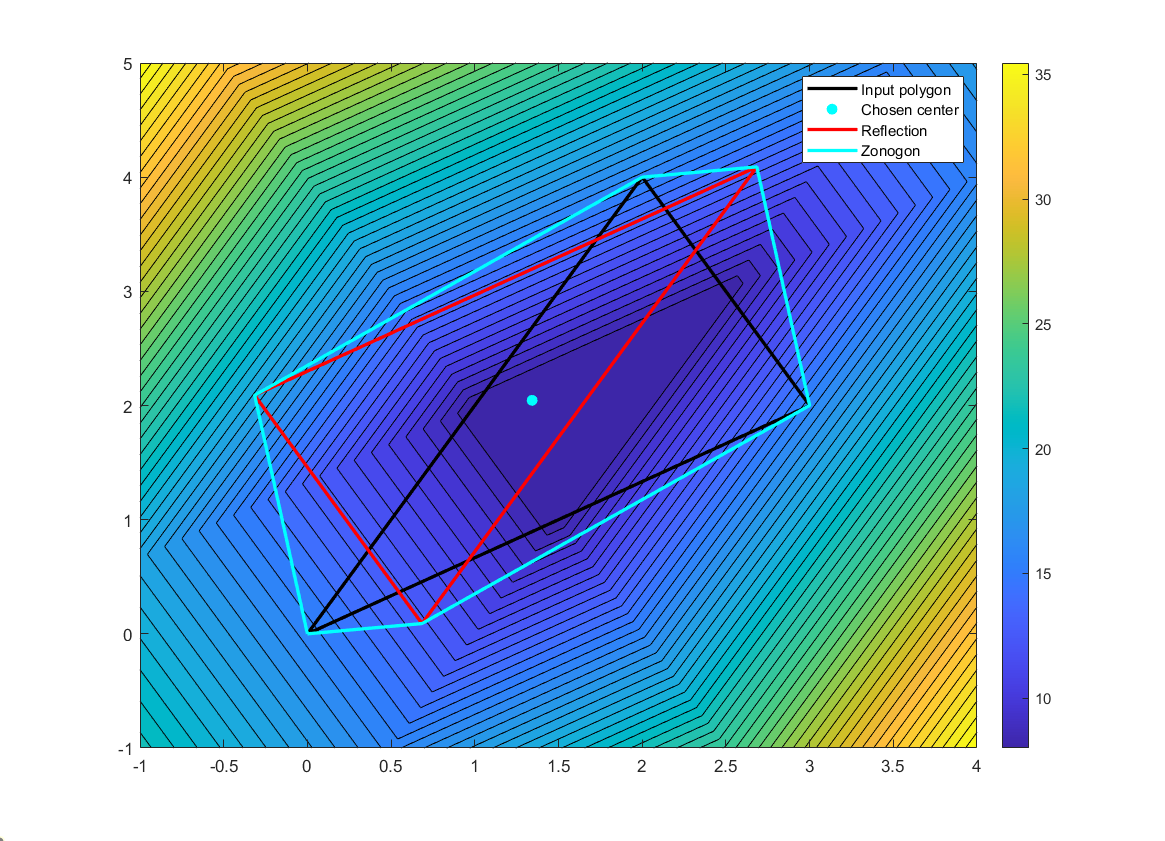
1. Given a convex polygonal region C, how does one find/characterize the smallest zonogon (centrally symmetric convex polygon https://en.wikipedia.org/wiki/Zonogon) that contains C?

Note 1: In question 1, smallest could mean least area or least perimeter. So, one can also ask which C maximizes the difference between its least area and least perimeter convex centrally symmetric container.

2. Given a convex planar region C, how does one find/characterize the largest zonogon contained within C?

Guess: At least the largest area internal zonogon of C seems to have its center on the medial axis of C. Not sure about the least perimeter one - this zonogon could degenerate into a zero area one in some cases.

Note 2: Analogous questions can be asked about axisymmetric containers - and containees - of C.

Note 3: Some similar questions are recorded at Curves of constant width that contain triangles, Smallest triangles that contain 2D convex regions with reflection symmetry and linked pages.

Answer 1

Question 1, in the minimum-area setting, has been studied by Guibas, Nguyen and Zhang (SODA 2003). They prove the following algorithmic result:

Theorem 4.5 The minimum area zonotope containing a set of $n$ points in $\mathbb{R}^2$ can be computed in $O(n \log^2 n)$ [time].

If you set the center of the zonotope at $O$, then the zonotope must contain the original vertices (of your convex polygon) and their reflections (with respect to $O$), and the minimum-area solution is the convex hull of those two point sets. So you can define a function $f$ that maps $O$ to the minimum area. They prove that this area function is unimodal and piecewise affine, and they design an efficient algorithm that finds its minimum.

Guibas, Leonidas J.; Nguyen, An; Zhang, Li, Zonotopes as bounding volumes, Proceedings of the fourteenth annual ACM-SIAM symposium on discrete algorithms, Baltimore, MD, USA, January 12–14, 2003. New York, NY: Association for Computing Machinery; Philadelphia, PA: Society for Industrial and Applied Mathematics (ISBN 0-89871-538-5/pbk). 803-812 (2003). ZBL1092.68697.

(A freely available version of their paper is in ResearchGate.)

Here is an illustration by myself of a minimum-area zonogon around a triangle. The contours illustrate zonogon area as a function of the centerpoint $O$. Observe that the function is indeed piecewise affine (as proven by Guibas et al.), and that somewhere inside the input triangle, there is a nonzero-size region (dark blue) where the area function attains its minimum, so $O$ can be chosen freely from that area (here: randomly).