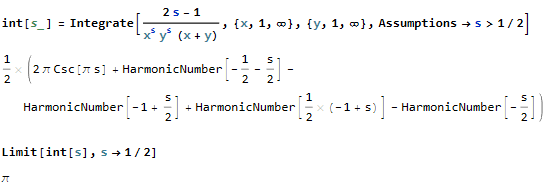While working with multi-zeta functions, I encountered the below (experimental) value for a certain evaluation (in a limit sense). Notice first this well-known fact in context $$\sum_{n,m\geq1}\frac1{nm(n+m)}=2\zeta(3).$$
QUESTION. Does this hold true? If yes, how? $$\lim_{s\rightarrow\frac12^+}\sum_{n,m\geq1}\frac{2s-1}{n^sm^s(n+m)}=\pi.$$
Edited. $s\rightarrow\frac12$ has been replaced by $s\rightarrow\frac12^+$.
The limit (as $s\downarrow1/2$) will not change if the double sum is replaced by the corresponding double integral, for which we find this, as desired:
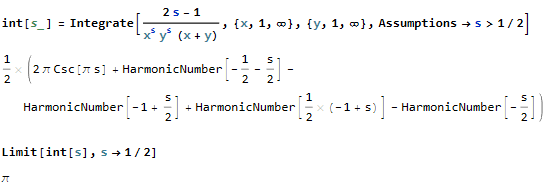
Alternatively, the limit (as $s\downarrow1/2$) will not change if the double sum is replaced by the double integral $$I(s):=(2s-1) \iint\limits_{x,y>0,\,x+y>1}\frac{dx\,dy}{x^s y^s(x+y)} \\ =(2s-1)\int_1^\infty \frac{du}u\,\int_0^u \frac{dx}{x^s (u-x)^s} \\ =(2s-1)\int_1^\infty \frac{du}{u^{2s}}\,J(s) =J(s),$$ where $$J(s):=\int_0^1 \frac{dt}{t^s (1-t)^s}\to J(1/2)=\pi.$$ So, $I(s)\to\pi$, as desired.
Details on why the limit (as $s\downarrow1/2$) will not change if the double sum is replaced by an appropriate double integral: The reasons for this are as follows:
$f(x,y):=\dfrac1{x^s y^s(x+y)}$ is decreasing in $x>0$ for each real $y>0$ and in $y>0$ for each real $x>0$. So, for any natural $i$ and $j$, $f(i,j)\ge\int_{(i,i+1]\times(j,j+1]}f\ge f(i+1,j+1)$.
For each natural $m$, $\sum_{n\ge1}f(m,n)<\infty$ and, similarly, for each natural $n$, $\sum_{m\ge1}f(m,n)<\infty$. Each of such ordinary sums will get multiplied by $2s-1$, and so, will not contribute to the limit as $s\downarrow1/2$.
2a. Similarly to point 2, for each real $x>0$, $\int_{\max(0,1-x)}^\infty f(x,y)\,dy\le\dfrac1{x^s}\int_0^\infty \min\Big(\dfrac1{y^s},\dfrac1{y^{s+1}}\Big)\,dy =\dfrac1{(1-s)sx^s}$, and $\int_{\max(0,1-y)}^\infty f(x,y)\,dx\le\dfrac1{(1-s)sy^s}$ for each real $y>0$.
