4
$\begingroup$

Among all convex pentagons, does the regular pentagon give least packing density?

Further question: For each $n > 6$, is the regular $n$-gon the minimum of packing density?

An analogous question can be asked on covering – for which values of $n= 5$ and above $6$, the regular n-gon gives the maximum covering density among all convex $n$-gons (ie. is the least economical for covering)?

Do the answers to these questions depend on central symmetry – whether $n$ is odd or even?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Concerning covering, see Terrible tilers for covering the plane, and in particular, @WlodekKuperberg's conjecture concerning regular pentagons. $\endgroup$
    – Joseph O'Rourke
    Commented Jul 22, 2021 at 22:51
  • 1
    $\begingroup$ Thanks very much. I understand you raised the special case of the second question above: which is the worst coverer among convex pentagons? And Kuperberg has conjectured that the regular pentagon is the worst coverer among pentagons - and that the best that can be managed with it is a double lattice. I guess it has not yet been proved since then. Further, I learned from your discussion that the disk is NOT the worst coverer from all convex regions and that this worst coverer has not been established. $\endgroup$
    – Nandakumar R
    Commented Jul 23, 2021 at 7:31
  • $\begingroup$ Note that even computing what the packing density of the regular pentagon is appears to be an open problem - see Wikipedia's description of the double-lattice packing as the best known one. So resolving this question in the affirmative is likely extremely difficult. $\endgroup$
    – RavenclawPrefect
    Commented Jul 24, 2021 at 21:16

2 Answers 2

Reset to default
5
$\begingroup$

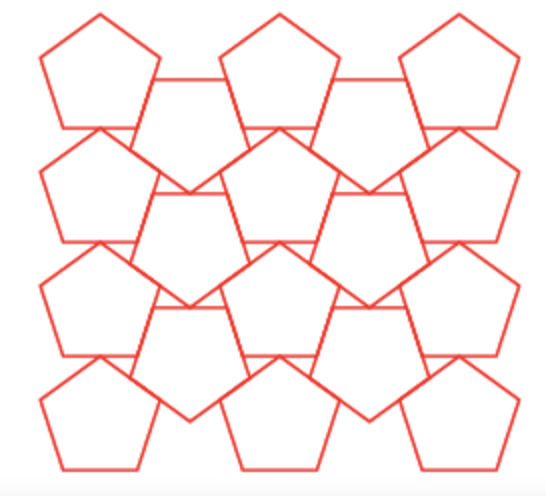
The answer is (very likely) no for all $n>20$.

Let $R_n$ be the regular $n$-gon of circumradius $1$, and given a convex shape $C$, let $\delta(C)$ be its maximal packing density in the plane.

Observe that the packing density of $R_n$ is at least the packing density of the disk times $\text{Area}(R_n)/\pi$ (since we can just inscribe an $R_n$ into each disk).

Thus, we have:

$$\delta(R_n)\ge \frac{\sqrt{3}}6 n\sin(\pi/n)\cos(\pi/n) $$

On the other hand, the regular heptagon $R_7$ is conjectured to have maximal packing density $0.89269$. (The fact that even this result is conjectural should give a sense of how tricky it is likely to be to prove optimality for the regular pentagon - maximal packing densities are hard.)

However, at $n=21$, our bound gives a packing density of at least $0.893$, so at this and all higher $n$ we can do better by taking an $n$-gon collapsed to a regular heptagon.

Probably this result can be improved a bit by finding explicit packings for some $n<20$ which exceed $\delta(R_7)$, if desired (since the circle-inscribing method is never optimal). In fact, I would not be surprised if every $n>7$ could be packed better than $R_7$.

(If you don't want the reliance on a conjectural result, using the smoothed octagon in place of the regular heptagon will give you counterexamples past $n=37$ or so, though the specifics are a bit messier since one has to use a polygonal approximation to the smoothed octagon.)

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Thanks! Even without getting into details, it is clear from what you say that if among all regular n-gons, the worst packing happens some FINITE value of n, say n_0, then for all larger values of n, the worst packer would be an n-gon that is arbitrarily close to a regular n_0-gon and not a regular n-gon. And since the disk is NOT the worst packer, there must be some finite n_0 (probably 7). $\endgroup$
    – Nandakumar R
    Commented Jul 23, 2021 at 11:17
3
$\begingroup$

A few years ago Tom Hales and Wöden Kusner determined the packing density of the regular pentagon, see:

https://www.semanticscholar.org/paper/Packings-of-Regular-Pentagons-in-the-Plane-Hales-Kusner/7b91d1e27c67cc0b99540fa58fbee348dd16e102

Hales-Kusner Fig. 1.

The packing densities of other pentagons, with few exceptions, are unknown. It is known, however, that if a pentagon (convex or not) has a pair of parallel sides, then it tiles the plane with its congruent replicas. This seems to indicate that the regular pentagon, having its pairs of sides farthest from being parallel, is a likely candidate for the "worst packer" among all pentagons.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ (I took the liberty of adding an image from the paper.) $\endgroup$
    – Joseph O'Rourke
    Commented Oct 2, 2022 at 14:08
  • $\begingroup$ Note that a pentagon is not required to have parallel sides to tile 100% of the plane. An equilateral pentagon with a mirror line and angles measuring 90°at the vertices farthest from this mirror line does the job, and it's not that far from a regular one. $\endgroup$
    – Oscar Lanzi
    Commented Nov 6, 2023 at 17:53

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .