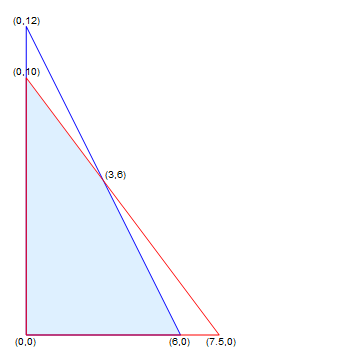
Is there a planar convex region whose enclosing triangles of least perimeter and least area have different areas and different perimeters? And if so, which region maximizes the difference between the two enclosing triangles? Note: The difference can be quantified as the ratio between areas (or perimeters) of the least area and least perimeter triangular containers.
An analogous question can be asked about maximum area and maximum perimeter triangles contained within the convex planar region.
A Claim: For any convex planar region C and specified integer n, the n-gon with least perimeter that contains C and the n-gon with least diameter that contains C are the same. I have no proof or counter to this.
Remarks: In questions 1 and 2, one can replace triangles with quadrilaterals or in general, n-gons and to derive many further questions.
A specific example where a convex polygon has quite different least area and least perimeter containing quadrilaterals is given in Oriented Convex Containers of Polygons (see figure 2; the containing quads are rectangles but there seem to be no smaller general quadrilateral containers). This example does not claim to maximize the difference between the least area and least perimeter quadrilateral containers.
A related earlier question: On convex polygons contained in convex polygons
Note added on 5th November 2021: The same set of questions - difference between min area and min perimeter containers - can also be asked with restricted classes of triangles (right, isosceles, ...) or quadrilaterals (say, kites) as containers of a given convex planar region (Indeed, in the examples given in the answer below the containers are right triangles); and about largest 'contained' triangles in regions etc..
Ref: http://nandacumar.blogspot.com/2021/11/still-more-on-oriented-containers-and.html