Have any proposals been advanced for the analytic continuation of the divisor function?

Question

While I was working on the evaluation of a certain series, the following limit came up:

\begin{align} \lim_{n \to 1} \frac{d(n)-1}{n(n-1)} &= \lim_{n \to 1} \frac{d'(n)}{2n-1} \\ &= d'(1) .\end{align}

Here, I used l'Hôpitals rule, and $d(\cdot)$ denotes the divisor function. In order to compute the derivative of a function, one must know how it is defined on the real numbers. Unfortunately, I have not found any proposals that described the notion of the analytic continuation for the divisor function so far. It seems that it is defined on $\mathbb{Z}$ only.

I did find identities for $\sigma_{\alpha} (x)$ for any $\alpha \in \mathbb{C}$ and $x \in \mathbb{Z}_{\geq 1}$. However, this is not what I am looking for. I seek to find a suitable expression for $\sigma_{\alpha} (x)$ when $\alpha = 0$, and real or complex arguments $x$.

I believe it may be possible to find such an extension of the divisor function, in part because such extensions have been found for the lowercase prime omega function. This arithmetic function is related to the divisor function. The continuation of the function is as follows: $$ \DeclareMathOperator{\sinc}{sinc} \omega(z) = \log_{2} \Bigg{(} \sum_{x=1}^{\lceil Re(z) \rceil} \sinc \Bigg{(} \prod_{y=1}^{\lceil Re(z) \rceil + 1 } (x^{2} + x - yz) \Bigg{)} \Bigg{)} ,$$ where $\sinc(\cdot)$ is the normalized sinc function.

Question: have any proposals been advanced for the analytic continuation of the divisor function, thereby extending the domain to $\mathbb{R}$ or $\mathbb{C}$ ?

Answer 1

I believe the divisor function $d(n)=\sigma_0(n)$ can be analytically continued at least for $n\in\mathbb{R}$, but I'm not sure about $n\in\mathbb{C}$.

---

Consider the divisor summatory function defined in formula (1) below.

$$D(x)=\sum\limits_{n=1}^x\sigma_0(n)\tag{1}$$

---

Now consider the analytic representation of $D_o(x)=\underset{\epsilon\to 0}{\text{lim}}\frac{D(x-\epsilon)+D(x+\epsilon)}{2}$ and it's first order derivative $D_o'(x)$ defined in formulas (2) and (3) below where the evaluation frequency $f$ is assumed to be a positive integer.

$$D_o(x)=\underset{N,f\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N \left(\frac{x}{n}-\left(\frac{1}{2}-\frac{1}{\pi}\sum\limits_{k=1}^{f\,n}\frac{\sin\left(\frac{2 \pi k x}{n}\right)}{k}\right)\right)\right),\quad x>0\tag{2}$$

$$D_o'(x)=\underset{N,f\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N\frac{1}{n}\left(1+2\sum\limits_{k=1}^{f\,n}\cos\left(\frac{2 \pi k x}{n}\right)\right)\right),\quad x>0\tag{3}$$

---

Finally consider the function $f'(x)$ defined in formula (4) below which is a subset of the function $D_o'(x)$ defined in formula (3) above.

$$f'(x)=\underset{N,f\to\infty}{\text{lim}}\left(2\sum\limits_{n=1}^N\frac{1}{n}\sum\limits_{k=1}^{f\,n}\cos\left(\frac{2 \pi k x}{n}\right)\right),\quad x>0\tag{4}$$

---

The function $f'(x)$ defined in formula (4) above evaluates exactly to $2\,f \sigma_0(n)$ when $x=n$ and $n\in\mathbb{Z}\land|n|\le N\land n\ne 0$ which leads to the following analytic formula for $\sigma_0(x)$ where the evaluation frequency $f$ may be chosen to be any positive integer.

$$\sigma_0(x)=\underset{N\to\infty}{\text{lim}}\left(\frac{1}{f}\sum\limits_{n=1}^N\frac{1}{n}\sum\limits_{k=1}^{f\,n}\cos\left(\frac{2 \pi k x}{n}\right)\right)\tag{5}$$

---

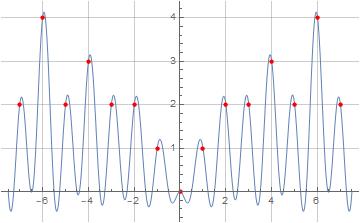
The following two figures illustrate formula (5) for $\sigma_0(x)$ above where Figure (1) is evaluated at $f=1$, Figure (2) is evaluated at $f=2$, and both figures are evaluated at $N=5$. The red discrete portions of the figures illustrate the value of $\sigma_0(x)$ at non-zero integer values of $x$. Note formula (5) for $\sigma_0(x)$ evaluates exactly correct when $x=n$ and $n\in\mathbb{Z}\land|n|\le N\land n\ne 0$. Also note that formula (5) evaluates to $N$ at $x=0$, and therefore the evaluation of formula (5) at $x=0$ diverges to $\infty$ as $N\to\infty$ which is consistent with the fact that zero has an infinite number of divisors. I'll also note that when evaluated at $f=2$, formula (5) for $\sigma_0(x)$ evaluates exactly to zero when evaluated at half-integer values of $x$ which is illustrated in Figure (2) below.

---

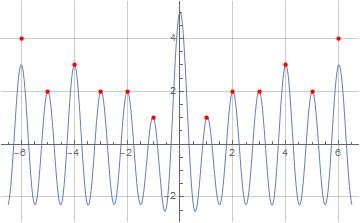
Figure (1): Illustration of formula (5) for $\sigma_0(x)$ evaluated at $N=5$ and $f=1$

---

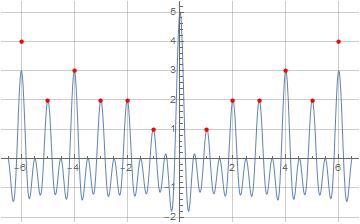
Figure (2): Illustration of formula (5) for $\sigma_0(x)$ evaluated at $N=5$ and $f=2$

---

The evaluation limit $N=5$ was used in Figures (1) and (2) above to illustrate that formula (5) for $\sigma_0(x)$ evaluates exactly correct when $x=n$ and $n\in\mathbb{Z}\land|n|\le N\land n\ne 0$. I usually select a value of $N$ much greater than the largest magnitude of $x$ in the evaluation range which I think is generally desirable. Figure (3) below illustrates formula (5) for $\sigma_0(x)$ evaluated at $f=1$ and $N=100$ in the range $0<x<20.5$.

---

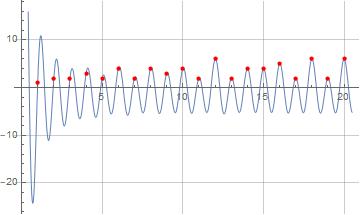
Figure (3): Illustration of formula (5) for $\sigma_0(x)$ evaluated at $f=1$ and $N=100$

---

The derivative $\sigma_0'(x)$ of formula (5) for $\sigma_0(x)$ above is illustrated in formula (6) below.

$$\sigma_0'(x)=\underset{N\to\infty}{\text{lim}}\left(-\frac{2\pi}{f}\sum\limits_{n=1}^N\frac{1}{n^2}\sum\limits_{k=1}^{f\,n} k \sin\left(\frac{2 \pi k x}{n}\right)\right)\tag{6}$$

---

Formula (6) for $\sigma_0'(x)$ above seems to be independent of the value of $f$ when evaluated at $x=1$ (see my related Math StackExchange question), so Figure (4) below just illustrates formula (6) above for $\sigma_0'(1)$ evaluated at $f=1$ as a function of $N$. Note as $N$ increases $\sigma_0'(1)$ also increases in an almost linear manner implying $\underset{N\to\infty}{\text{lim}}\sigma_0'(1)=\infty$.

---

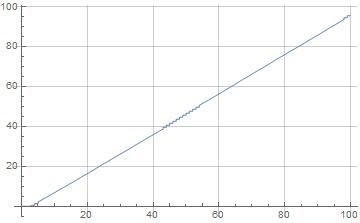
Figure (4): Illustration of formula (6) for $\sigma_0'(1)$ evaluated at $f=1$ as a function of $N$

---

The following table illustrates the trend illustrated in Figure (4) above continues as the magnitude of $N$ increases.

---

$$\begin{array}{cc} N & \sigma_0'(1) \\ 10 & 6.96764 \\ 100 & 96.6867 \\ 1000 & 996.657 \\ 10000 & 9996.65 \\ \end{array}$$

Answer 2

Since the formula I posted in my previous answer only converges as $N\to\infty$ at $x\in\mathbb{Z}_{\ne 0}$, I decided to post another formula which I believe converges as $N\to\infty$ for $x\in\mathbb{C}$. The previous answer I posted is consistent with the fact that zero has an infinite number of divisors, but this answer assumes the definition $\sigma_0(0)=0$.

---

Consider the following definitions of the analytic extension $\tilde{\sigma}_0(x)$ of $\sigma_0(n)$ and it's first order derivative $\tilde{\sigma}_0'(x)$ which are based on partial evaluations of real analytic formulas for $\tilde{f_{\sigma_0}}'(x)=\sum\limits_n \sigma_0(n)\,\delta(x-n)$ and $\tilde{f_{\sigma_0}}''(x)=\sum\limits_n \sigma_0(n)\,\delta'(x-n)$ (see this answer I posted to a related question on Math StackExchange).

---

$$\tilde{\sigma}_0(x)=\underset{N\to\infty}{\text{lim}}\left(\frac{1}{2}\sum\limits_{n=1}^N\left(-\frac{\sin(2 \pi x)}{\pi x}+\frac{1}{n}\sum\limits_{k=1}^n\left(\cos\left(\frac{2 \pi k x}{n}\right)+\cos\left(\frac{2 \pi (k-1) x}{n}\right)\right)\right)\right)\tag{1}$$

$$\tilde{\sigma}_0'(x)=\underset{N\to\infty}{\text{lim}}\left(\frac{1}{2}\sum\limits_{n=1}^N\left(\frac{\sin(2 \pi x)-2 \pi x \cos(2 \pi x)}{\pi x^2}-\frac{2 \pi}{n^2}\sum\limits_{k=1}^n\left(k \sin\left(\frac{2 \pi k x}{n}\right)+(k-1) \sin\left(\frac{2 \pi (k-1) x}{n}\right)\right)\right)\right)\tag{2}$$

---

Figure (1) below illustrates formula (1) for $\tilde{\sigma}_0(x)$ evaluated at $N=100$ in blue where the red discrete evaluation points illustrate $\sigma_0(x)$ for $x=n\in\mathbb{Z}$.

---

Figure (1): Illustration of formula (1) for $\tilde{\sigma}_0(x)$

---

The following table illustrates formula (2) for $\tilde{\sigma}_0'(x)$ evaluated at $x=1$ seems to converge to approximately $-3.35$ as $N\to\infty$.

---

$$\begin{array}{cc} N & \tilde{\sigma}_0'(1) \\ 100 & -3.31334 \\ 1000 & -3.34278 \\ 10000 & -3.34574 \\ \end{array}$$