The Hofstadter–Conway \$10000 sequence is defined by the nested recurrence relation $$c(n) = c(c(n-1)) + c(n-c(n-1))$$ with $c(1) = c(2) = 1$. This sequence is A004001 and it is well-known that this sequence has many amazing properties which are investigated in a very interesting paper Kubo and Vakil - On Conway's recursive sequence.
A related curious sequence can be defined by similar recurrence relation as below $$c^*(n) = n - c^*(c^*(n-1)) - c^*(n-c^*(n-1))$$ with $c^*(1) = c^*(2) = 1$. I introduced this sequence in A287422 and recurrence is also investigated in terms of sensitivity of initial conditions selections Alkan and Aybar - On Families of Solutions for Meta-Fibonacci Recursions Related to Hofstadter–Conway $10000 Sequence.
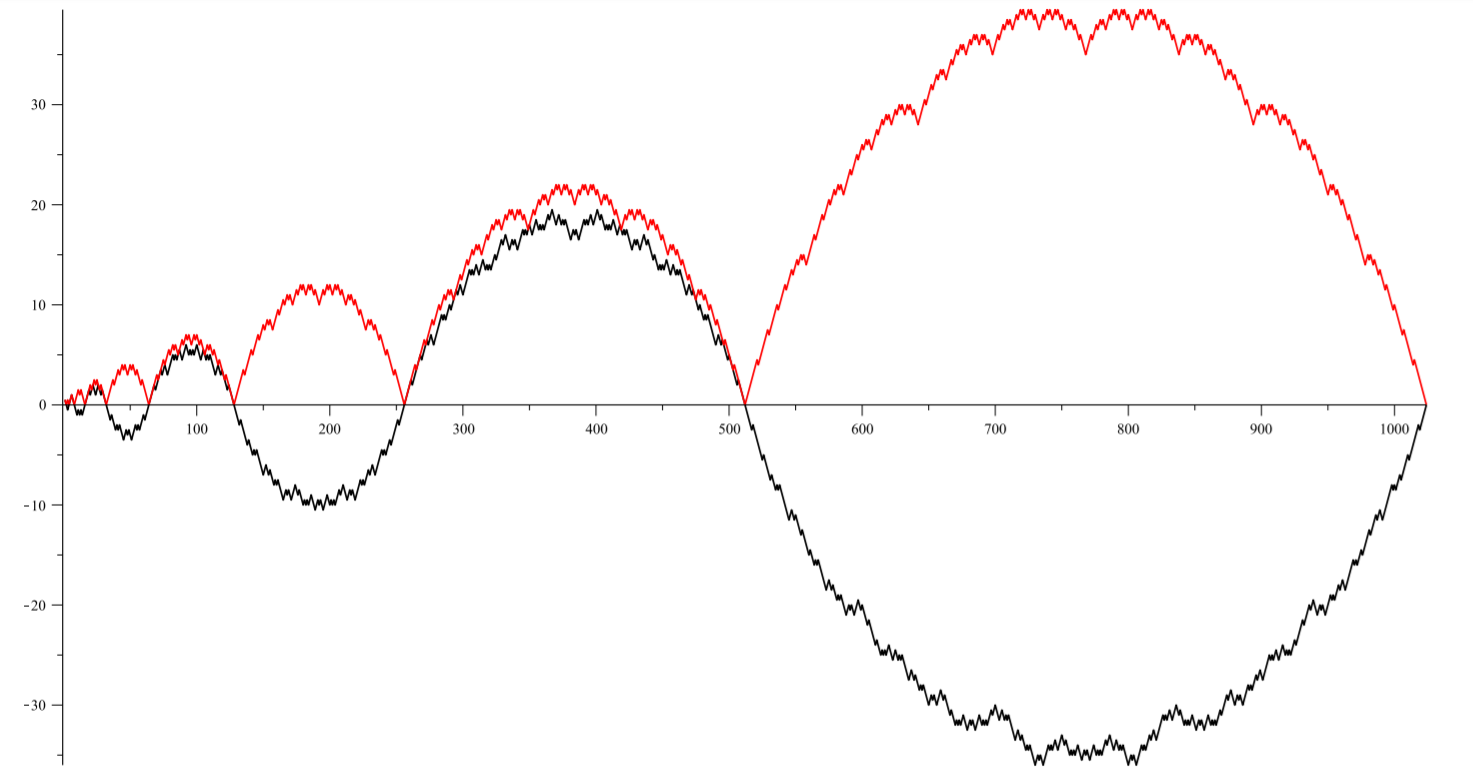
Conjecture. $c(n) - \frac{n}2$ $\ge$ $\left\lvert c^*(n) - \frac{n}2 \right\rvert$ for all $n \ge 1$. (It is checked up to $2^{32}$.)
Question. Can someone show that $c(n) - \frac{n}2$ $\ge$ $\left\lvert c^*(n) - \frac{n}2 \right\rvert$ for all $n \ge 1$ ?
Comments that are related to combinatorial characterization of $c^*(n)$ are also very welcome.
(I the share below graph in order to display behaviours of both sequences for $n \le 2^{10}$. Red: $c(n) - \frac{n}2$, Black: $c^*(n) - \frac{n}2$.)
Note. I believe that it is also possible to see a way to connection of $c(n)$ and $c^*(n)$ thanks to certain auxiliary variants defined by A317754 and A317854, see the below graph (red: transformation of $c(n)$, black: transformation of $c^*(n)$). (These variants also make probably interesting sounds if one can think that recurrences that produce sequences with curious sounds are general of interest.)