23
$\begingroup$

Q. Can every knot be realized as the trajectory of a spaceship weaving among a finite number of fixed planets, subject to gravity alone?

         

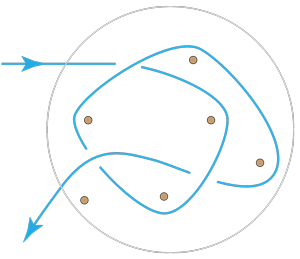
To make this more specific, let $S$ be a large sphere, containing a finite number of planet-points $P=\{p_1, \ldots, p_n\}$. The planet-points have (in general) different masses, and are fixed in $\mathbb{R}^3$. A spaceship $x$ approaches $S$ from $\infty$, interacts via gravity with the point masses, and eventually exits $S$ to $\infty$. Define the knot $K$ realized by the ship's trajectory as the path of $x$ plus a connection between the two ends at $\infty$. (Assume those two $\infty$-ends are distinct.)

Q'. For any given knot $K$, can one arrange point masses in $P \subset S$ and a line and speed of approach to $S$ so that $x$'s path weaves $K$ by interacting with the planets via gravity alone, i.e., without the use of rocket fuel?

One approach might be to design a "gadget" that mimics a vertex $v$ of a stick knot and $v$'s two incident segments. But preventing the vertex gadgets from interfering with one another might not be straightforward.

         
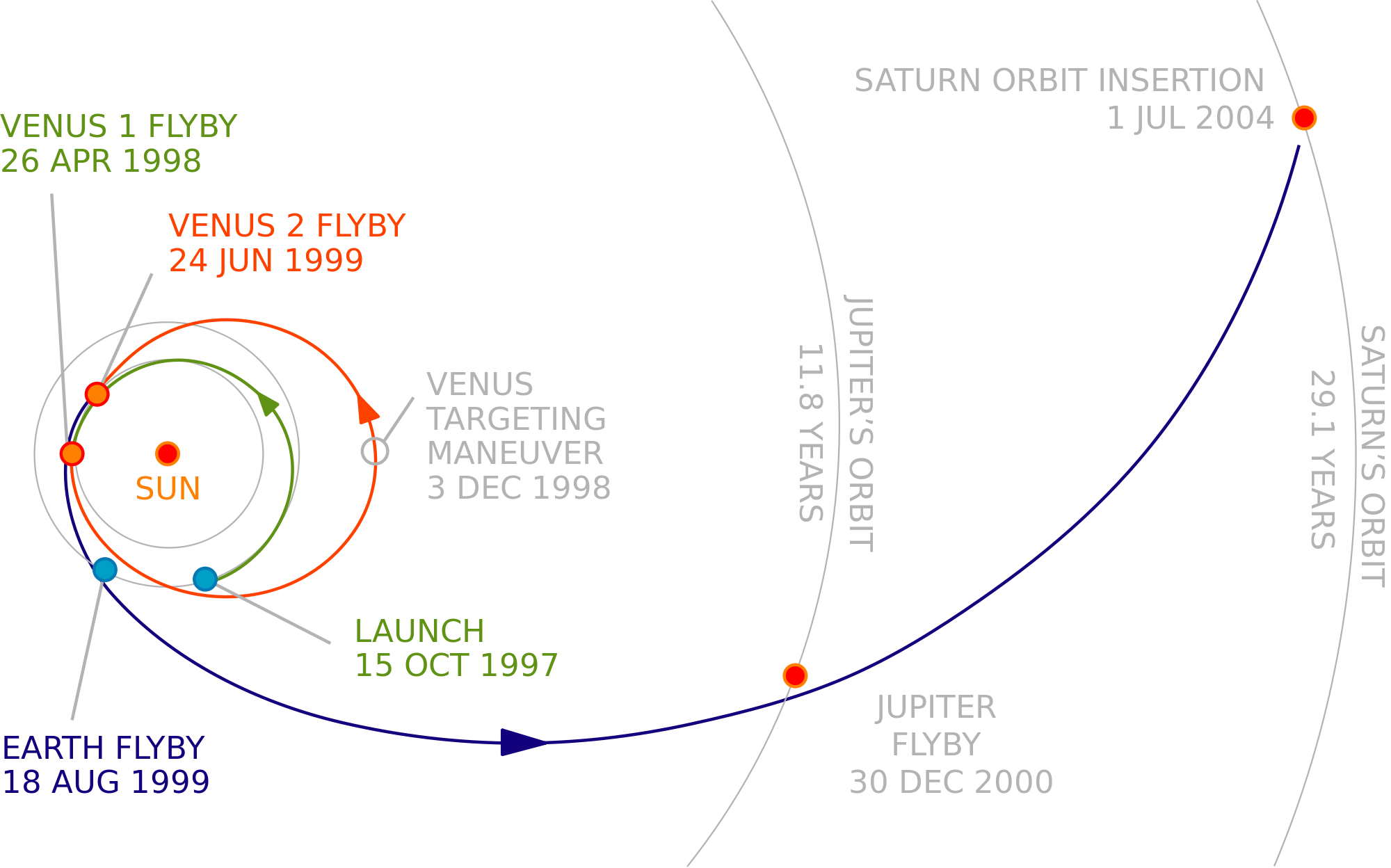
          Cassini gravity-assist trajectory. Image from NASA/JPL.

Improve this question
$\endgroup$
10
  • 4
    $\begingroup$ You can also ask if every knot type occurs as a periodic orbit in the system. $\endgroup$
    – Thomas Rot
    Commented Jun 1, 2018 at 11:33
  • 2
    $\begingroup$ @ThomasRot: Yes. I suspect that is considerably more difficult to achieve. $\endgroup$
    – Joseph O'Rourke
    Commented Jun 1, 2018 at 11:59
  • 8
    $\begingroup$ You can make a hyperbolic orbit around a single planet, which on large scale is just a turn. Now tie the knot with a broken line and place masses close to the turns. Note that you can use an arbitrarily small mass to make any turn in a small external field if you place it strategically near the empty space trajectory (this requires proof, of course, but intuitively it is clear). Thus, adding new turns won't spoil the existing ones and you should be able to happily do the induction. I'll try to make some rigorous sense of it later. $\endgroup$
    – fedja
    Commented Jun 2, 2018 at 0:20
  • 1
    $\begingroup$ I would expect fedja’s method to be extendable to produce exactly periodic orbits, assuming you close off the induction with some sort of fixpoint theorem. $\endgroup$
    – Geoffrey Irving
    Commented Jun 2, 2018 at 23:18
  • 1
    $\begingroup$ @JosephO'Rourke Thank you for the clarification, I meant that my statement about realizing differentiable paths using a Lagrangian needs the paths to be twice differentiable at least for it to be true. I think fedja's intuition is more precise, and correct -- to make the observation slightly more explicit, we can note that the contribution to the spaceship Lagrangian for each planet is of the form $G\frac{m_i}{r_i},$ so as suggested we should be able to place sufficiently small masses sufficiently close to the unperturbed path to reproduce any loop. $\endgroup$
    – Alec Rhea
    Commented Jun 3, 2018 at 6:41

1 Answer 1

Reset to default
8
$\begingroup$

Yes, with some caveats. For an authoritative source, please see the monograph Koon et al. "Dynamical systems, the three-body problem and space mission design"

The short story is that with 2 or more large bodies, trajectory of a spacecraft is "chaotic", and hence under some conditions, it can be shown that horseshoe-type dynamics exist. In other words, if you label the regions around each large body with an alphabet, any arbitrary string of alphabets can be achieved"

Also see: http://www2.esm.vt.edu/~sdross/papers/AmericanScientist2006.pdf

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ Koon, Wang Sang, Martin W. Lo, Jerrold E. Marsden, and Shane D. Ross. "Dynamical systems, the three-body problem and space mission design." (2008). World Scientific link. $\endgroup$
    – Joseph O'Rourke
    Commented Jun 2, 2018 at 1:18
  • 3
    $\begingroup$ The paper you cite deals with PLANAR 3-body problem. In the original problem with knot, what "regions around each body" mean? There is only one region. $\endgroup$
    – Alexandre Eremenko
    Commented Jun 2, 2018 at 13:54
  • 1
    $\begingroup$ It does seems this is a suggestion for a possible existence proof, but far from a constructive proof, which would be most satisfying. $\endgroup$
    – Joseph O'Rourke
    Commented Jun 2, 2018 at 21:59
  • 2
    $\begingroup$ A typical case here is to think of a planet-moon environment, where there is a dominant large body, and N-1 smaller "large" bodies (aka moons). Then one can build arbitrarily ordered "tours" of the moons, given certain conditions are met, i.e. it is possible to find a trajectory that goes around moon1 five times, then moon3 two times, and moon2 three times. See here: cds.caltech.edu/~koon/presentations/barcelona_june.pdf $\endgroup$
    – Piyush Grover
    Commented Jun 3, 2018 at 20:24
  • 1
    $\begingroup$ This is a nice problem Joseph. I do not think the Koon-Marsden work will help. The system you speak of is called the ``N-center problem''. The fact that you do not want to collide with the centers themselves adds topology in that is not there without them. For the planar version with periodic solutions related to braid types, see: arxiv:1201.0280. $\endgroup$
    – Richard Montgomery
    Commented Jun 5, 2018 at 23:30

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .