Rolling wheel unicycle knots

Question

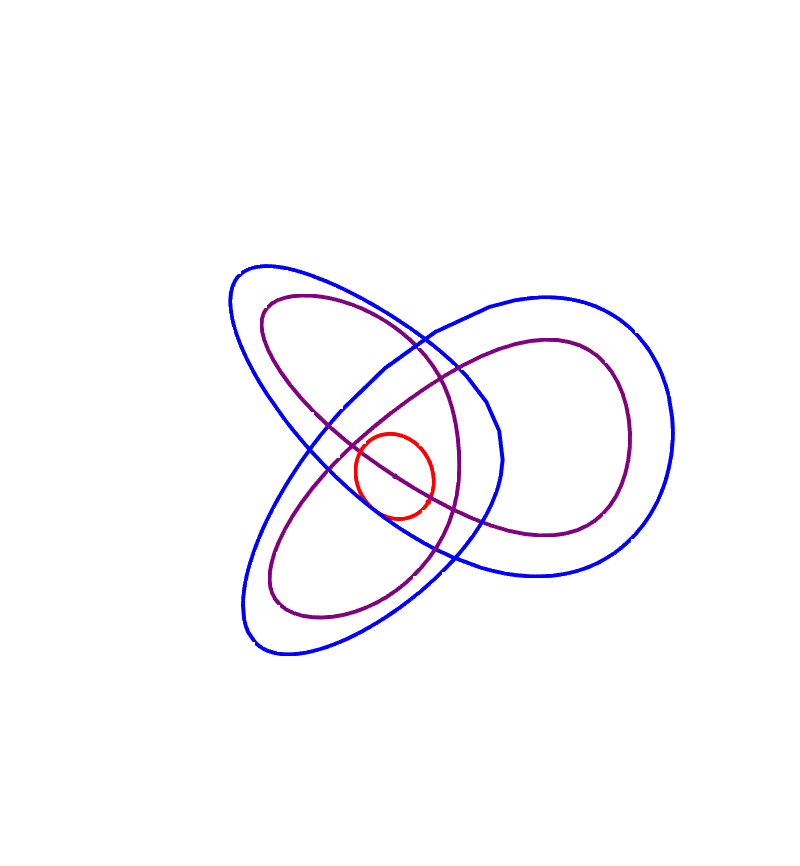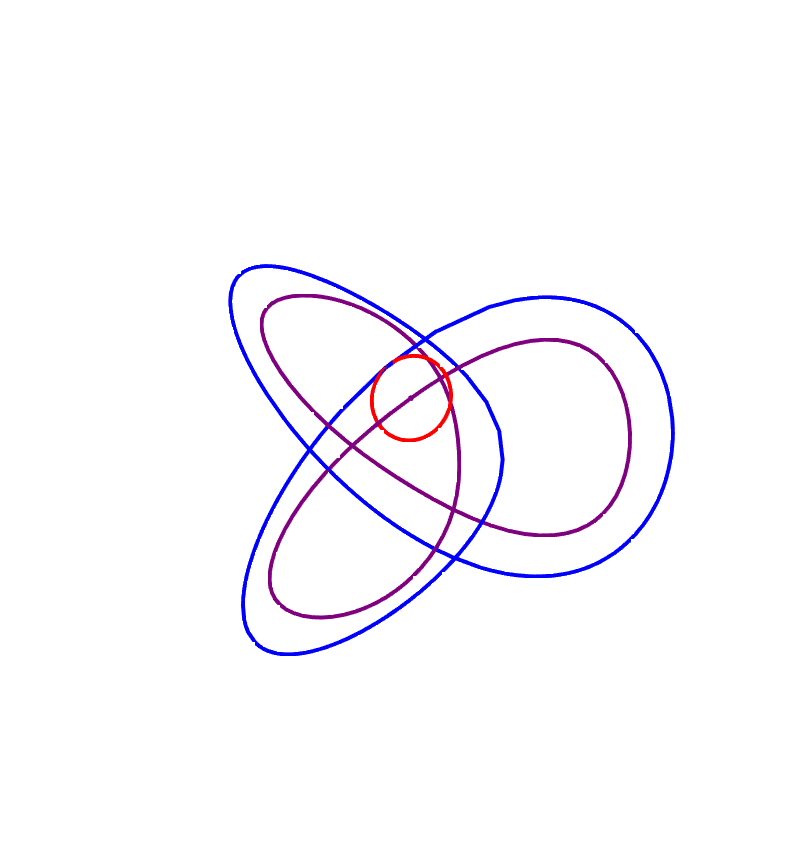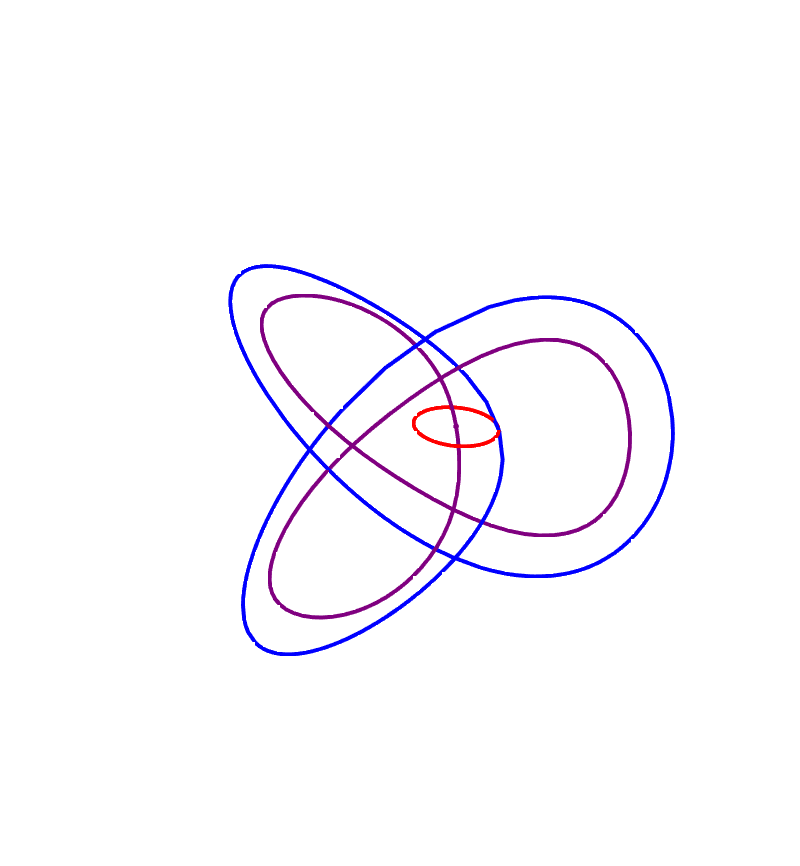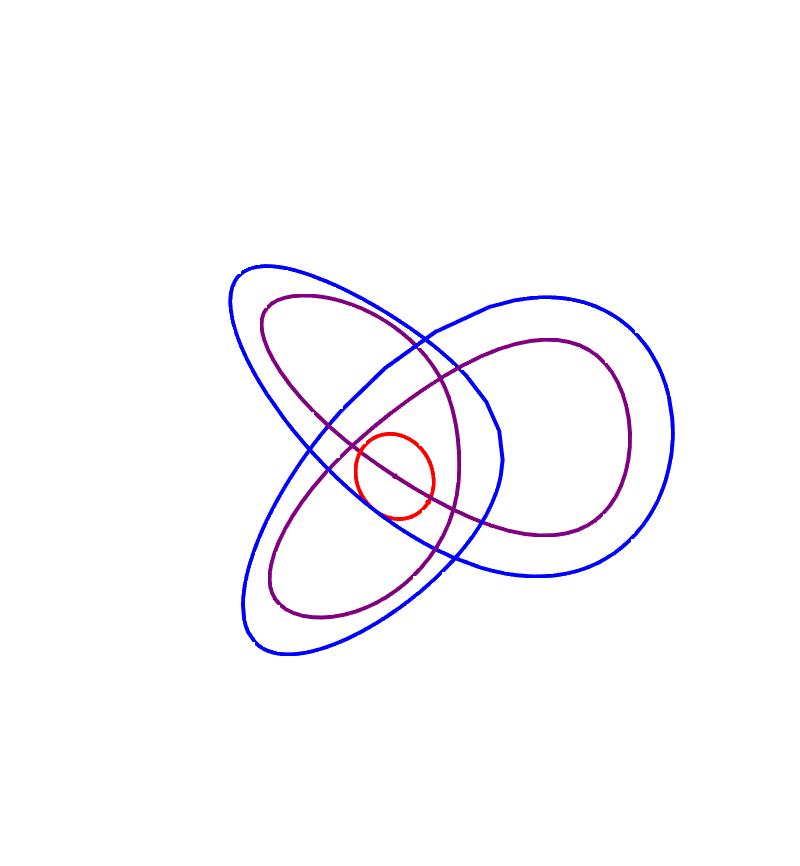
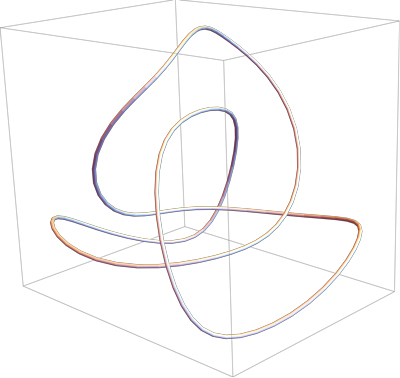
Let $K$ be a knot, and $K(t)$ a parametrization of a space curve that realizes $K$. Roll a wheel $W$ of radius $r$ on $K(t)$ so that $W$ remains in the tangent-normal plane. Now track the wheel's center $c(t)$. Call $c(t)$ a unicycle knot. See the crude animation below, where $K$ (blue) is a trefoil knot, as is $c(t)$ (purple).

---

(Animation code based on that of ubpdqn.)

Increasing the radius of $W$ by a factor of $10$ creates this $c(t)$, which is still a trefoil:

     

Q. For a knot $K$ realized as a space curve $K(t)$, is every unicycle knot $c(t)$ for all radii topologically the same knot as $K$? If not, what is the range of possibly different unicycle knots for a given $K(t)$? In particular, does every $K(t)$ have a unicycle unknot?

Answer 1

First of all, we should assume that the original knot $K(t)$ is twice differentiable and has non vanishing curvature, so that its principal normal $N(t)$ exists and the curve $c(t)$ you want is well defined. Then we will have $c(t)=K(t)+r N(t)$.

By the tubular neighborhood theorem, for small $r$ the perturbation $c(t)$ will be isotopic to $K(t)$; however, this will not be the case in general as $r$ increases. One can certainly construct specific examples where $c(t)$ develops self intersections for certain values of $r$, and beyond those radii the knot type will change.

By arranging pairs of circular arcs in $K(t)$ which curve towards each other, one can construct all kinds of examples where a knot becomes unknotted, or an unknot becomes knotted after a small perturbation. I doubt if there are any general theorems one can prove here without further restrictions or conditions.