I am seeking literature on 3D orthogonal drawings of knots, especially minimum bend drawings. An orthogonal drawing employs segments parallel to the axes of a Cartesian coordinate system. A bend is a vertex at which two segments meet orthogonally. A drawing insists on simplicity in the sense that nonadjacent segments are disjoint, and adjacent segments meet only at their shared endpoint.
One can imagine first drawing a 2D projection with a minimal
number of crossings and then removing the crossings.
For the trefoil below, naive crossing-removal
increments the $8$ bends in the 2D drawing to $8 + 3 \cdot 4 = 20$
bends, but the trefoil can be drawn with $12$ bends:
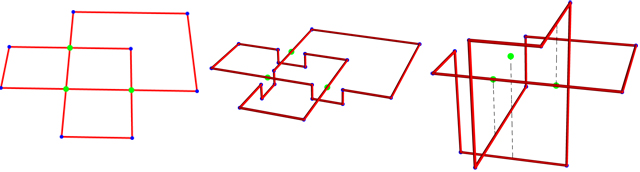
I would be especially interested in algorithmic methods to derive
the right 3D drawing above from the left 2D projection.
Thanks for ideas and pointers!
