1
$\begingroup$

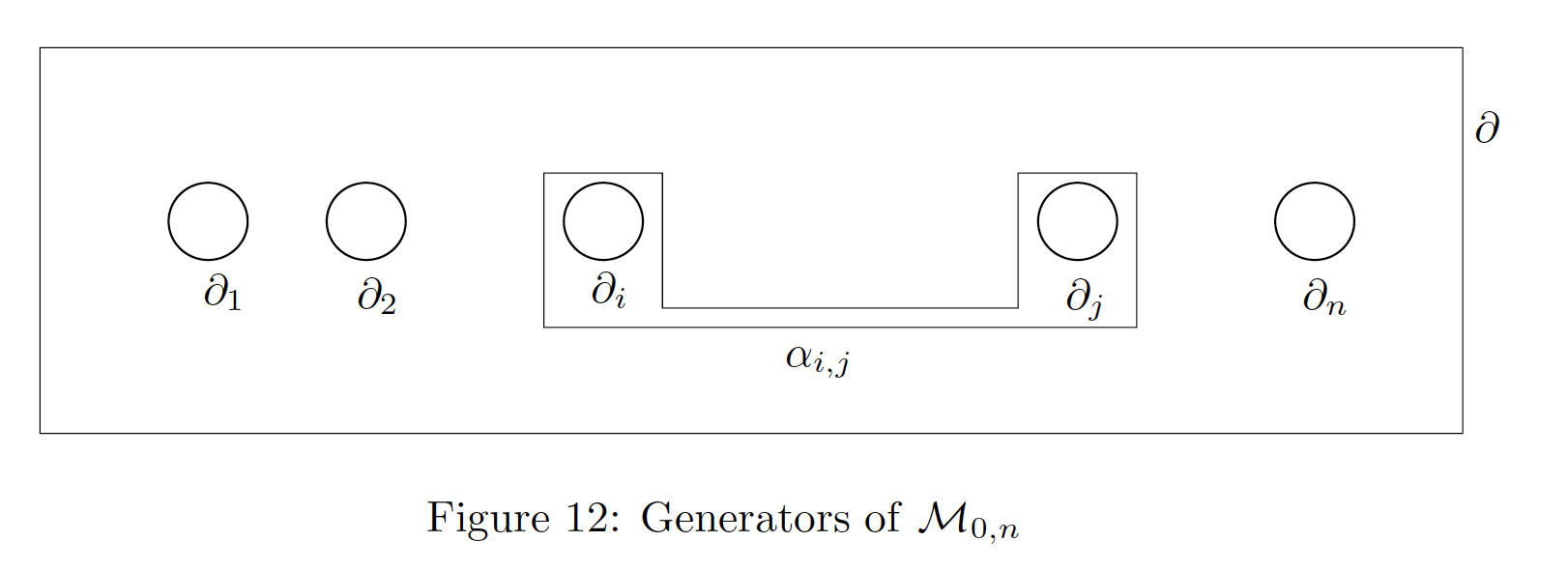
Can you help me find a reference or explain how to find explicit Dehn twist generators for $MCG(S_{0,n})$, the mapping class group of a genus $0$ surface with $n$ boundary components, fixing the boundary components pointwise? (for the problem I am working on, $n=4,5$ would be sufficient.)

PS: My original post was about the mapping class group of a $n$-punctured sphere, but then I realized what I am looking for is Dehn twist generators for the mapping class group of a sphere with $n$ boundary components, so I edited the problem accordingly.

Improve this question
$\endgroup$
8
  • 2
    $\begingroup$ It is not generated by Dehn twists. The reason is that Dehn twist act trivially on the puncures, so the induced permutation is the identity. In other words, they are pure braids. On the other hand, a half twist induces a transposition. $\endgroup$
    – Daniele Zuddas
    Commented Jan 12, 2018 at 23:11
  • 1
    $\begingroup$ All you want to know is contained here: math.stackexchange.com/questions/616981/… $\endgroup$
    – Igor Rivin
    Commented Jan 12, 2018 at 23:14
  • $\begingroup$ @DanieleZuddas So does that mean that Dehn' theorem, that the mapping class group of a surface is generated by Dehn twists, is only valid for closed surfaces? $\endgroup$
    – braid rep
    Commented Jan 13, 2018 at 0:09
  • $\begingroup$ I suspect I did not specify what I meant by a punctured disc. I am trying to find Dehn twist generators for a disc with $n$ holes, i.e. a disc with $n$ inner boundary components, or equivalenty a $2$-sphere with $n+1$ boundary components. $\endgroup$
    – braid rep
    Commented Jan 13, 2018 at 0:18
  • $\begingroup$ To be precise... by punctures we mean points you remove, and they can be permuted by a mapping class. On the other hand, for a compact genus-0 surface with n boundary components, the mapping class group acts trivially on the boundary, so half twists do not occur, and this mapping class group is not the braid group. $\endgroup$
    – Daniele Zuddas
    Commented Jan 13, 2018 at 1:45

1 Answer 1

Reset to default
2
$\begingroup$

The answer is in Wajnryb's paper from which I attach Figure 12. enter image description here

Improve this answer
$\endgroup$
0

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .