3
$\begingroup$

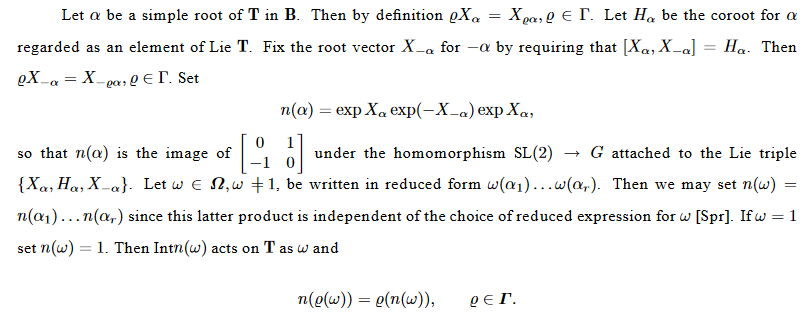
Let $G$ be a connected, reductive group which is quasisplit over a field $k$ of characteristic zero. Let $B$ be a Borel subgroup defined over $k$, containing a maximal torus $T$ defined over $k$. Let $\Delta$ be the base of $\Phi(G,T)$ corresponding to $B$, and let $\Gamma = \textrm{Gal}(\overline{k}/k)$. A $k$-splitting is a choice of isomorphisms of algebraic groups $X_{\alpha}: \mathbf G_{a,\overline{k}} \rightarrow U_{\alpha} : \alpha \in \Delta$, where $U_{\alpha}$ is the root subgroup of $U_{\overline{k}}$ corresponding to $\alpha$. We say that $\Gamma$ preserves the splitting if $\gamma.X_{\alpha} = X_{\gamma.\alpha}$. There exists a $k$-splitting preserved by $\Gamma$.

In the paper On the Definition of Transfer Factors by Langlands and Shelstad, a method to choose canonical Weyl group representatives is given:

enter image description here

One first chooses representatives for the simple reflections $\varpi(\alpha) : \alpha \in \Delta$, and then representatives for the other reflections by means of a reduced decomposition.

I am unfamiliar with the way the authors are thinking of these things, and had a few questions. I would appreciate any explanation or references.

1 . If $H_{\alpha}: \mathbf G_{m,\overline{k}} \rightarrow T$ is the coroot attached to $\alpha$, how can $H_{\alpha}$ be regarded as an element of the Lie algebra $\mathfrak t$ of $T$?

2 . What is meant by $\textrm{exp } X_{\alpha}$? Does this have something to do with the tangent space map associated to $X_{\alpha}$?

3 . What is meant precisely by "the homomorphism $\textrm{SL}(2) \rightarrow G$ attached to the Lie triple $\{X_{\alpha},H_{\alpha}, X_{-\alpha}\}$? I assume this has something to do with a choice of an isogeny $\textrm{SL}_2 \rightarrow [Z_G((\textrm{Ker } \alpha)^0),Z_G((\textrm{Ker } \alpha)^0)]$.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ (1) $H_\alpha$ as an element of $\mathfrak t$ is $\mathrm dH_\alpha(1)$ in your sense. (2) Yes, precisely; it uses an identification of the root subspace with the root subgroup. This is canonical only up to conjugation by $T$. (3) This is the homomorphism coming from the theory of Jacobson–Morosov triples. It too is canonical only up to conjugation by something or other. $\endgroup$
    – LSpice
    Commented Jun 17, 2017 at 3:12
  • $\begingroup$ (2) $X_\alpha$ is a nilpotent matrix, so in char. 0 $\exp X_\alpha$ is defined. (3) You have $H_\alpha$, then you choose $X_\alpha$ and get $X_{-\alpha}$ such that $[X_\alpha,X_{-\alpha}]=H_\alpha$. This triple $(X_\alpha, H_\alpha,X_{-\alpha})$ defines an embedding ${\rm Lie\,SL}(2)\to {\rm Lie}\,G$, which is the differential of some homomorphism ${\rm SL}(2) \to G$. This homomorphism is what you need. $\endgroup$
    – Mikhail Borovoi
    Commented Jun 18, 2017 at 13:01

1 Answer 1

Reset to default
1
$\begingroup$

The three questions asked are fairly elementary, as the comment by LSpice indicates; in the format here, it's best to avoid multiple questions however. Aside from this, it's probably more natural to think of choosing canonical representatives for a Weyl group in purely algebraic terms. For this a good reference would be the lecture notes written up at Yale in 1967-68 as Robert Steinberg gave his course there. Though formerly quite readily accessible online (for instance in the original typewritten format), it's harder to locate a PDF version now that there is a somewhat edited AMS softcover publication using LaTeX: Lectures on Chevalley Groups, University Lecture Series, Vol. 66 (2016).

Here the exponentials are formal when the field has prime characteristic, but the ideas are the same as in characteristic 0. For the split groups, see especially Chapter 3. For non-split but quasi-split groups see Chapter 11; but here the "Weyl group" is a subgroup of the usual one, so extra care must be taken to include this situation. In any case, the canonical representatives can be chosen essentially as indicated: fix an arbitrary reduced expression and take a product of expressions for the various simple roots involved. (In the quasi-split case, the procedure is similar.)

By the way, it's more precise to use tags here such as 'weyl-groups', 'reference-request', 'algebraic-groups', along with 'lie-algebras'.

ADDED: Concerning the term "canonical" used here, it's potentially rather delicate when choosing representatives of Weyl group elements. Typically the choices (say in a split group) differ by an element of a maximal torus (Cartan subgroup). Indeed, the Weyl group itself may or many not embed naturally into the given group. For most practical purposes it's often enough to fix various data involved and then work with the resulting coset representatives.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .