Suppose that $f(x):=\sum_{k\geq 0}(-1)^ke^{-(2k+1)^2x}$ has a holomorphic continuation to a neighborhood of $0$, that is, $f(x)=\sum_{n\geq 0}a_n x^n$ for $x> 0$ small. I want to know the value of $a_1$. Let $z=e^{-x}$, $g(z):=\sum_{k\geq 0}(-1)^k z^{(2k+1)^2}$; then, formally, $g'(1)$ is what we want to compute. Note that $1$ is on the circle of convergence of $g$ so differentiating term-wise is not guaranteed.
-
1$\begingroup$ Just differentiate $f(x)$ element-wise, then input $x = 0$ (then add all the formal "what I did was ok, because..."). As mathoverflow deals with research level questions, you might want to try at math.SE. However, note that also there you will need your own approaches. $\endgroup$– DirkCommented Jun 8, 2017 at 9:38
-
1$\begingroup$ @Bemte: I am afraid you may have judged this question too quickly. $\endgroup$– js21Commented Jun 8, 2017 at 9:53
-
4$\begingroup$ @Menglin: a formal expansion of the exponentials suggests that $a_n = (-1)^n n!^{-1} L(-n,\chi)$ where $\chi $ is the nontrivial Dirichlet characer of odrer $4$. One can rigorously prove an asymptotic expansion $f(x) = \sum_{n=0}^N a_n x^n + o(x^N)$, with the $a_n$ as above, by writing the exponentials as an inverse Mellin transform of $\Gamma(s)$, and by moving the line of integration to the left using the residue theorem. $\endgroup$– js21Commented Jun 8, 2017 at 9:59
-
2$\begingroup$ @მამუკაჯიბლაძე: Indeed. I also made (while editing) the very similar observation that "supposing" that $f(x)$ has a holomorphic continuation to a neighborhood of zero won't help if that is actually false. $\endgroup$– Christian RemlingCommented Jun 8, 2017 at 23:28
-
1$\begingroup$ Tried to make some plots, looks like it cannot be continued beyond $\operatorname{Re}(x)>0$ $\endgroup$– მამუკა ჯიბლაძეCommented Jun 9, 2017 at 12:16
3 Answers
We can indeed prove, as already suspected by მამუკა ჯიბლაძე, that $g(z)=\sum (-1)^k z^{(2k+1)^2}$ cannot be holomorphically continued past $z=1$. In fact, every point on $|z|=1$ is singular. This is a consequence of the following rather general version of the classical results on lacunary power series:
Theorem: Suppose that $a_n$ is bounded. Suppose further that there exists a sequence $n_j\to\infty$ such that: (1) $|a_{n_j}|\ge\delta>0$; (2) $a_{n_j-k}\to 0$ as $j\to\infty$ for every fixed $k\ge 1$. Then $\sum a_n z^n$ cannot be holomorphically continued to any open set larger than the unit disk.
(Note that $R=1$ under these assumptions.)
Of course, this applies to our power series, with $n_j=(2j+1)^2$.
A very elegant proof of the Theorem was recently given by Breuer-Simon. See reference 328 here, Theorem 1.6 of the paper.
-
$\begingroup$ From numerical experiments, $g$ seems to have well-defined radial limits at all roots of unity. It tends to infinity very quickly along radii to $e^{2\pi i\frac pq}$ with both $p$ and $q$ odd, and to certain finite limit when only one of $p$, $q$ is even. $\endgroup$ Commented Jun 10, 2017 at 8:42
-
$\begingroup$ In fact, from the Coogan-Ono paper I linked in a comment to the question, one has$$g(z)=\frac{z}{1+z^2}+\frac{z^3 \left(1-z^2\right)}{\left(1+z^2\right) \left(1+z^6\right)}+\frac{z^5 \left(1-z^2\right) \left(1-z^6\right)}{\left(1+z^2\right) \left(1+z^6\right) \left(1+z^{10}\right)}+\frac{z^7 \left(1-z^2\right) \left(1-z^6\right) \left(1-z^{10}\right)}{\left(1+z^2\right) \left(1+z^6\right) \left(1+z^{10}\right) \left(1+z^{14}\right)}+...$$ $\endgroup$ Commented Jun 10, 2017 at 9:02
-
$\begingroup$ and similarly to what Zagier does in his paper "Vassiliev invariants and a strange identity related to the Dedekind eta-function", I believe one can prove that these radial limits coincide with the result of substituting roots of unity in the above series (on roots of unity this actually terminates, giving infinity on "odd-odd" roots of unity and a well-defined explicit expression on all other roots of unity) $\endgroup$ Commented Jun 10, 2017 at 9:05
-
$\begingroup$ Hey, can I ask how $|a_{n_j-k}|\rightarrow 0$ in our case? I thought $a_{i}=(-1)^{i}$. Not sure what I am missing at here... $\endgroup$ Commented Jun 13, 2017 at 2:06
-
1$\begingroup$ @Bombyxmori: $a_n=0$ unless $n=(2j+1)^2$. $\endgroup$ Commented Jun 13, 2017 at 16:47
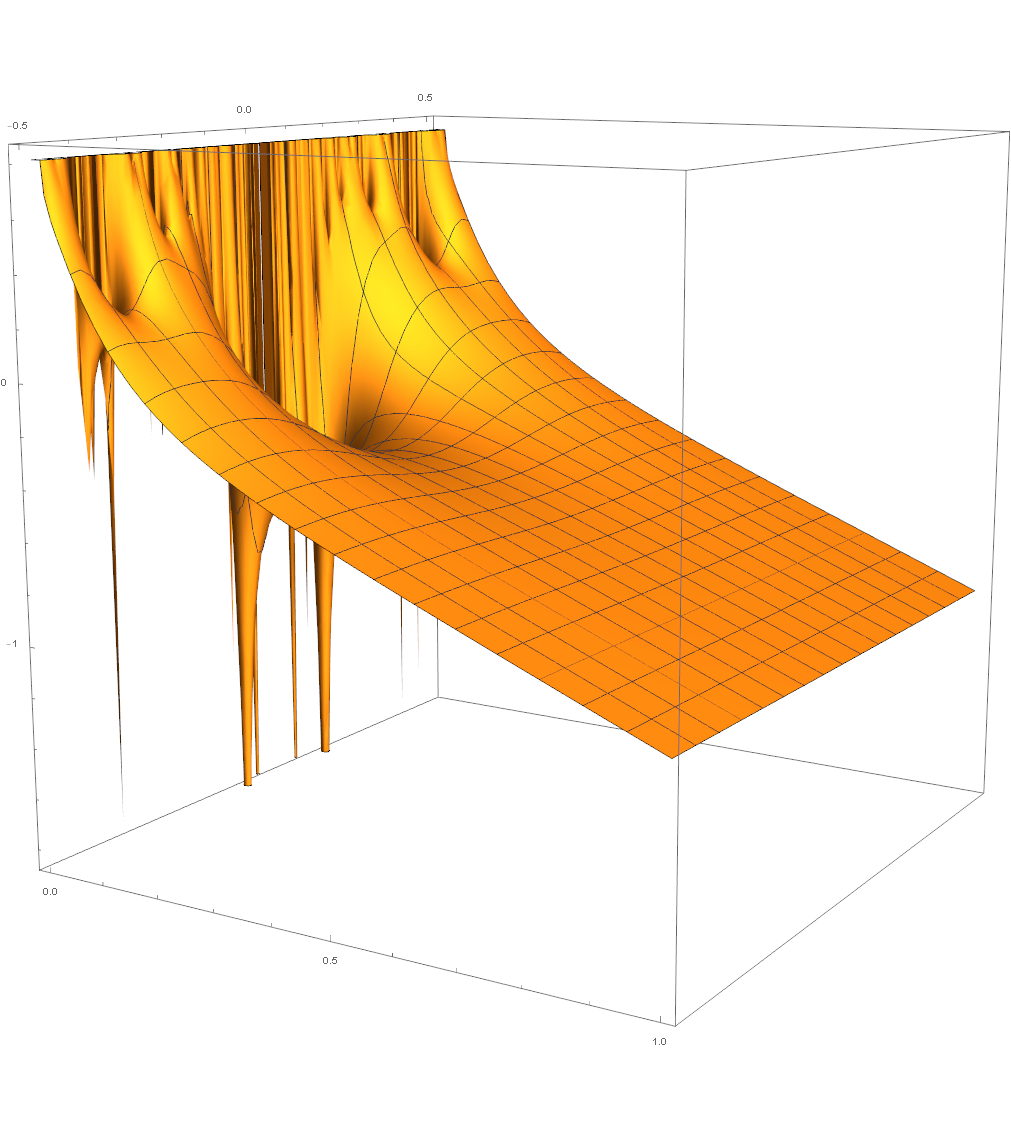
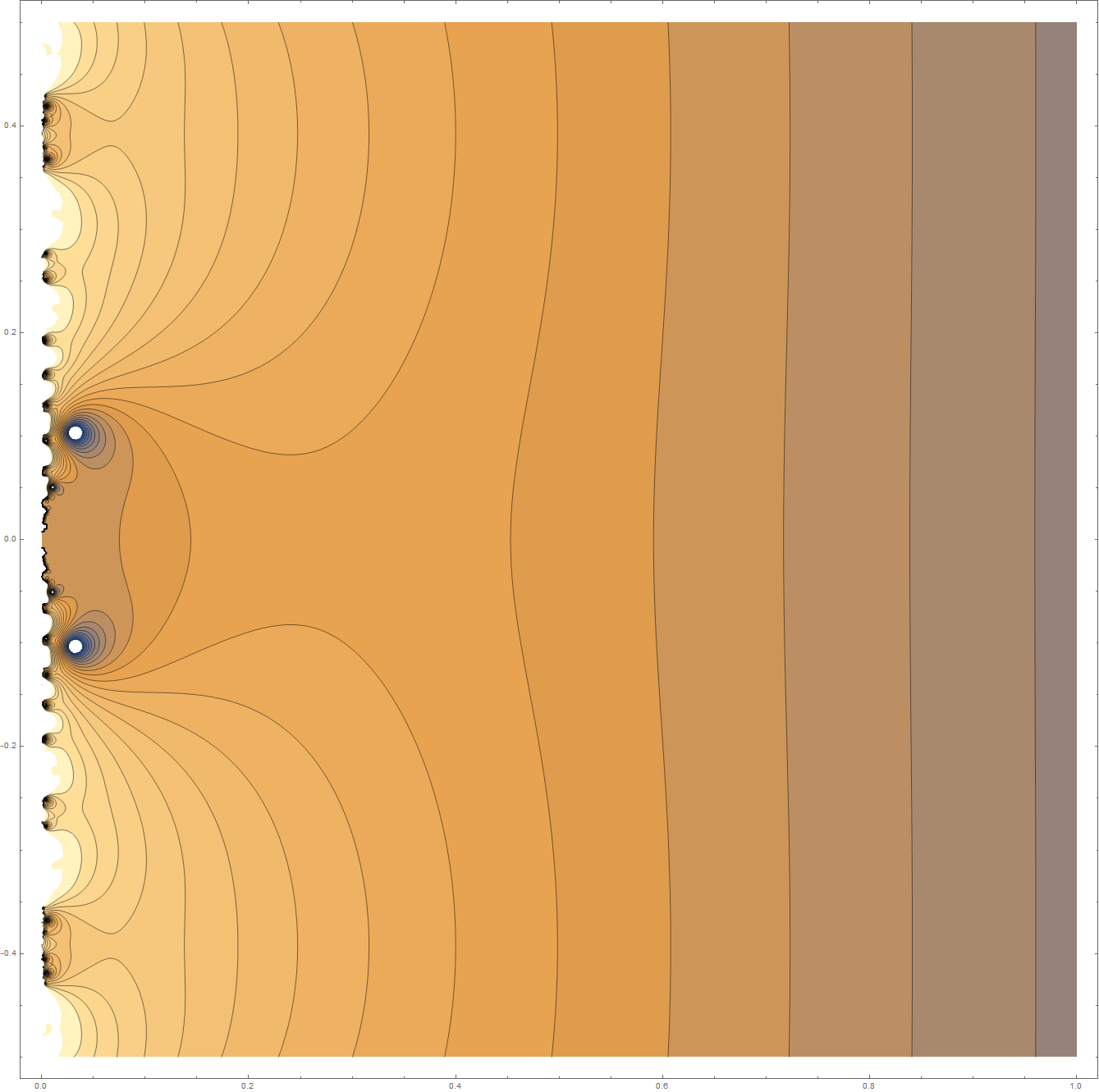
Rather than an answer, this is (I believe) an evidence of analyticity boundary along the imaginary axis. Here are plots for the logarithm of the absolute value of $f(x)$:
(can be enlarged by clicking twice)
(not finished) We have $$ g(z)=\sum_{k=0}^\infty z^{(4k+1)^2}-z^{(4k+3)^2}=(1-z)\sum_{k=0}^\infty \left(z^{(4k+1)^2}+\dots+z^{(4k+3)^2-1}\right)=\\ =\frac12+(1-z)\sum_{k=0}^\infty \left(z^{(4k+1)^2}+\dots+z^{(4k+3)^2-1}\right)-\frac12\left(z^{(4k+1)^2-1}+\dots+z^{(4k+5)^2-2}\right). $$ Thus $(2g(z)-1)/(1-z)$ evaluated at $z=1$ (that is, $2g'(1)$) is an Abel sum of the corresponding alternating series, which, I guess, converges by certain multiple Cezaro.