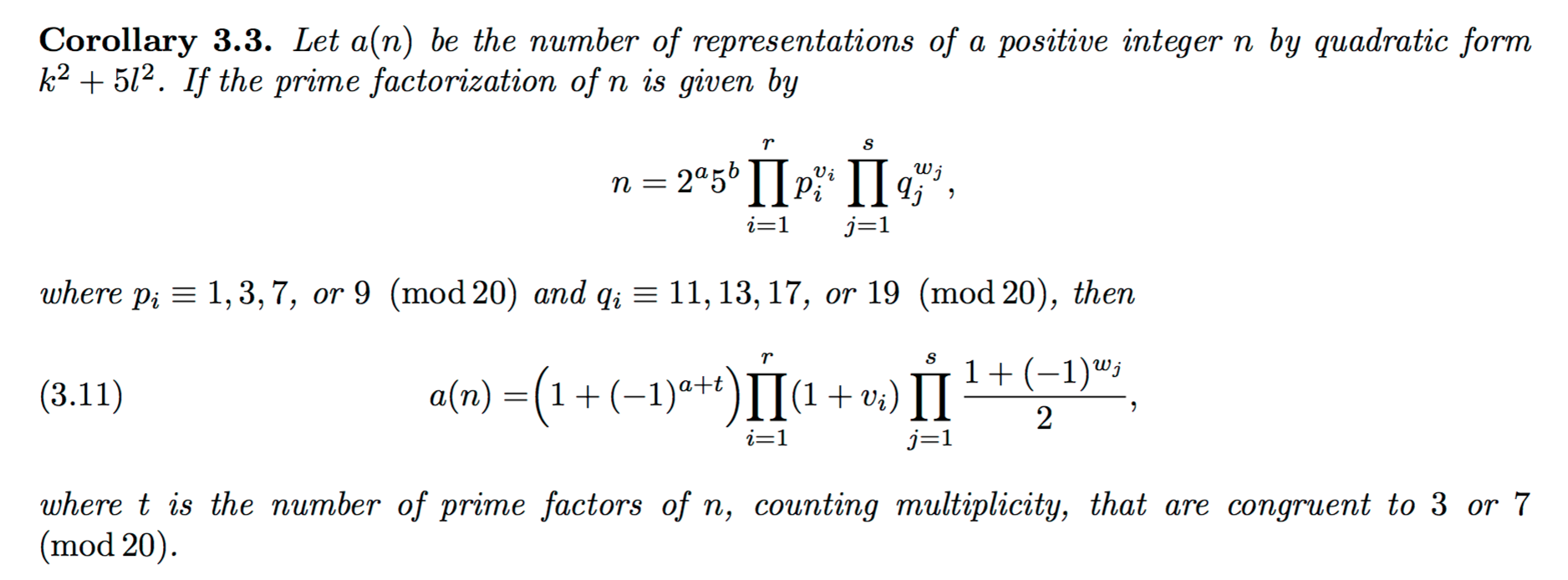
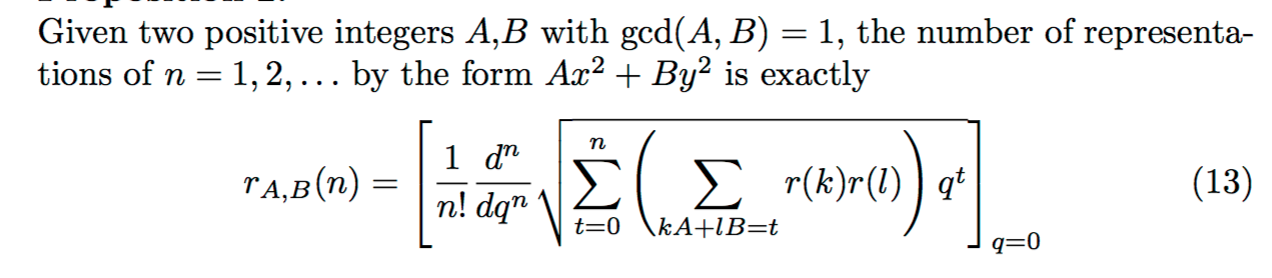Using class field theory one can prove for every integer $n>0$ there exists a monic polynomial $f$ of degree $h(-4n)$* such that for any odd integer $m$ coprime to $n$ we have the following equivalence:
$$\exists x,y\in\mathbb{Z}:(m=x^2+ny^2)\iff \exists x,y\in\mathbb{Z}:(f(x)\equiv 0\bmod m)\land (y^2\equiv-n\bmod m)$$
However depending on the polynomial $f$ this may or may not readily translate into a "nice" sum of Jacobi symbols, namely any expression counting solutions of this form is going to likewise need to count adjusted roots in $f$ modulo primes dividing $m$ which can then be lifted back to solutions with Henzels lemma and with the Chinese remainder theorem (even ignoring other problems). Though we can as I said find similar "nice" formulas for special cases e.g. the particular values of $n$ which force certain pdbqf's of discriminate $D=-4n$ to lie in the same genus, in particular when all the forms are in the same genus as the principal form i.e. they range over the same values in $(\mathbb{Z}/D\mathbb{Z})^{\times}$ we find that it is possible to fully characterize the primes $p$ with solutions $x,y\in\mathbb{Z}$ to $p=x^2+ny^2$ using only conditions on the residues of $p$ modulo fixed integers. For example we can show that:
$$\exists x,y\in\mathbb{Z}:p=x^2+6y^2\iff p\equiv 1, 7\bmod 24$$
$$\exists x,y\in\mathbb{Z}:p=x^2+21y^2\iff p\equiv 1,25,37\bmod 84$$
$$\exists x,y\in\mathbb{Z}:p=x^2+15y^2\iff p\equiv 1, 19, 31, 49\bmod 60$$
Though despite this the formula $r_2(n)=4(d_1(n)-d_3(n))$ can be generalized to counting proper representations of any integer $n$ by all the positive definite binary quadratic forms of an arbitrary fixed discriminant which can also be expressed as a similar divisor like sum involving Jacobi symbols using Dirichlet's Mass formula. While since the arbitrary representations of any $n\in\mathbb{N}$ by a quadratic form are proper representations of $n/d$ coordinate wise scaled by $\sqrt{d}$ for a square $d\mid n$. We can further prove that if $r_2(n)=4(d_1(n)-d_3(n))$ is the number of integer pairs $(x,y)\in\mathbb{Z}^2$ which satisfy $x^2+y^2=n$ whereas if $s(n)=|\{d\mid n:\sqrt{d}\in\mathbb{N}\}|=\prod_{p\mid n}\left(1+\lfloor v_p(n)/2\rfloor\right)$ is the number of perfect squares dividing $n$ and $\omega(n)=\sum_{p\mid n}1$ is the number of distinct primes dividing $n$ then if for every integer $m$ coprime to $n$ we define:
$$w(m)=\begin{cases}6&\text{ if }m=-3\\4&\text{ if }m=-4\\2&\text{ otherwise}\end{cases}$$
As well as the indicator function:
$$e_m(n)=\prod_{p\mid n}\frac{1}{2}\left(1+\left( \dfrac{m}{p}\right)\right)=\begin{cases}1&\text{ if }\exists x\in \mathbb{Z}:x^2\equiv m\bmod n\\0&\text{ if }\not\exists x\in \mathbb{Z}:x^2\equiv m\bmod n\end{cases}$$
We know if $\mathscr{F}_D$ is the set of reduced positive definite binary quadratic forms of discriminant $D$ so that by definition $h(D)=|\mathscr{F}_D|$ then for an arbitrary odd integer $n\in\mathbb{N}$ coprime to $D$ we must get that:
$$\small |\{(x,y,f)\in \mathbb{Z}^2\times\mathscr{F}_D:f(x,y)=n\land \gcd(x,y)=1\}|=w(D)\sum_{d\mid n}\left( \dfrac{D}{d}\right)=w(D)e_D(n)2^{\omega(n)}$$
$$\small\implies |\{(x,y,f)\in \mathbb{Z}^2\times\mathscr{F}_D:f(x,y)=n\}|=w(D)\sum_{d\mid n}\left( \dfrac{D}{d}\right)s(d)=w(D)e_D(n)(d_1(n)-d_3(n))$$
Thus in particular if $D=-4$ we get $f(x,y)=x^2+y^2$ is the only reduced form of discriminant $D$ so these formula simplify to your original identity for counting the number of representations of any integer as a sum of two squares. Whereas note if $D=-28$ then $f(x,y)=x^2+7y^2$ is the only reduced form of discriminant $D$ because $h(-28)=1$ therefore for every odd integer $n>0$ not divisible by $7$, when there exists integers $a,b\in\mathbb{Z}$ such that $n=a^2+7b^2$ then we must have by both of our previous formula that:
$$|\{(x,y)\in\mathbb{Z}^2:x^2+7y^2=n\land \gcd(x,y)=1\}|=2^{\omega(n)+1}$$
$$|\{(x,y)\in\mathbb{Z}^2:x^2+7y^2=n\}|=2(d_1(n)-d_3(n))$$
For details on reduction theory read Chapter 4 of this: http://www2.math.ou.edu/~kmartin/ntii/ntii.pdf Whereas for Dirichlet's formula read page 5 of this: http://www2.math.ou.edu/~kmartin/ntii/chap4.pdf For a more general approach that includes material discussed at the start, try this book by David Cox.