Let $P$ be a finite set of points on a unit-radius sphere $S$ in $\mathbb{R}^3$. Treat $P$ as a fixed pattern that can be rigidly slid around $S$ as a unit (no reflection).
Let $R$ be a subset of $S$. Say that $P$ fits in $R$ if there is some placement of $P$ such that each point of $P$ is strictly in the interior of $R$. Finally, say that $R$ avoids $P$ if $P$ cannot fit in $R$.
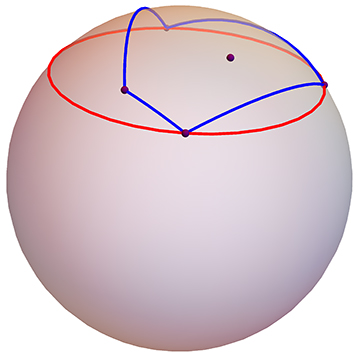
For example, let $P$ be the $n{=}5$ points shown below; I've drawn in the convex hull (blue) and an enclosing circle (red) for perspective. A hemisphere does not avoid $P$, because $P$ easily fits in a hemisphere.
My question is:
Q. What is the largest-area region $R$ that avoids a given set $P$?
Here area is the measure of $R$.
The convex hull of $P$ avoids $P$ (above, the hull is the illustrated quadrilateral formed of (blue) geodesic arcs), because the vertices of the hull are not strictly interior to the hull. A larger region that avoids $P$ is the smallest enclosing disk, whose boundary is outlined in red above. But I believe this is not the largest $R$ that avoids this particular $P$, for a slightly larger disk with an annular hole to "capture" the interior $5$th point has larger area.
The problem feels different if (a) $P$ fits in a hemisphere, and (b) all points of $P$ lie on its convex hull.
It is likely this question has been considered before, in which case I'd appreciate a reference. The same question may be asked for spheres in $\mathbb{R}^d$ for arbitrary $d$.
Update (23Oct15). I posed this question at the Canadian Conference on Computational Geometry, and a participant, Alexandru Damian, proved that, for an $n$-point set $P$, the area of an avoiding set cannot be larger than $\frac{n-1}{n} A$, where $A$ is the area of the sphere. The argument is sketched here (PDF download). The bound is tight for $n=2$ antipodal points.
