1
$\begingroup$

It is intuitive to see that the 3-components links (under Alexander–Briggs notations) $6^3_1, 6^3_2, 6^3_3$ are closely related to each other; in a sense by doing a cut-gluing or sew-gluing surgery, we can transform one to the others.

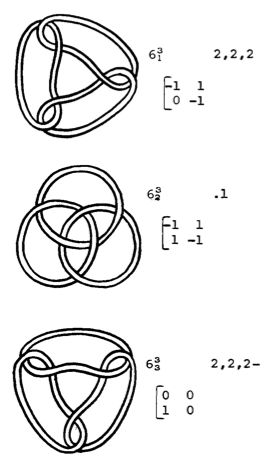
enter image description here

Similarly, one can also do the surgery to transform $6^3_1, 6^3_2, 6^3_3$ to $3_1$ trefoil knots. This surgery is especially intuitive from $6^3_2$ to $3_1$.

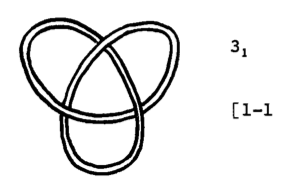
enter image description here

So my question is that what is the mathematical procedure to formulate this surgery? Are those procedures fully captured by Reidemeister moves? And what are the explicit math relations to transform $6^3_1, 6^3_2, 6^3_3$, $3_1$ to each other?

For example, one can write direct sums of Hopf links as $2^2_1\# 2^2_1$ to form a new 3-component non-prime links. it may be the easiest one in the case of $$6^3_2 \to 3_1.$$ There should be also some considerations of orientations and framing for the others: $$6^3_1 \to 3_1,$$ $$6^3_3 \to 3_1.$$

p.s. It will be greatly appreciated that if someone please makes the statement explicitly and as simple as possible. (excuse me, I am a physicist.) Thank you.

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ I think the relation is more natural in high dimensions. For example, there is a non-trivial 3-component link $\sqcup_3 S^3 \to S^6$ which when you take the connect-sum of all three components gives you the simplest non-trivial smooth embedding $S^3 \to S^6$. This is an old result of Haefliger's. $\endgroup$
    – Ryan Budney
    Commented Apr 21, 2014 at 3:11
  • $\begingroup$ Indeed Ryan, thanks for the comment. $\endgroup$
    – wonderich
    Commented Apr 21, 2014 at 3:16
  • 2
    $\begingroup$ Cross-posted: math.stackexchange.com/questions/763496/… $\endgroup$
    – Ryan Budney
    Commented Apr 23, 2014 at 19:19
  • 2
    $\begingroup$ This question appears to be off-topic because it is cross-posted on MSE. $\endgroup$
    – Stefan Kohl ♦
    Commented Apr 23, 2014 at 19:47
  • 1
    $\begingroup$ Dear @Idear: I don't know whether this question would be accepted if it had not been asked at Math.StackExchange. I voted to close because simultaneous posting of questions on multiple sites leads to duplication of effort. It is better to pick one site for your question and wait at least a few days for an answer. $\endgroup$
    – Ricardo Andrade
    Commented Apr 24, 2014 at 5:06

0

Reset to default

Browse other questions tagged .