Chirality and Anti-Chirality of links in 3 and in 5 dimensions

Question

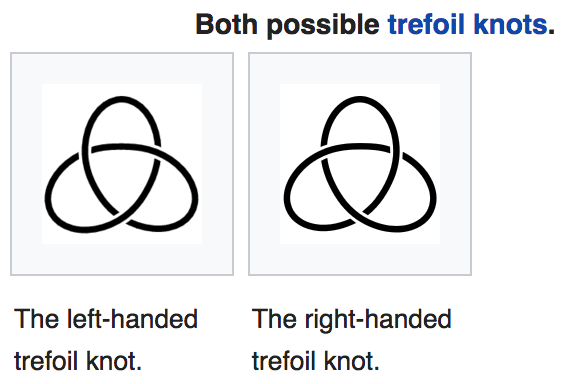
We know there is a chiral knot which is a knot that is not equivalent to its mirror image. It is well known in the mathematical field of knot theory: https://en.wikipedia.org/wiki/Chiral_knot

My first question is about

(1) the literature and the References on

• the chirality of link in 3 dimensions

• the chirality of link in 5 dimensions

What are some good text/Refs on the topological invariants of these chiralities of links of 1-submanifolds in 3 dimensions? (addressed somewhere in the literature?)

For example, in 5 dimensions, let me consider a 5-sphere $S^5$. Let me define a new quartic link Q of 5-dimensions in $S^5$: such that $\Sigma^3_{W_{{(i)}}},\Sigma^3_{W_{{(ii)}}},\Sigma^3_{W_{{(iii)}}}$ are 3 sets of 3-submanifolds, while the $\Sigma^2_U$ is a 2-surface. Let $V^4_{W_{{(i)}}}, V^4_{W_{{(ii)}}}, V^4_{W_{{(iii)}}}, V^3_U$ be their Seifert volumes in one higher dimensions.

Are these chiralities of link of 2-submanifolds and 3-submanifolds in 5 dimensions also addressed somewhere in the literature?

There can be a link invariant defined in this manner: $$ { \#(V^4_{W_{{(i)}}}\cap V^4_{W_{{(ii)}}}\cap V^4_{W_{{(iii)}}}\cap V^3_U)\equiv\text{Q}^{(5)}(\Sigma^3_{W_{{(i)}}},\Sigma^3_{W_{{(ii)}}},\Sigma^3_{W_{{(iii)}}},\Sigma^2_U)} $$

I am suspecting there could be a opposite chirality of this invariant defined as $\overline{\text{Q}^{(5)}}$ as follows: $$ { \#(V^4_{W_{{(ii)}}}\cap V^4_{W_{{(i)}}}\cap V^4_{W_{{(iii)}}}\cap V^3_U)+\dots \equiv\overline{\text{Q}^{(5)}}(\Sigma^3_{W_{{(ii)}}},\Sigma^3_{W_{{(i)}}},\Sigma^3_{W_{{(iii)}}},\Sigma^2_U)} $$

(2) Do similar chirality and anti-chirality of link invariants in 3 dimensions, happening for example to Borromean rings? Or other Brunnian links? Examples and References are welcome.

Answer 1

With regard to the 5-dimensional part of the question, there are plenty of obstructions to chirality (and variations thereof like invertibility.) This is best developed in the case of knots. For instance, in the classical dimension one can show the trefoil to be chiral by using the signature, or more generally using Tristram-Levine signatures. There are similar signature invariants defined for knots in 5-space, and they can be used in similar ways. (You have to be careful because the forms whose signatures are computed have different symmetries in dimensions $1 \pmod{4}$ as compared to $-1 \pmod{4}$.)

There is a fair amount on this in the knot and link theory literature from the 70's and 80's. You can find some useful information in chapter 9 of Hillman's book, Algebraic invariants of links, and in his references.

Answer 2

This answer will only be about the 3-dimensional case.

The book

Burde, Gerhard; Zieschang, Heiner, Knots, De Gruyter Studies in Mathematics, 5. Berlin - New York: Walter de Gruyter. XII, 399 p. DM 49.95 (1985). ZBL0568.57001.

is a pretty good resource for chirality/amphichirality of knots and links. For example, these issues are discussed at least 8 times (according to the index) and it is usually in the context of some examples, e.g. Theorem 3.29(b) torus knots are not amphichiral. Pages 310-318 have a number of examples were chirality is explicitly computed.

Finally, the software for working with hyperbolic 3-manifolds, SnapPy computes chirality via a computation in the symmetry group (the example below is for the Borromean rings $6^3_2$ in Rolfsen's notation):

In[27]: L = Manifold('6^3_2')
In[28]: G = L.symmetry_group()
In[29]: G.is_amphicheiral()
Out[29]: True

I should point out `out of the box' SnapPy uses an approximation of a hyperbolic structure and the symmetry group computation tries to find symmetries of the canonical cell decomposition relative to this structure, so it is subject to computational error. Specifically, for more complicated examples, SnapPy could return a subgroup of the symmetry group. In principal this could omit an orientation reversing symmetry that would establish amphi-chirality.

If you are concerned about this, SnapPy's development team offers a version which runs in sage (https://www.math.uic.edu/t3m/SnapPy/verify.html) not subject such errors of omission.