I believe Misha's comment directly addresses some of the problems with you question as stated. However, let me say a few words about the issues here and then mention some observations that might help you refine your ideas of your question.
Ignoring the issues of base points (which are also important) and instead concerning ourselves with only free homotopy classes of curves still leaves some issues. In the picture below, consider the green curve as $a$. The homotopy class of $a$, $\alpha$ is trivial, and so it commutes with everything in $\pi_1(M)$. 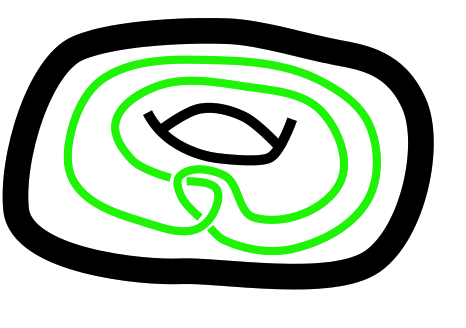
(This picture highlights one distinction between homotopy classes and isotopy classes.)
Also, in this case the underlying manifold is $S^1 \times D^2$ so all elements in the fundamental group commute. In fact, we could choose a more complicated manifold, say a Seifert fibered space, and still see elements commuting. For example, Seifert fibered spaces have non-trivial center. Thus, choosing $M$ to be a Seifert fibered space and $\alpha$ to be in the center of $\pi_1(M)$ will give the desired $a$ and $b$. Again, this has nothing to do with the isotopy classes of the knots $a$ and $b$.

