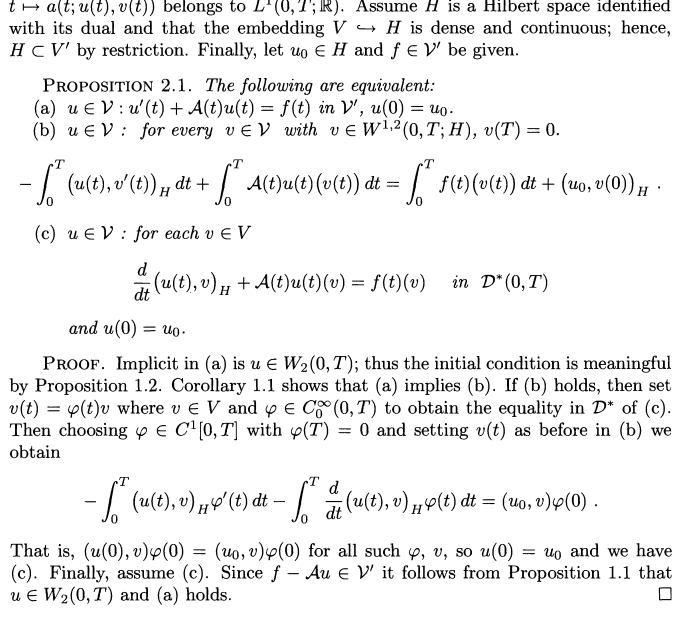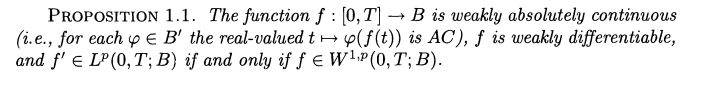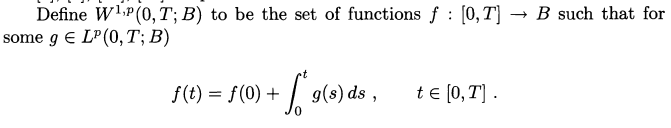In my opinion, OP has not given enough information in order for the question to be able to be answered properly. Maybe the author has made somewhere implicit assumptions that are not explicitly stated in Proposition 2.1. In (c) of that proposition, nothing implies that the weak derivative of $u$, interpreted as a function $[0,T]\to V'$ (or more properly, an a.e. equivalence class of such) would be in (possibly) the space $L^2([0,T],V')$. However, something like this would be needed to apply Proposition 1.1.
If we knew that $\mathcal A\,{.\,}u:t\mapsto\mathcal A(t)u(t)$ is in $L^2([0,T],V')$, and if we knew that $V$ is reflexive, then (c) would imply that $u:[0,T]\to V'$ is weakly differentiable with the weak derivative in $L^2([0,T],V')$. This also would give the superfluous assumption of weak absolute continuity, provided that continuity of $u:[0,T]\to V'$ is implicit somewhere. Thence $u$ being in $W^{1,2}([0,T],V')$ would follow. The definition of the latter space then directly would give (a) of Proposition 2.1.
Remark. OP has not given what the author means by $f:[0,T]\to B$ being weakly differentiable. Above, I have interpreted it so that for every $v\in B'$ the function $v\circ f:[0,T]\owns t\mapsto v(f(t))\in\mathbb R$ is differentiable in the distributional sense with derivative $v\circ g$ for some $g:[0,T]\to B$.