Let $ABC$ be arbitrary triangle, $D$, $E$, $F$ are the midpoints of $BC$, $CA$, $AB$ respectively. Define points, segments in the figure below. I am looking for a proof that:
$$DE+EF+FD \le (DG+DH+EI+EJ+FJ+FQ).\frac{\varphi}{2}$$
Wher $\varphi=\frac{\sqrt{5}+1}{2}$ the golden ratio.
Equality if only if $ABC$ is the equilateral triangle.
See also:
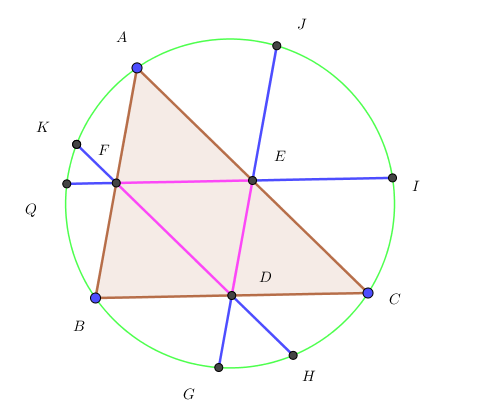
Let $DH=x$, $DG=y$, $FK=z$, $FQ=t$, $EG=u$ and $EI=v$.
Thus, in the standard notation we obtain: $$u\left(y+\frac{c}{2}\right)=\frac{b^2}{4},$$$$y\left(u+\frac{c}{2}\right)=\frac{a^2}{4},$$ Which gives $$u-y=\frac{b^2-a^2}{2c},$$ $$y\left(y+\frac{b^2-a^2}{2c}\right)+\frac{c}{2}y=\frac{a^2}{4},$$ $$y=-\frac{b^2+c^2-a^2}{4c}+\sqrt{\left(\frac{b^2+c^2-a^2}{4c}\right)^2+\frac{a^2}{4}},$$ $$u=-\frac{a^2+c^2-b^2}{4c}+\sqrt{\left(\frac{b^2+c^2-a^2}{4c}\right)^2+\frac{a^2}{4}}$$ and by C-S we have: $$u+y=-\frac{c}{2}+\sqrt{\left(\frac{b^2+c^2-a^2}{2c}\right)^2+a^2}=$$ $$=-\frac{c}{2}+\sqrt{\frac{(a^2-b^2)^2+c^2(c^2+2a^2+2b^2)}{4c^2}}\geq-\frac{c}{2}+\frac{1}{2}\sqrt{c^2+2a^2+2b^2}=$$$$=-\frac{c}{2}+\frac{1}{2\sqrt5}\sqrt{(1+2+2)(c^2+2a^2+2b^2)}\geq-\frac{c}{2}+\frac{c+2a+2b}{2\sqrt5}.$$ By the same way we obtain: $$v+t\geq-\frac{a}{2}+\frac{a+2b+2c}{2\sqrt5}$$ and $$x+z\geq-\frac{b}{2}+\frac{b+2a+2c}{2\sqrt5}.$$ Can you end it now?
