A generalization of Barrow's inequality

Question

More than seven years ago. I posted this problem in stackexchange:

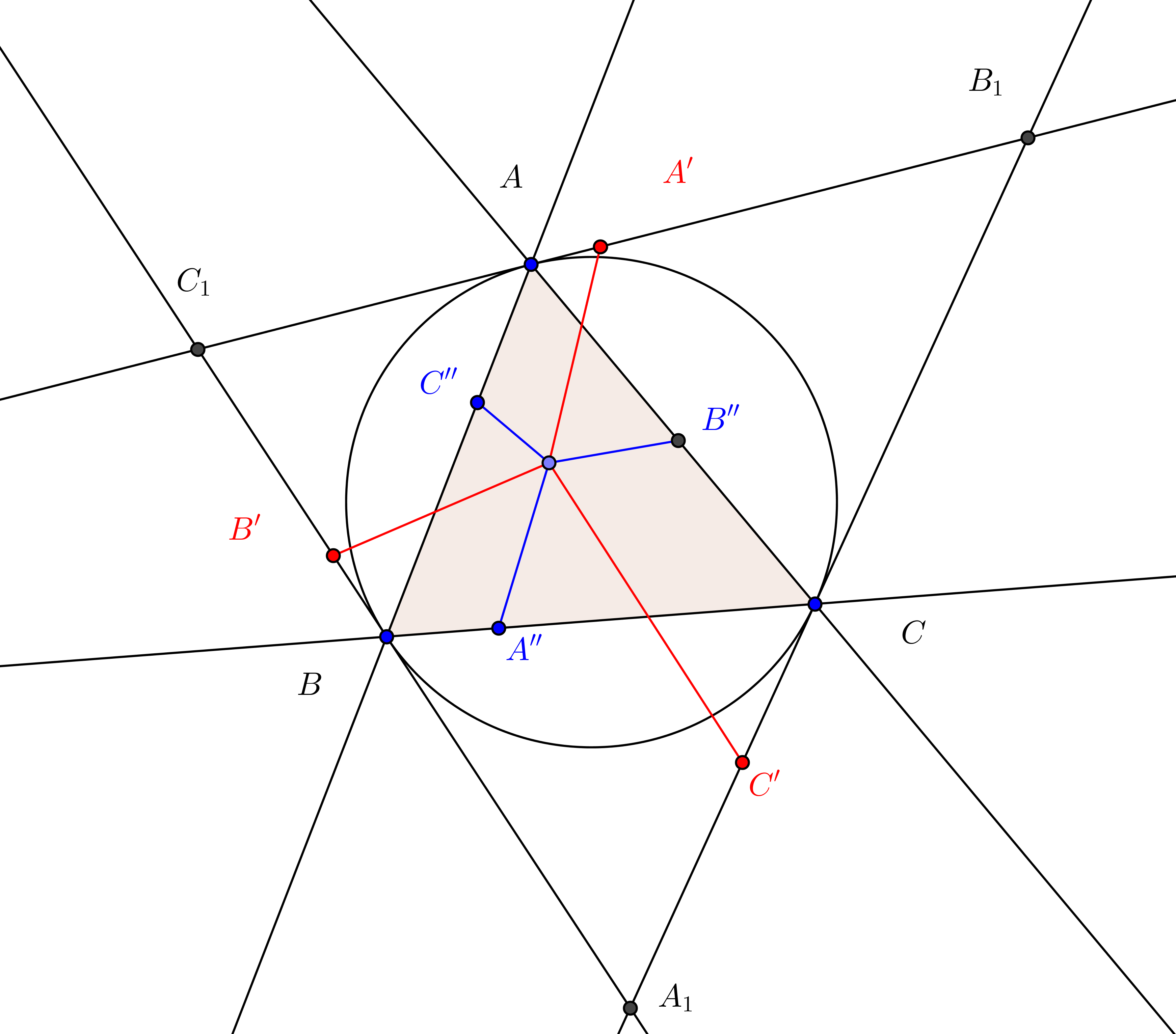
1. Let $ABC$ be a triangle, $P$ be arbitrary point inside of $ABC$. Let $A_1B_1C_1$ be the tangential traingle of $ABC$. Let $A'$, $B'$, $C'$ be three be arbitrary points on $B_1C_1, C_1A_1, A_1B_1$ respectively. Let internal angle bisectors of three angles $\angle B'PC'$, $\angle C'PA'$, $\angle A'PC'$ meet $BC, CA, AB$ at $A'', B'', C''$ respectively. Then $$PA'+PB'+PC' \ge 2(PA''+PB''+PC'')$$.

2. Equality hold iff ABC be an equilateral triangle and $\angle B'PC'= \angle C'PA'=\angle A'PC' =120^0$

3. When $A' \equiv A, B' \equiv B, C' \equiv C$ we have Barrow's inequality

I am looking for a proof the conjecture.

See also:

• Erdős–Mordell inequality
• Barrow's inequality

Answer 1

Let us fix the directions of the blue and red segments and consider the function $$f(P)=PA’+PB’+PC’-2(PA’’+PB’’+PC’’).$$It is easy to see that it will be linear, therefore, to prove the inequality $f(X)\geqslant 0$, it is necessary to check it at the vertices A, B, C. Let us check at the vertex A (similarly at the other two). Let us prove a stronger inequality: $AB’\cdot AC’ \geqslant (AA’’)^2$. Let us put a point $X$ on $AC’$ so that $AB’\cdot AX= (AA’’)^2$. Note that the composition of the inversion with center $A$ and radius $AA’’$ and symmetry with respect to $AA’’$ maps $B’$ to $X$, then $X$ lies on the image of the tangent at point $B$ to the circle $(ABC)$. Let us show that this circle $(DAE)$, $D$ is a point on the tangent to the circle $(ABC)$ at point $C$, such that $AA'CD$ is cyclic, $E$ is a point on $AA'$, such that $DE\parallel CB$. This is easy to understand, since $AD_0E_0$ is similar to $AED$, and $AD_0A’’$ and $AA’’D$ are also similar, $D_0$ is a point on the tangent to the circle $(ABC)$ at point $B$, such that $AA'BD_0$ is cyclic, $E_0=AA' \cap BD_0$. Note (this is obvious) that circle $(DAE)$ is tangent to $CE$, then $X$ lies on the segment $AC’$. Otherwise, $PA’’$ is the external, not the internal, bisector of angle $B’PC’$. Thus, $AC’ \geqslant AX$, which gives us the solution of the problem.

Picture for the solution: