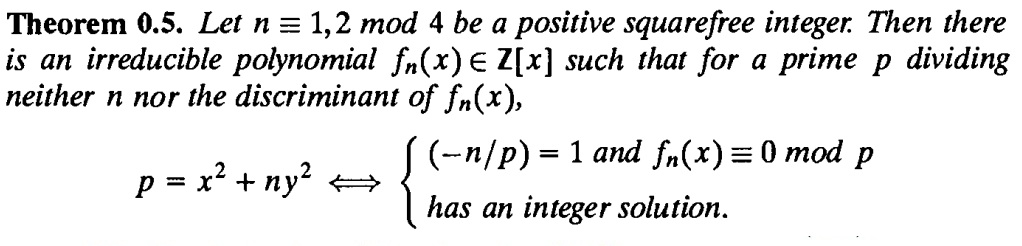6
$\begingroup$

A SPECIFIC CASE:

Any prime number can be classified as either $p \equiv 1 \pmod 3$ or $p \equiv 2 \pmod 3$.

If $p = 3$ or $p = 1 \pmod 3$, then the prime $p$ can be represented by the quadratic form $ x^2 + 3y^2, x,y \in \mathbb Z.$

But what if $p \equiv 2 \pmod 3$?

Is there a quadratic form $ax^2+bxy+cy^2$ such that $p= ax^2+bxy+cy^2, $ when $p \equiv 2 \pmod 3$ where $x,y, a, b,c \in \mathbb Z$?

GENERAL CASE:

The general question is, is there a set of quadratic forms which represent all prime numbers?

We will classify the prime numbers, say, by $m$. Any prime is defined by $p \equiv i \pmod m$ where $1 \leq i\leq m-1$.

In above example, $i \in \{1, 2\}, m=3$. Let, the set of quadratic forms is $A$, then the number of elements in $A$ is at-least $(m-1)$.

QUESTION:

For a given $m$ can we find a set $A$ such that any prime $p$ can be represented by one of the quadratic form of $A$ ?

If it is possible then how? If there is a condition on $m$, what is it?

Does the question has any relation to the following theorem ?

enter image description here

One can answer only the specific case, if they wish to do so.

EDIT:

Is there a finite set of (preferably irreducible) binary quadratic forms such that every prime is represented by at least one of the forms in the set?

Improve this question
$\endgroup$
11
  • 2
    $\begingroup$ I'm fairly sure that for any primitive quadratic form and any $m>0$, the form represents infinitely many primes which are $1\pmod m$. This should follow from Chebotarev's density theorem. Therefore there cannot be such a form which only represents non-1 residues modulo a number. $\endgroup$
    – Wojowu
    Commented Oct 11, 2020 at 23:08
  • 3
    $\begingroup$ Actually on second reading, you didn't ask for the form to only represent the forms of specific residue. In that case there are some trivial examples, like the form $xy$, or $x^2-y^2$. I'm not sure if you can do with only irreducible forms. $\endgroup$
    – Wojowu
    Commented Oct 11, 2020 at 23:11
  • 4
    $\begingroup$ If a quadratic form represents $p$, then the discriminant $b^2 -4ac$ of the form is a perfect square modulo $p$. (Proof: The discriminant is invariant under change of variables. Because the form represents $p$, we can change variables so that $a=p$.) So indeed no non-split form represents all primes and sets like the set of primes congruent to $2$ mod $3$ can never be represented. $\endgroup$
    – Will Sawin
    Commented Oct 11, 2020 at 23:25
  • 4
    $\begingroup$ You can certainly get all primes if you are willing to accept overlap between the different quadratic forms. $\endgroup$
    – Will Sawin
    Commented Oct 11, 2020 at 23:37
  • 3
    $\begingroup$ I read the question as permitting overlap. I think the question is, is there a finite set of (preferably irreducible) binary quadratic forms such that every prime is represented by at least one of the forms in the set? $\endgroup$
    – Gerry Myerson
    Commented Oct 12, 2020 at 4:02

1 Answer 1

Reset to default
14
$\begingroup$

Every prime $p$ is represented by at least one of the following quadratic forms: $x^2+y^2$, $x^2+3y^2$, $3x^2-y^2$:

  • if $p=2$ or $p\equiv 1\pmod{4}$, then $p$ is represented by $x^2+y^2$;
  • if $p=3$ or $p\equiv 1\pmod{3}$, then $p$ is represented by $x^2+3y^2$;
  • if $p\equiv 11\pmod{12}$, then $p$ is represented by $3x^2-y^2$.

This follows from Lemma 2.5, Corollary 2.6, (page 26) in Cox: Primes of the form $x^2+ny^2$ coupled with the fact that $x^2+y^2$, $x^2+3y^2$, $3x^2-y^2$, $x^2-3y^2$ represent all integral binary quadratic forms of discriminant lying in $\{-4,\pm 12\}$.

Added. More generally, if an odd number of discriminants multiply to a square, then the quadratic forms of those discriminants together represent all primes coprime to those discriminants. In the example above, the discriminants were the elements of $\{-4,\pm 12\}$, and we could do without the form $x^2-3y^2$. See also this related post.

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ I gave him $x^2 + y^2, x^2+ 2 y^2, x^2 - 2 y^2$ at math.stackexchange.com/questions/3820129/… and suggested he read Dickson's little 1929 book Intro to.. It would appear he refuses to do that. The claim I made there, no finite set of positive binaries could work, is something for which I lack a proof. $\endgroup$
    – Will Jagy
    Commented Oct 12, 2020 at 16:37
  • 2
    $\begingroup$ posted a new question here on MO about the finiteness claim. $\endgroup$
    – Will Jagy
    Commented Oct 12, 2020 at 17:00

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .