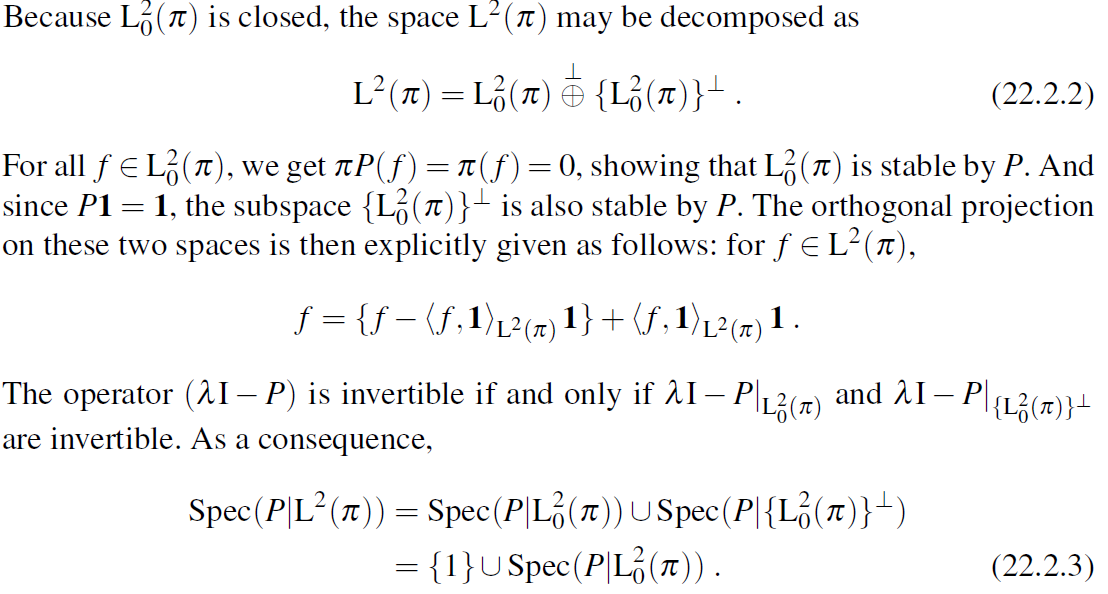
Let $P$ be a Markov kernel on a measurable space $(E,\mathcal E)$ admitting an invariant probability measure $\pi$. $P$ acts on $L^2(\pi)$ via $$Pf:=\int\kappa(\;\cdot\;{\rm d}y)f(y).$$ The invariance means that $\int\kappa f\:{\rm d}\pi=\int f\:{\rm d}\pi$. Let $L^2_0(\pi):=\left\{f\in L^2(\pi):\int f\:{\rm d}\pi=0\right\}$. I've read the following:
The first equality in (22.2.3) holds since they've argued that $L^2_0(\pi)$ is a reducing subspace for $P$. But how does the second equality follow? Moreover, I've often read that $\operatorname{Spec}\left(P\mid L^2_0(\pi)\right)\subseteq[-1,1)$ (so, $1$ is excluded from the spectrum when restricting to $L^2(\pi)$. How does this follow?
Note that $$U:L^2(\mu)\to L^2(\mu)\;,\;\;\;f\mapsto\langle1,f\rangle_{L^2(\mu)}1$$ is an orthogonal projection with $\mathcal N(U)=L^2_0(\mu)$. So, $1-U$ is the orthogonal projection of $L^2(\mu)$ onto ${\mathcal R(U)}^\perp=L^2_0(\mu)$. Now, if $\lambda\in\mathbb R$, then $\lambda-\left.P\right|_{{L^2_0(\mu)}^\perp}$ is injective if and only if \begin{equation}\begin{split}\{0\}&=\mathcal N\left(\lambda-\left.P\right|_{{L^2_0(\mu)}^\perp}\right)\\&=\left\{g\in\mathcal R(U):(\lambda-P)g=0\right\}\\&=\left\{Uf:f\in L^2(\mu)\text{ and }(\lambda-P)Uf=0\right\}\\&=\left\{Uf:f\in L^2_0(\mu)\text{ and }(\lambda-P)Uf=0\right\}\\&\;\;\;\;\;\;\;\;\;\;\;\;\uplus\left\{Uf:f\in L^2(\mu)\setminus L^2_0(\mu)\text{ and }(\lambda-P)Uf=0\right\}\\&=\left\{0\right\}\uplus\left\{\langle1,f\rangle_{L^2(\mu)}1:f\in L^2(\mu)\setminus L^2_0(\mu)\text{ and }\lambda=1\right\}\\&=\left\{0\right\}\uplus\left\{c:c\in\mathbb R\setminus\{0\}\text{ and }\lambda=1\right\}\\&=\begin{cases}\mathbb R&\text{, if }\lambda=1\\\{0\}&\text{, otherwise}\end{cases},\end{split}\tag1\end{equation} where we've used that $P1=1$ (and we treat $c\in\mathbb R$ as the constant function $E\ni x\mapsto c$).
So, we can conclude that $\lambda\in\mathbb R$ is contained in the point spectrum of $\left.P\right|_{{L^2_0(\mu)}^\perp}$ if and only if $\lambda=1$. How can we conclude?