9
$\begingroup$

Q. Is it known what is the thinnest covering of the infinite plane by regular pentagons?

By covering I mean every point of the plane is covered. By thinnest I mean the proportion of the plane covered more than once is minimal among all coverings.

This seems like it must be known, but I cannot find it, perhaps because I don't know the correct terminology.

This is a natural attempt:

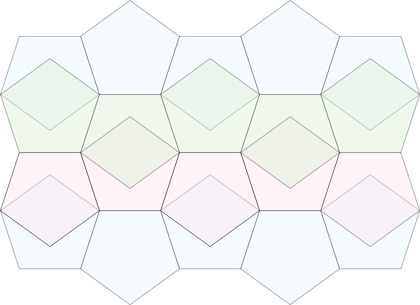
          PentCovering

If I've calculated correctly, this covering doubly covers about $38\%$ of the plane: $$\tfrac{1}{2} \left(3-\sqrt{5}\right) \approx 0.382 \;.$$ I am interested because the above covering can be achieved by "rolling" a dodecahedron, and I'd like to know if there is a thinner cover which might not be "rollable."

Improve this question
$\endgroup$
2
  • $\begingroup$ Suppose you line them up. (The nth pentagon has its base on [n,n+1].). How much space does that overuse? Gerhard "Minding Ones Pents And Gons" Paseman, 2017.11.08. $\endgroup$
    – Gerhard Paseman
    Commented Nov 9, 2017 at 1:03
  • $\begingroup$ @GerhardPaseman: This would not be the best way to do it. See my answer below and click on the link. You may also enjoy the animation auburn.edu/~kuperwl/pent_movie.mp4 showing a continuous transition between the densest packing and this covering. $\endgroup$
    – Wlodek Kuperberg
    Commented Nov 9, 2017 at 2:34

1 Answer 1

Reset to default
7
$\begingroup$

The thinnest known covering of the plane with congruent regular pentagons is shown in my answer to: Terrible tilers for covering the plane. What you see there is probably not "rollable". The covering is of the "double-lattice" type and is known to be the thinnest among double lattice coverings with regular pentagons (to be published). Also, it is conjectured to be the thinnest one among all coverings with congruent regular pentagons. The conjecture is still open.

By the way, Joe, your "natural attempt" is of a double-lattice type also, generated by a trapezoid contained in the pentagon. The trapezoid is a p-hexagon too, but not of maximum area, and the larger the p-hexagon, the thinner the covering generated by it.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Apologies for forgetting your answer to that earlier question also answers this question! $\endgroup$
    – Joseph O'Rourke
    Commented Nov 9, 2017 at 11:10
  • 1
    $\begingroup$ No need to apologize, Joe. The pentagon packing conjecture was confirmed not long ago by Tom Hales, with a computer-aided proof, but the covering conjecture appears to be much harder. Your post helps to draw more attention to it, making it more likely to be solved in some future. Thanks! $\endgroup$
    – Wlodek Kuperberg
    Commented Nov 9, 2017 at 18:30
  • $\begingroup$ A late correction to my earlier comment: The proof of the pentagon packing conjecture is due to Thomas Hales and Wöden Kusner, see arxiv.org/abs/1602.07220 . My apologies to Wöden. $\endgroup$
    – Wlodek Kuperberg
    Commented Dec 18, 2017 at 16:34

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .