[Edited to add a two-parameter family of hexagons]
Here's a family of convex pentagons $K_\epsilon$, each of which
does not tile the plane but does have a perfect $2$-covering
by rotated translates.
Start with a regular hexagon $\,\widetilde{\!H}$.
Its six shorter diagonals form a "star of David" with a smaller hexagon
$H$ at its center whose area is $1/3$ the area of $\,\widetilde{\!H}$.
Now tile the plane with parallel copies of $\,\widetilde{\!H}$,
and remove the corresponding copy of $H$ from the center of each translate.
The resulting region, call it $R$, is shown shaded in the first part
of the image below (with $\,\widetilde{\!H}$ and its translates
outlined in blue, and the star of David in light blue).
This picture shows how $R$ is also the hexagonal tiling by copies of $H$
with every third hexagon removed. Hence three parallel copies of $R$
constitute a perfect $2$-covering of the plane.
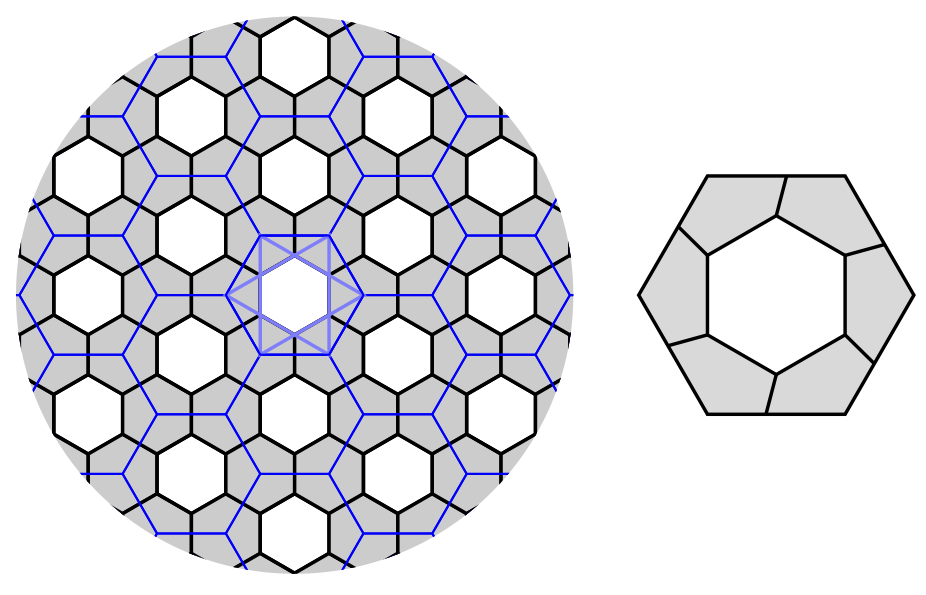
Now each copy of $\,\widetilde{\!H} - H$ is tiled by
six rotations of a pentagon $K_0$ with angles
$120^\circ, 90^\circ, 120^\circ, 90^\circ, 120^\circ$.
So $K_0$ has a perfect $2$-covering. This is not yet our pentagon:
it tiles the plane, because three copies fill $H$ exactly.
But we can deform $K_0$ by rotating its shortest two sides
by $\epsilon$ degrees to a pentagon $K_\epsilon$,
with angles $(120+\epsilon)^\circ, (90-\epsilon)^\circ,
120^\circ, (90+\epsilon)^\circ, (120-\epsilon)^\circ$,
six copies of which still tile $\,\widetilde{\!H} - H$
as shown in the second part of the image. For small but
nonzero $\epsilon$ this $K_\epsilon$ cannot tile the plane,
not even with reflections allowed $-$ a contradiction is soon reached
starting with a neighborhood of a $(120\pm\epsilon)^\circ$ angle.
But $K_\epsilon$ still perfectly $2$-covers the plane
via $\,\widetilde{\!H}-H$ and $R$,
so we have our family of convex pentagons
with a minimal tiling thickness of $2$.
P.S. I later noticed a further modifiction to a two-parameter family of
convex hexagons $K = K_{\epsilon,\delta}$ that do not tile the plane
but do tile $R$ and thus perfectly $2$-cover the plane. Deform each edge of
$\,\widetilde{\!H}$ to the same Z-shaped path ("Z-shaped":
centrally-symmetric concatenation of three line segments).
Call the resulting polygon $\,\widetilde{\!H}'$; it still tiles the plane.
Do not change $H$. Then $\,\widetilde{\!H}' - H$ is tiled by
six rotations of the same convex hexagon $K$, as seen in the
first part of the next image:
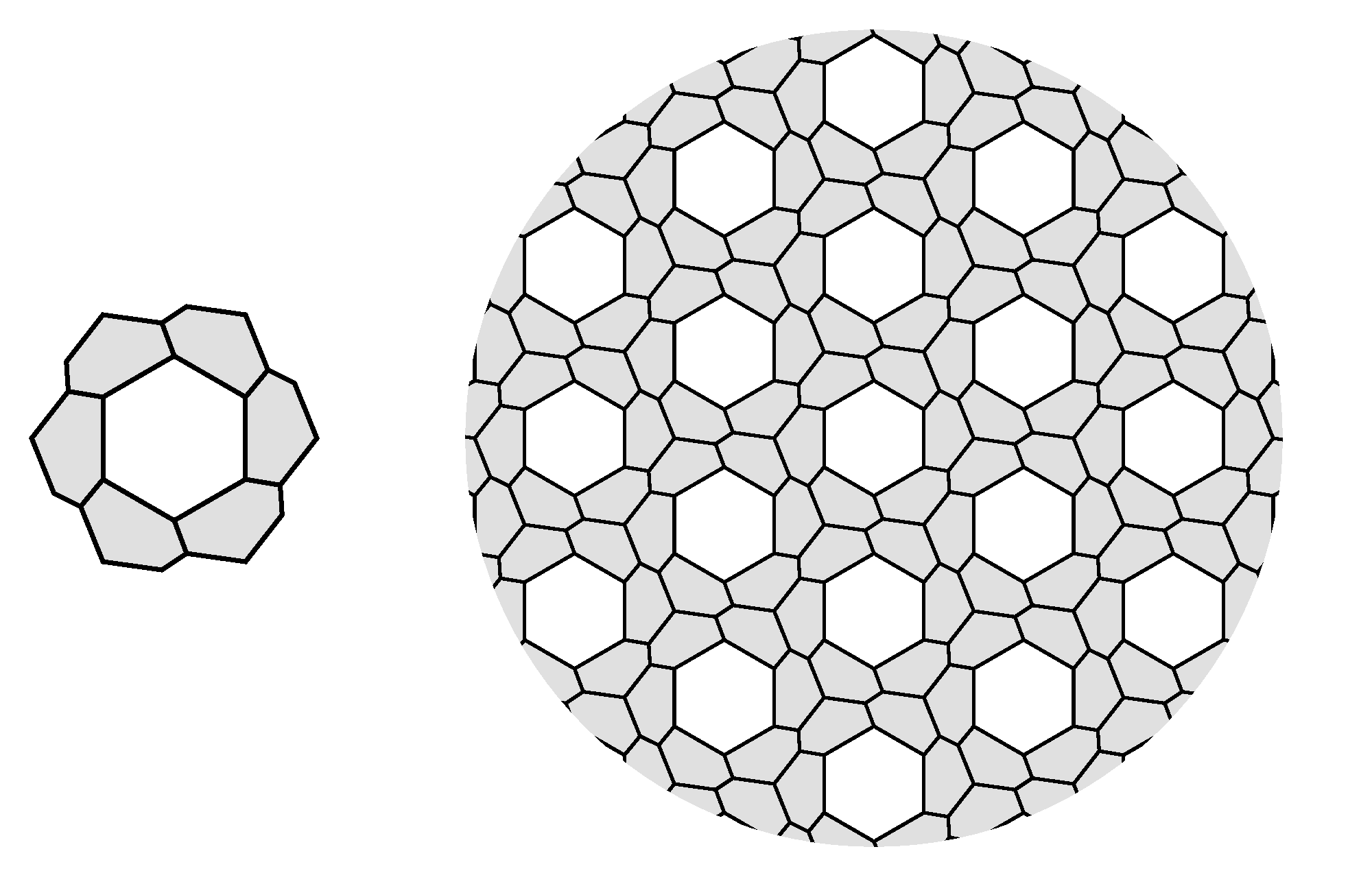
Hence $K$ tiles $R$, as seen in the second part, and therefore
$K$ perfectly $2$-covers the plane. Compared with the pentagons $K_\epsilon$,
this variation has the advantage not just of an extra parameter but also
that it's much easier to check there's no perfect $1$-covering:
If a convex hexagon tiles the plane then we can assume that
any two adjacent tiles must share a complete edge,
and that no more than three tiles meet in every corner;
and that's soon seen to be impossible here.