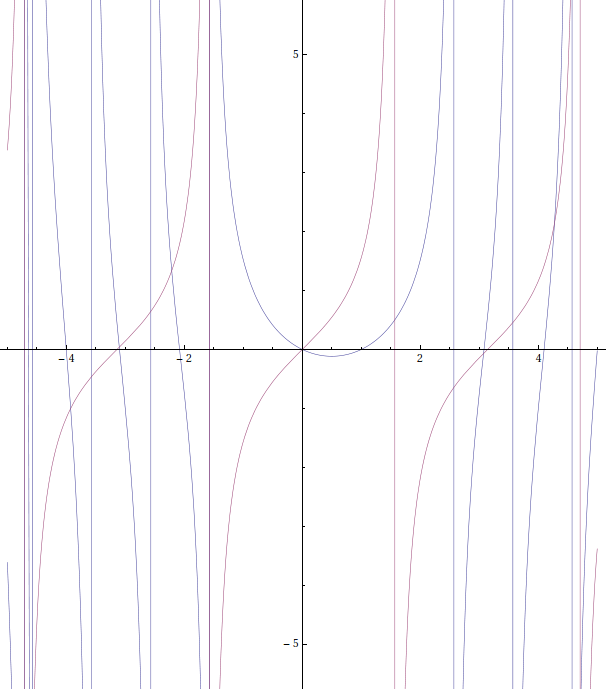
What is the indefinite sum of the tangent function, that is, the function $T$ for which
$\Delta_x T = T(x + 1) - T(x) = \tan(x)$
Of course, there are infinitely many answers, who all differ by a function of period 1. Ideally, I would like the solution to be of the form
$T(x) = $ nice_function$(x)$ + possibly_ugly_periodic_function$(x)$, where nice is at least piece-wise continuous.
If any of the following sums can be found, the sum of tan can also be found:
$\sum \sec x$
$\sum \csc x$
$\sum \cot x$
$\sum \frac{1}{e^{ix} + 1}$
I have tried several methods without success, including using a newton series (which does not converge for non-integer $x$), and trying to guess possible functions.
I would also appreciate lines of attack if a solution is not known.