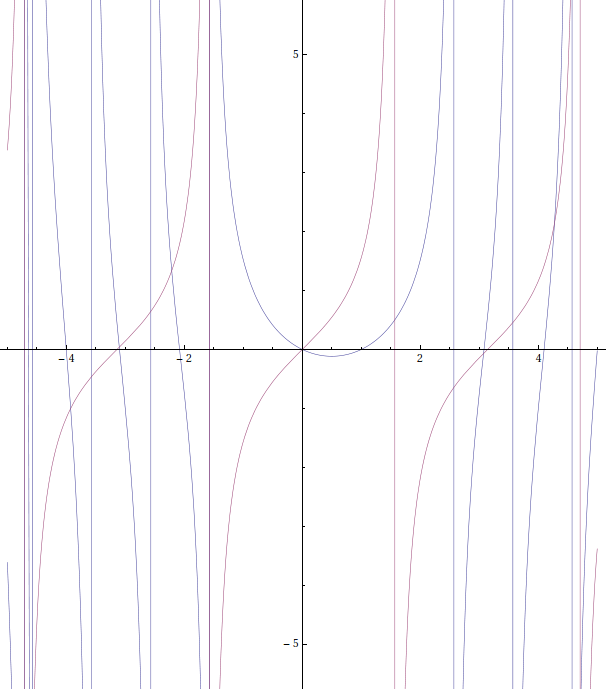
And here is the plot of indefinite sum of tan(x):
Here you can see tan(x) in red and its indefinite sum is in blue.
As you can see, the indefinite sum is fairly continuous. Oleg Eroshkin's conclusion that this function should be discontinuous everywhere apparently came from a false assumption that indefinite sum of a periodic function should also be periodic.
Though it is true that as $|x|$ grows the density of the poles grows, showing the same behavior as in function $f(x)=\tan(x^2)$
The function shown on this plot is
$$T(z)=-\sum _{k=1}^{\infty } \left(\psi \left(k \pi -\frac{\pi }{2}+1-z\right)+\psi \left(k \pi -\frac{\pi }{2}+z\right)-\psi \left(k \pi -\frac{\pi }{2}+1\right)-\psi \left(k \pi -\frac{\pi }{2}\right)\right)+C$$
It can be derived from the first formula on this page:
$$\tan(z)=8z \sum_{k=1}^{\infty} \frac1{(2k-1)^2\pi^2-4z^2}$$$$\tan(x)=8x \sum_{k=1}^{\infty} \frac1{(2k-1)^2\pi^2-4x^2}$$
We notice that there is a difference of squares in the denominator and separate the terms so to obtain
$$\tan(x)=-\sum_{k=1}^{\infty}\left(\frac1{z-\pi k+\frac{\pi}2}+\frac1{z+\pi k-\frac{\pi}2}\right)$$$$\tan(x)=-\sum_{k=1}^{\infty}\left(\frac1{x-\pi k+\frac{\pi}2}+\frac1{x+\pi k-\frac{\pi}2}\right)$$
Now we take indefinite sum by each term to obtain the expression for T(x). All simple.