Introduction
I have been in search of methods of assinging values to divergent series that have a nice intuitive or geometric interpretation. One fairly straightforward method I've considered for alternating series is to split the sum into two parts, and add those parts after 'aligning' them. Symbolically this equates to: $$\sum_{n=0}^x (-1)^n f(n) = \sum_{n=0}^\frac{x}{2} (-1)^{2n}f(2n) + \sum_{n=0}^\frac{x-1}{2} (-1)^{2n+1} f(2n+1) =\sum_{n=0}^\frac{x}{2}f(2n)-\sum_{n=0}^\frac{x-1}{2}f(2n+1)$$
The intuition for this method is that if $f(n)$ is function that doesn't favor the even or odds more (i.e. its not something like $\cos^2(\frac{\pi n}{2})$), it seems like $\sum_{n=0}^\infty(-1)^n f(n)$ should about cancel itself out. However, what stops this cancellation from happening is that we are subtracting the function from itself unevenly. In particular, to sum we do $(f(0) - f(1)) + (f(2) - f(3)) + \dots$. But this gives the negative side more time to grow. In each case, the negative term occurs later than the positive term. To remedy this, we could instead make sure the positive and negative terms have equal time to grow. We can write: $$\phi_+(N) = \sum_{n=0}^{N} f(2n)$$ $$\phi_-(N) = -\sum_{n=0}^{N} f(2n+1)$$ To align these chunks, we want them to end at the same point. So our sum should be $\phi_+(\frac{x}{2}) + \phi_-(\frac{x-1}{2})$, so that they both end at $f(x)$. Of course, the clear issue becomes: how do we take partial sums at fractional values?--for something like $\sum_{n=0}^{\frac{1}{2}} f(2n)$ does not make much sense on its own.
Extending Partial Sums
There are at least a few options for defining partial sums at non-integer values. One approach can be found here: (https://arxiv.org/pdf/math/0502109.pdf), where the general idea is to extend partial sums using approximate polynomials. Another strategy is to use Newton series, which is guaranteed to be unique under some assumptions using Carlson's theorem. Another option is to use the Euler-Maclaurin formula, but we need to require that the error term goes to zero for this one to work. In each of these cases, however, the approach will fail if the growth rate of the function becomes too large.
There, the heart of my question centers around finding a formal way to define and approximate the 'right' way to extend partial sums, irrespective of the growth rate.
At the end, I've included what the right way to extend partial sums is in a few examples where it's possible to define exactly the correct way to extend partial sums. The number of examples is somewhat overkill, and each should be self-contained, so they are mainly there for reference.
Proposed methods to approximate $\phi$
Since we wish to continue partial sums, one reasonable definition to start with is: $$\phi(x+1) = \phi(x) + f(x+1)$$ With $\phi(0) = f(0)$. $\phi$ will agree at integers if this definition is satisfied. However, this still allows us to choose the behavior at (0,1) arbitrarily, and then extend it outwards. We can somewhat constrain the space of functions by adding the requirement that $\phi(x)$ is infinitely differentiable and analytic, however, this is not sufficient to give a unique value for $\phi(x)$.
The primary method I have been investigating is to approximate $f$ using the truncated taylor series. For instance, rewriting the equation as $$\Delta \phi(x) = \sum_{n=0}^N \frac{f'(0)}{n!} x^n$$. This gives a unique $N+1$ degree polynomial for $\phi(x)$. However, the later terms have a larger and larger impact on $\phi(x)$, so this method doesn't quite converge. Yet, it interestingly seems that increasing $N$ seems to simply add in a $\alpha (\cos(2\pi x-\beta) -\cos(2\pi \beta))$. So, it seems that, at least in general, this method gives the correct value, but off by this cosine term. Perhaps there is some way to find the right value for this term using some harmonic series approach since we could look only at the function within (0,1), and so the question of finding the right way to define $\phi$ reduces to finding the right coefficients for $\alpha \cos(2 \pi x)$ and $\beta \sin(2 \pi x)$. Either way, finding the right value for the coefficients requires creating a notion of what the 'right'.
So, my question is: How should $\phi$ be defined? What properties should be required of $\phi$ so that it is uniquely defined?
Examples
Eta function and polynomials
Let's look at the example $\sum_{n=0}^\infty (-1)^n$. This splits into $$\sum_{n=0}^{\frac{x}{2}} 1-\sum_{n=0}^{\frac{x-1}{2}} 1 = \frac{x}{2} - \frac{x-1}{2} = \frac{1}{2} = 1-\eta(0)$$ Then $$\sum_{n=0}^\infty n(-1)^n = \sum_{n=0}^{\frac{x}{2}} 2n - \sum_{n=0}^{\frac{x-1}{2}} 2n+1 = \frac{x(x-2)}{4} - \frac{(x-1)^2}{4} = \frac{-1}{4}= -\eta(-1)$$
Likewise $$\sum_{n=0}^\infty n^2(-1)^n = \sum_{n=0}^{\frac{x}{2}} 4n^2 - \sum_{n=0}^{\frac{x-1}{2}} (2n+1)^2 = \frac{x(\frac{x}{2}+1)(x+1)}{3} - \frac{(\frac{x+1}{2})(x)(x+2)}{3} = 0= -\eta(-2)$$
Use Faulhaber's formula, it can be shown that this will get the correct value for $\eta(-n)$ for any natural number. So interestingly, the correct value to assign to polynomial is the simplest (lowest degree) polynomial which interpolates the points.
Geometric series We are looking at the sum $\sum_{n=0}^\infty (-1)^n x^n$. Splitting into two parts gives $$\sum_{n=0}^{\frac{N}{2}}x^{2n} -\sum_{n=0}^{\frac{N}{2}}x^{2n} = \frac{x^2(x^N-1)}{x^2-1} - x\left(\frac{x^2(x^{N-1}-1)}{x^2-1} + 1 \right) = \frac{1}{1+x}$$
It seems that again the correct partial sum is sort of the simplest function that agrees at the integers.
Alternating series with 0 radii of convergence This divergent series summation method is particularly interesting in that it can sum alternating divergent series regardless of their growth rates. On a number of important series, I've either asked questions or found others' questions to see that my methods agree. A short list of a few important ones includes:
- https://math.stackexchange.com/q/311583/709559 $\sum_{n=0}^\infty (n!)^k x^n$
- Value of divergent sum $\sum_{n=0}^\infty (-1)^n n^n$ $\sum_{n=0}^\infty (n^n) x^n$
- http://go.helms-net.de/math/tetdocs/Tetra_Etaseries.pdf Gottfried's tetra eta function: $\sum_{n=1}^\infty (-1)^{n-1} n^{n^x} $
- https://math.stackexchange.com/q/4219281/709559 $\sum_{n=0}^\infty (\alpha n)! x^n$
In each case, the partial sums appear to still be the 'simplest' interpolating function. In order, the correct interpolating function for different functions is shown below
$\sum_{n=0}^\infty (n!)^2 (-1/2)^n$------------$\sum_{n=0}^\infty (n^n) (-1/3)^n$------------$\sum_{n=0}^\infty (-1)^{n} (n+1)^{(n+1)^{\frac{1}{5}}} $

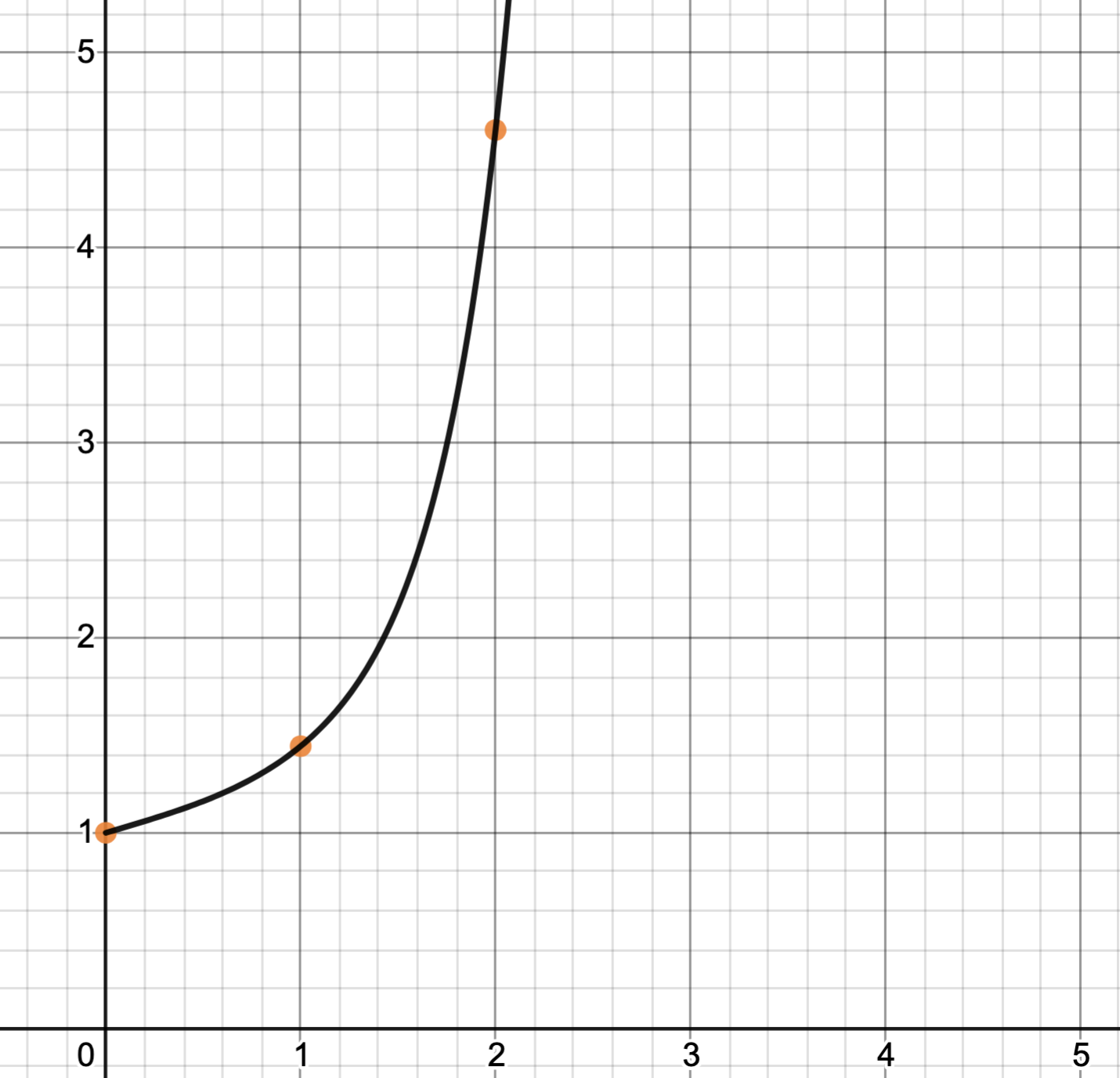
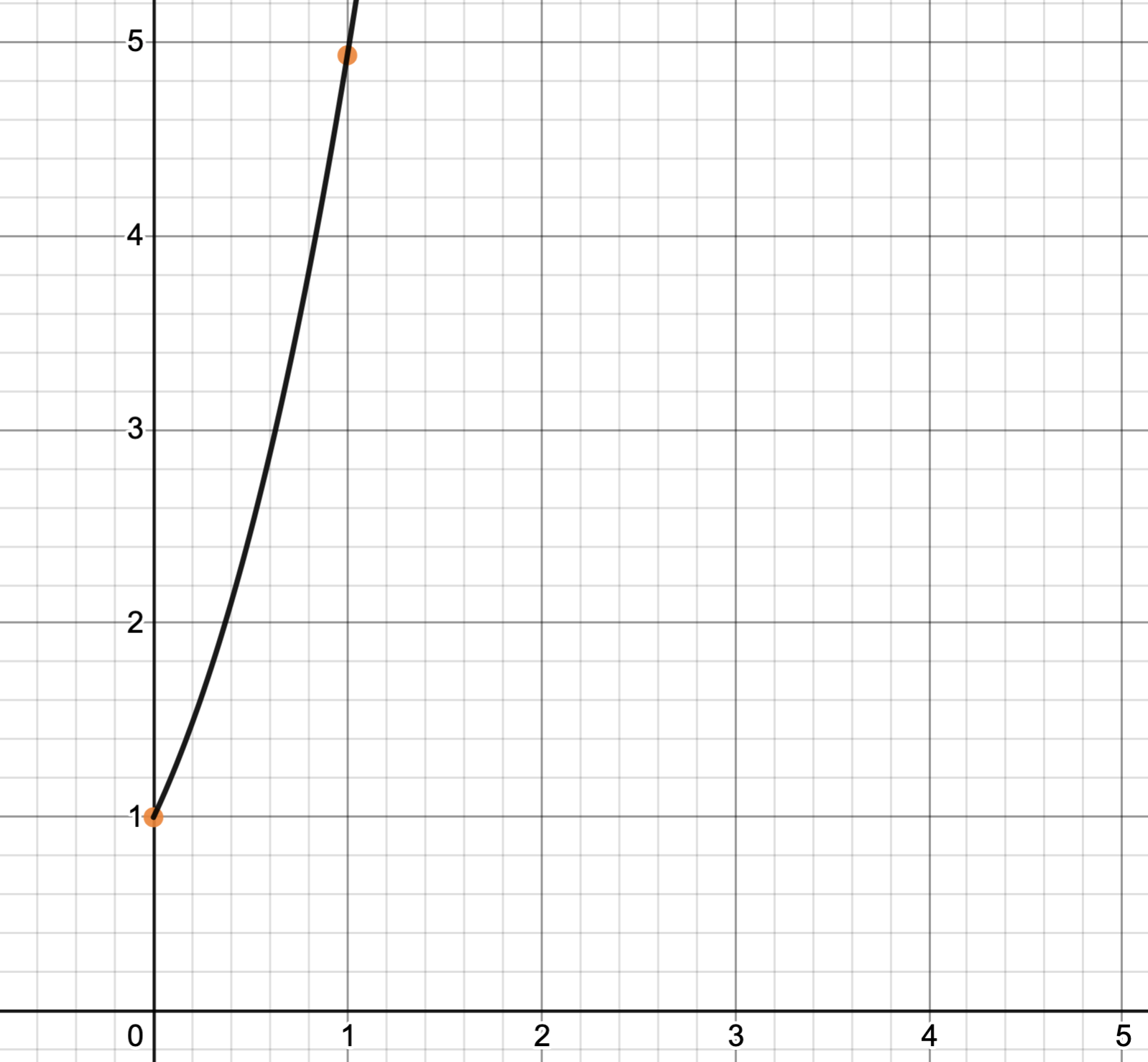
In each image, the black line is the interpolating sum for the positive part, and the orange dots are the partial sums.
An example of a less simple interpolating function for $\sum_{n=0}^\infty (-1)^{n} (n+1)^{(n+1)^{\frac{1}{5}}} $is this: 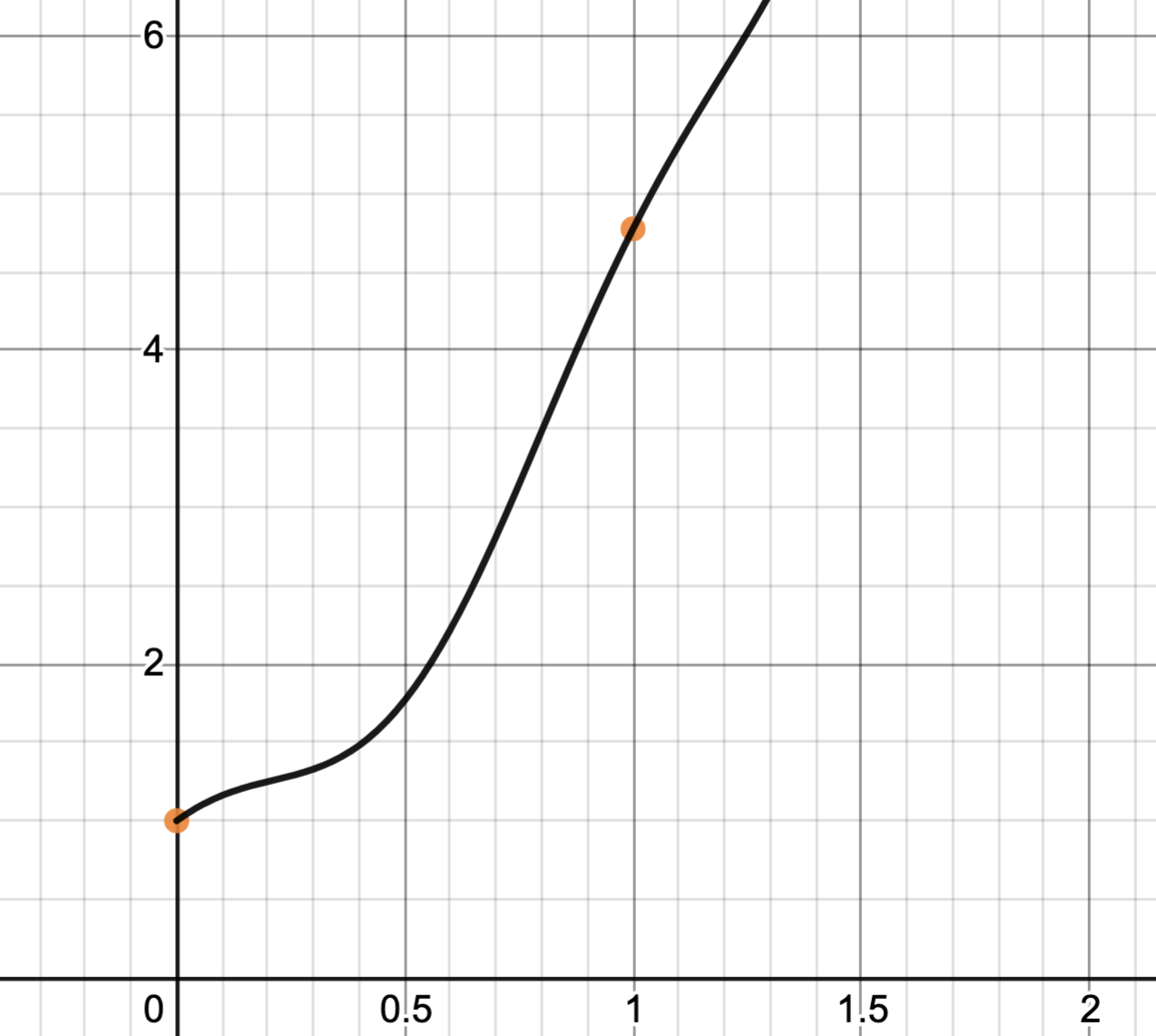
which gives the incorrect value when this interpolating function is assumed to be $\phi$.
As a final thought, I have usually been comparing the 'right' value to the value that other methods give, though a slightly more concrete requirement I often use is that if the series is a formal series to a differential equation, its regularization should solve the differential equation. For some of the series above, I have found the corresponding differential equations which can be transformed into the original equation: $$f'-2xf + 1 = 0 \to \sum_{n=0}^\infty \frac{(-1)^n}{2\sqrt{\pi}}\left(n-\frac{1}{2}\right)! x^{-(2n+1)} $$ $$f'' - \frac{1}{x}f + \frac{1}{x^2} = 0 \to \sum_{n=1}^\infty (n-1)!^2 n x^{-n} $$ $$f^{(k)} + (-1)^{k}f-\frac{(-1)^k}{x} = 0 \to \sum_{n=0}^\infty (kn)! (-1)^n x^{-(kn+1)}, k \in \mathbb{N}$$ $$ \frac{ds}{dx} = \frac{ (x-s)}{x^2} \to \sum_{n=0}^\infty (-1)^n n! x^{n+1}$$ (this last one is taken from https://math.stackexchange.com/questions/1832501/the-divergent-sum-of-alternating-factorials). Though, I suspect that thinking of divergent series as simply tools that solve differential equations is too narrow since I suspect there is some greater truth lurking beyond all of this.
Finally, I'm not sure if this question is well suited for mathoverflow, as it is full of unrefined ideas that aren't yet at the point of rigor. If this is too off-topic, feel free to close this.
EDIT: I was considering some ways to get exact equations for the partial sums of some of those 0 radii of convergence functions. One way we can get the partial sums of the geometric series is to notice that $$S_n = \sum_{k=0}^n x^k \implies xS_n - S_n = x^{n+1}-1 \implies S_n = \frac{x^{n+1}-1}{x-1}$$ However, since we have removed the summation, $S_n$ depends on something continuous and so we might expect $S_n$ to agree with the calculated partial sum even at non-integer values.
Since we already have a differential equation representation for many of our sums, it struck me that we can do something similar to obtain exact forms for the partial sum formula. In the case of $S_N = \sum_{n=1}^N (n!)^2 (n+1)x^{-n}$, we have the differential equation $$\frac{d^2}{dx^2}S_N - \frac{1}{x}S_N + \frac{1}{x^2} = (N!)^2 (N+1) x^{-(N+2)}$$
Letting Mathematica solve this gives something in terms of the Meijer-G and Bessel functions. Interestingly though, this does very closely agree with the approximations of the partial sums. However, I think this strategy can't work in general, since finding the differential equation that the function solves is probably not possible for all functions. However, it still might be possible that there is an approximation method that finds approximate differential equations, though I don't have a good idea for how to do this.
