Wonderful images, Edmund! :-)
A net $P$ can fold to a polyhedron iff there exists what I called in the book you cite an Alexandrov gluing, which is
an identification of its boundary points
that satisfies the three conditions of Alexandrov's theorem
[the subject of a recent MO question]:
(1) The identifications (or "gluings'') close up the perimeter of $P$ without gaps or overlaps;
(2) The resulting surface is homeomorphic to a sphere; and
(3) Identifications result in $\le 2 \pi$ surface angle glued at every point.
Under these three conditions, Alexandrov's theorem guarantees that the
folding produces a convex polyhedron, unique once the gluing is specified.
Let me quote two results from the book, informally, the first quite disappointingly negative, the second
compensatingly positive:
Theorem 25.1.2 (p.382): The probability that a random net of $n$ vertices can fold to a convex polyhedron
goes to $0$ as $n \to \infty$.
Theorem 25.1.4 (p.383): Every convex polygon folds to an uncountably infinite variety of incongruent convex polyhedra.
In particular, for example, a square folds to an infinite number of convex polyhedra, whose space
consists of six interlocked continuua, as detailed in our book (Fig.25.43,p.416).
The exact question you pose—Which nets can fold to convex polyhedra?—remains open.
Although if you give me a specific net, we have an algorithm that will produce all the convex
polyhedra to which it may fold.
But note my emphasis on "convex," an adjective you left out in your question.
That is Open Problem 25.1 in our book (p.384), on which topic I have written a separate
note subsequent to the book's publication: "On Folding a Polygon to a Polyhedron."
In a nutshell: every polygon folds to some (generally) nonconvex polyhedron,
by a result of Burago and Zalgaller. But their proof is complex enough that I have no understanding
what that polyhedron might look like.
Aside from the paper I cite above, you may be interested in this result:
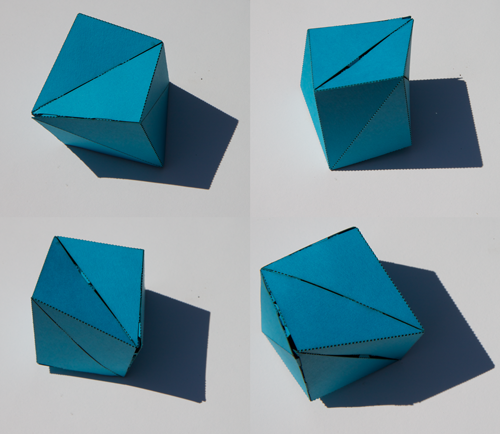
Four of the five Platonic solids may be "unzipped" and "rezipped" to be doubly covered
parallelograms (which may conveniently be placed in your wallet!). See this MSE question for
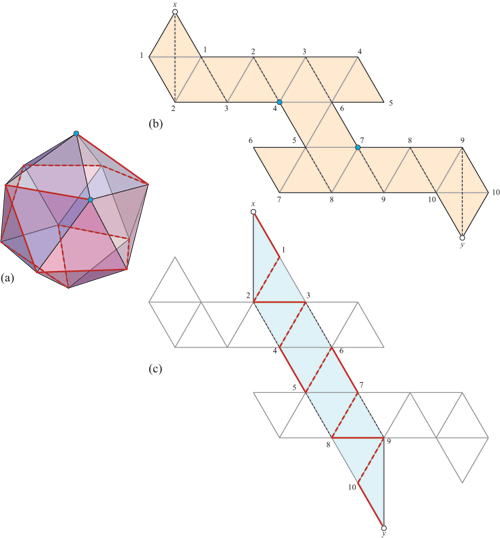
the (difficult to find) icosahedron net [below]. The holdout here is the
dodecahedron, whose 43,380 edge unfoldings each may only fold back to the dodecahedron.