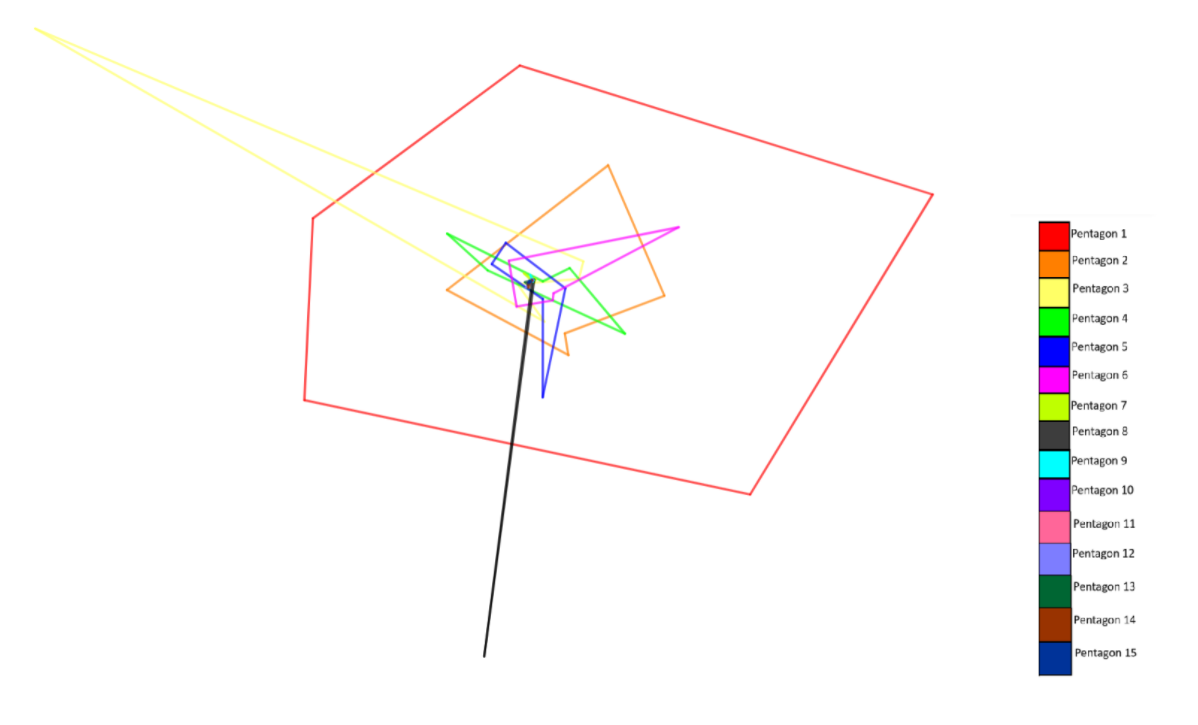
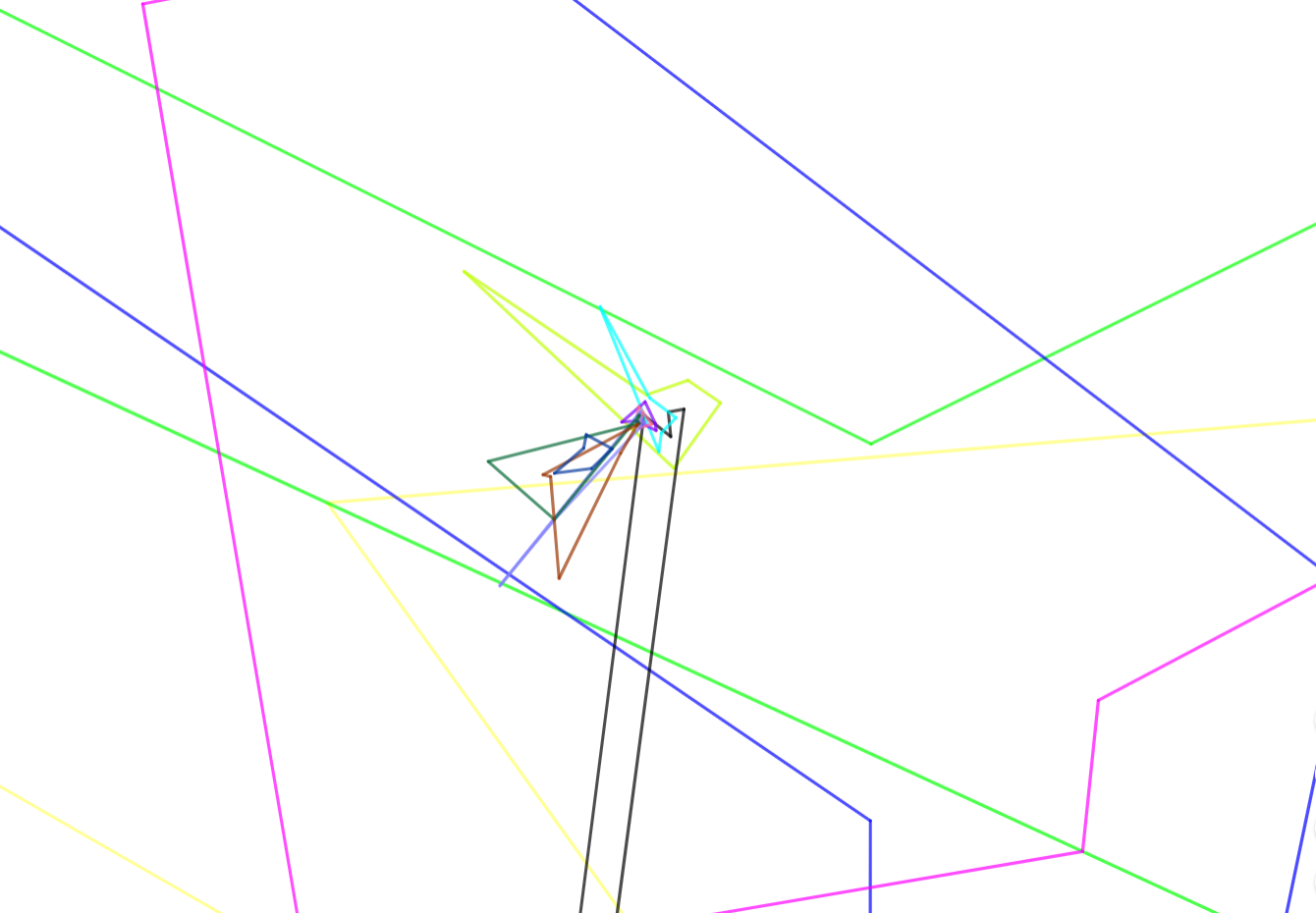
I asked this question on MSE here.

Given a non-regular pentagon $A_1B_1C_1D_1E_1$ with no two adjacent angle having a sum of 360 degrees, from the pentagon $A_nB_nC_nD_nE_n$ construct the pentagon $A_{n+1}B_{n+1}C_{n+1}D_{n+1}E_{n+1}$ as follows:

• $A_{n+1}$ is the intersection between the angle bisector of $\angle C_n $ and $\angle D_n$.
• $B_{n+1}$ is the intersection between the angle bisectors of $\angle D_n$ and $\angle E_n$.
• $C_{n+1}$ is the intersection between the angle bisectors of $\angle E_n$ and $\angle A_n$.
• $D_{n+1}$ is the intersection between the angle bisectors of $\angle A_n$ and $\angle B_n$.
• $E_{n+1}$ is the intersection between the angle bisectors of $\angle B_n$ and $\angle C_n$.

(the two opposite angles)

The reason why I chose this construction is that the point $A_{n+1}$ is the only point that doesn't depend on $A_n$. I am allowing self-intersecting polygons in this constructions.

My question is if this process is repeated indefinitely would the sequences ${A_n}$ , ${B_n}$ , ${C_n}$ , $D_n $ and $E_n$ converge ?

There are only four possible scenarios:

1. The points will converge.
2. The points will eventually trapped on a loop.
3. The points will diverge completely.
4. Two or more points coincide, or two adjacent angle have a sum of 360 degrees ending the sequence.

I tried to draw the first few pentagons to see if the point will converge or not.

Here is the first 50 pentagons in a zoom-in animation:

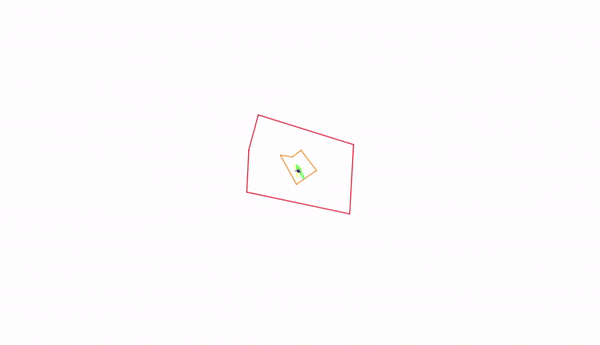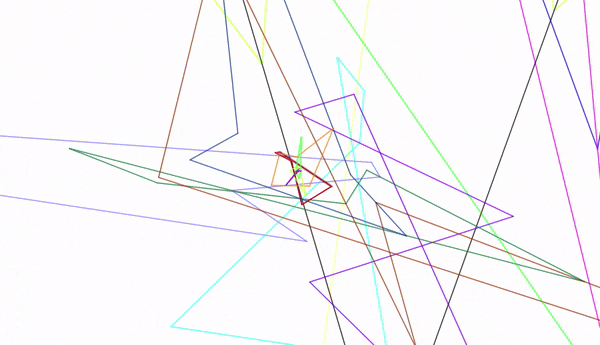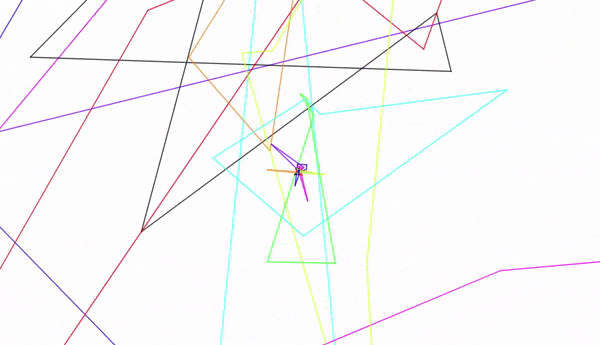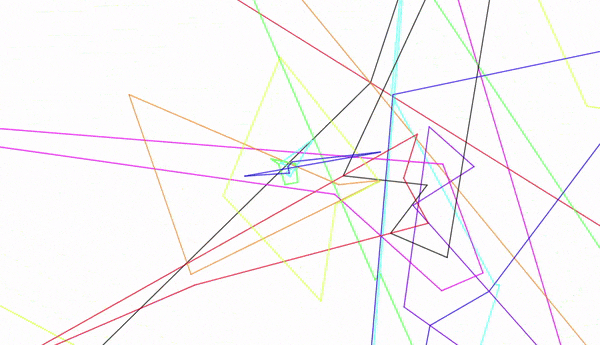
I conjecture that the sequences converge to a single point for all non-regular pentagons. If convergence occurs, how can we determine the limit point based on the initial pentagon? If the limit point exist what interesting properties does it have for the pentagon?

Here is a Geogebra file that have the first 50 pentagons.