Is it true that $$f(x):=\sum_{j=1}^\infty(-1)^j j^2 x^{j^2}\to0$$ as $x\uparrow1$?
(One may note that $f(x)=xh'(x)$, where $h(x):=\vartheta _4(0,x)/2$ and $\vartheta _4$ is a theta function, so that $f(1-)=h'(1-)$.)
Here is the graph $\{(x,f(x)):0.7<x<1\}$:
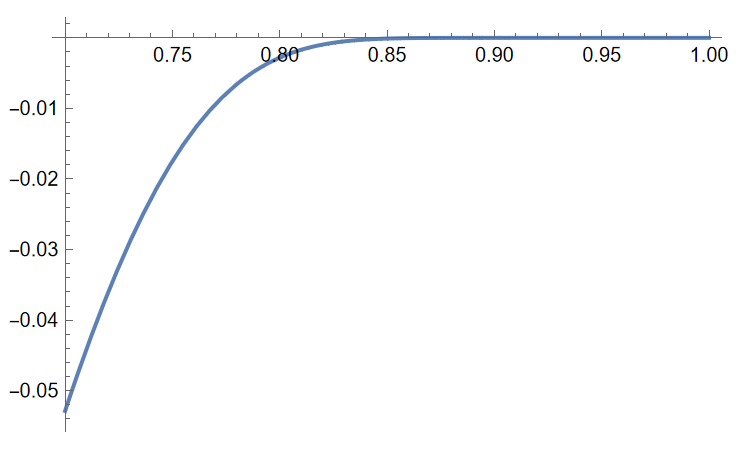
Writing $x=e^{-a}$ for $a>0$ and then using the Poisson summation formula with $s(u)=e^{i\pi u} u^2 e^{-au^2}$ (in the notation of the Wikipedia article), we rewrite the sum in question as $$G(a):=\frac12\,\sum_{k=-\infty}^\infty g_a(k),$$ where $$g_a(t):=\int_{-\infty}^\infty s(u)e^{-2\pi itu}\,du =\frac{\sqrt{\pi } \left(2 a-\pi ^2 (1-2 t)^2\right)}{4 a^{5/2}}\, e^{-\pi ^2 (1-2 t)^2/(4 a)}.$$ Letting now $a\downarrow0$ (which corresponds to $x\uparrow1$) and using dominated convergence, we get $G(0+)=0$. $\quad\Box$
It also follows that the convergence of $G(a)$ to $0$ as $a\downarrow0$ (or, equivalently, as $x\uparrow1$) is very fast, faster than that of any power of $a$ (or, equivalently, than that of any power of $1-x$). This corresponds to what we see in the picture in the OP.