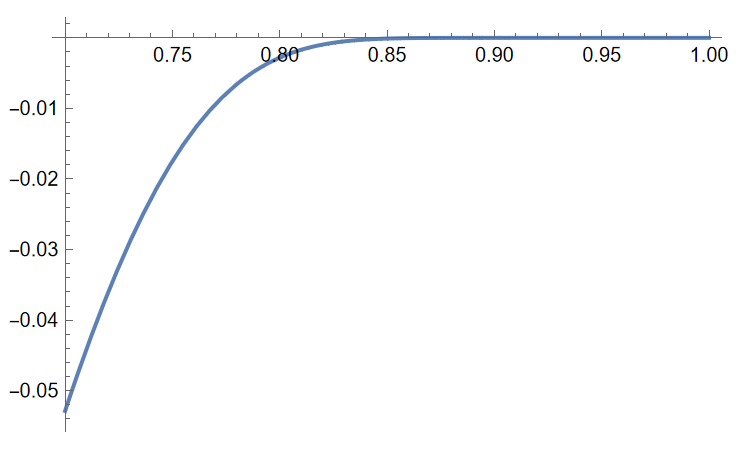
Is it true that $$f(x):=\sum_{j=1}^\infty(-1)^j j^2 x^{j^2}\to0$$ as $x\uparrow1$?
(One may note that $f(x)=xh'(x)$, where $h(x):=\vartheta _4(0,x)/2$ and $\vartheta _4$ is a theta function, so that $f(1-)=h'(1-)$.)
Here is the graph $\{(x,f(x)):0.7<x<1\}$: