Difference between parallel transport and ambient projection

Question

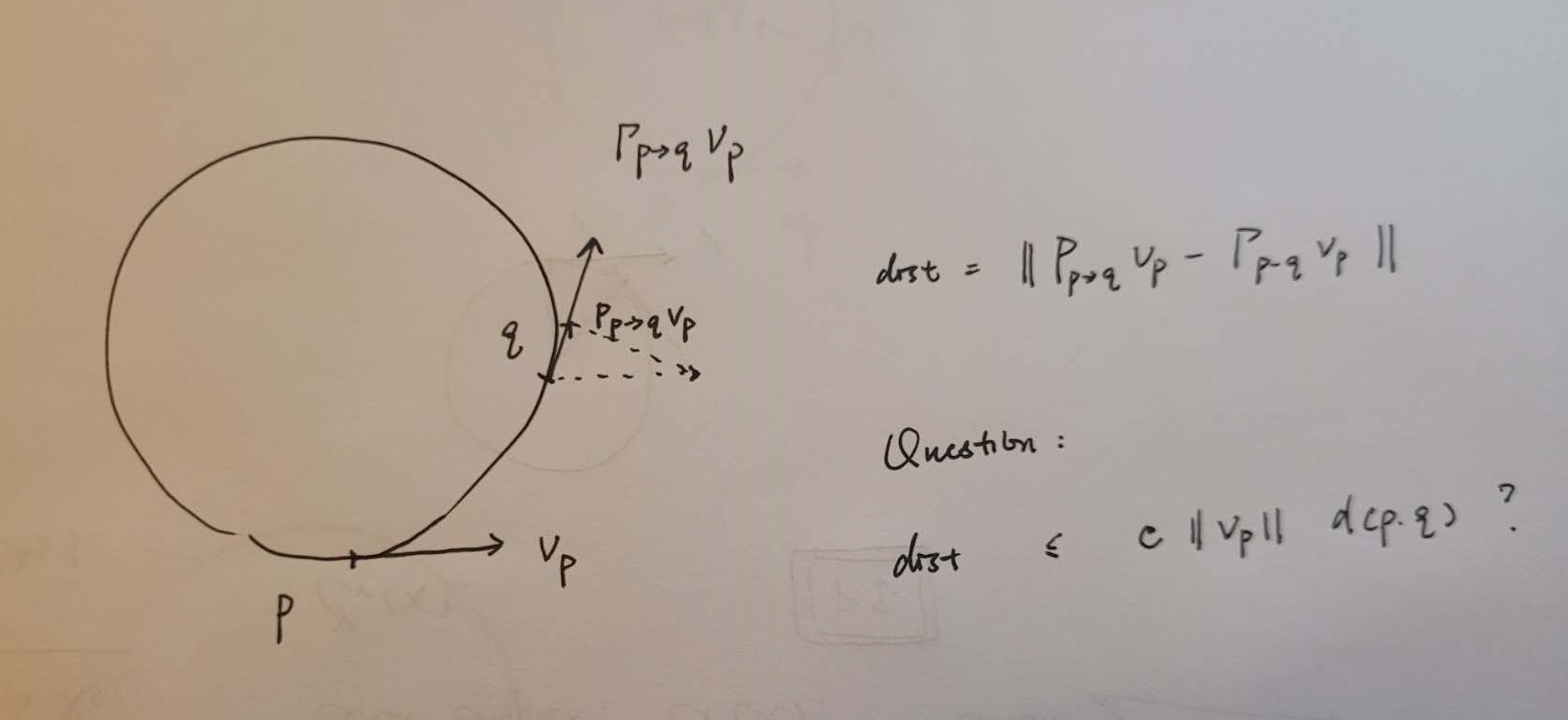
Consider a $d$-dimensional complete embedded Riemannian submanifold $(M,g)$ of a Euclidean space $\mathbb{R}^D$ (The major examples we consider are sphere and Stiefel manifold). Assume the sectional curvatures of $M$ are bounded within $[\kappa_{\min},\kappa_{\max}]$. Let $p,q\in M$ be two points in $M$ and $v_p\in T_pM$ be a tangent vector. Define the following distance: $$ {\rm dist}(p,q,v_p)=\|\Gamma_{p\to q}v_p-P_{p\to q}v_p\|, $$ where $\Gamma_{p\to q}:T_{p}M\to T_{q}M$ is the parallel transport along the shortest geodesic between $p$ and $q$, and $P_{p\to q}:T_pM\subseteq\mathbb{R}^D\to T_qM\subseteq\mathbb{R}^D$ is the projection to $T_qM$ in the ambient space $\mathbb{R}^D$. In other words, $P_{p\to q}v=P_{T_qM}v$ is the orthogonal projection of $v\in\mathbb{R}^{D}$ to $T_qM$. Notice that $\Gamma_{p\to q}$ is an intrinsic concept while $P_{p\to q}$ is an extrinsic concept.

Our question is whether the following inequality holds: $$ {\rm dist}(p,q,v_p)\leq c\|v_p\|d(p,q),\quad \forall p,q\in M,\forall v_p\in T_{p}M, $$ where $c$ is a constant independent of $p,q,$ and $v_p$. Any reference or comment is appreciated.

The intuition of this question is to demonstrate that the parallel transport and the projection mapping are close when $p$ and $q$ are close. We expect that this argument holds in a certain uniform sense so the constant $c$ should be independent of $p,q,$ and $v_p$.

Answer 1

This is false as stated. Take a surface of revolution generated by $(r(t),z(t))$. I claim I can choose the curve so that there are pieces that look like $(e^{-j},t)$ for $j$ large. The point is that if the curve is parametrized by arc length then the (intrinsic) Gaussian curvature is $-r''(t)/r(t)$. Let $r(t)=e^{-u(t)}$ so the Gaussian curvature becomes $-u''(t)-u'(t)^2$. Now it's easy to draw a $u(t)$ interpolating between regions where $u(t)=j$ but with bounded first and second derivative.

However in the cylindrical regions of radius $\rho\ll1$ you can go around the cylinder a distance $\pi\rho/2$. A tangent vector is parallel transported by angle $\pi/2$ but extrinsically projected to $0$. Thus the asserted inequality would read $$ 1\leq O(\rho) $$ Which cannot hold.

---

To prove a correct statement, let's upgrade the assumption of bounded sectional curvature to bounded second fundamental form (this implies bounded sectional curvature by the Gauss equations).

Consider a minimizing geodesic $\gamma$ from $p$ to $q$ of length $l$. Let $v(t)$ denote the parallel transport of $v$ (assumed to be unit vector) along $\gamma$, namely $$ (v'(t))^\top=0 $$ where this is projection to tangent space. Also write $v=v^\perp(t)+v\top(t)$.

Take $Z(t)$ (intrinsically) parallel along $\gamma$. Then $$ \langle (v^\perp)'(t),Z(t)\rangle = -\langle v^\perp(t),Z'(t)\rangle = -\langle v^\perp(t),II(\gamma'(t),Z(t))\rangle $$ Since $(v^\top)'=-(v^\perp)'$ we deduce that $$ |((v^\top)')^\top| \leq C $$ for $C$ that only depends on the second fundamental form bound.

Thus $|v^\top|'$ and $\langle v^\top(t),v(t)\rangle'$ are bounded by $C$. This shows that $$ |v(t)-v^\top(t)|'\leq C. $$ Integrate this along $\gamma$ to conclude that $$ |v(t)-v^\top(t)|\leq C |v|d(p,q) $$ (where we reinserted the norm of $v$).