Consider a $d$-dimensional complete embedded Riemannian submanifold $(M,g)$ of a Euclidean space $\mathbb{R}^D$. Assume the sectional curvatures of $M$ are bounded within $[\kappa_{\min},\kappa_{\max}]$. Let $p,q\in M$ be two points in $M$ and $v_p\in T_pM$ be a tangent vector. Define the following distance: $$ {\rm dist}(p,q,v_p)=\|\Gamma_{p\to q}v_p-P_{p\to q}v_p\|, $$ where $\Gamma_{p\to q}:T_{p}M\to T_{q}M$ is the parallel transport along the shortest geodesic between $p$ and $q$, and $P_{p\to q}:T_pM\subseteq\mathbb{R}^D\to T_qM\subseteq\mathbb{R}^D$ is the projection to $T_qM$ in the ambient space $\mathbb{R}^D$. In other words, $P_{p\to q}v=P_{T_qM}v$ is the orthogonal projection of $v\in\mathbb{R}^{D}$ to $T_qM$. Notice that $\Gamma_{p\to q}$ is an intrinsic concept while $P_{p\to q}$ is an extrinsic concept.

Our question is whether the following inequality holds: $$ {\rm dist}(p,q,v_p)\leq c\|v_p\|d(p,q),\quad \forall p,q\in M,\forall v_p\in T_{p}M, $$ where $c$ is a constant independent of $p,q,$ and $v_p$.

The intuition of this question is to demonstrate that the parallel transport and the projection mapping are close when $p$ and $q$ are close. We expect that this argument holds in a certain uniform sense so the constant $c$ should be independent of $p,q,$ and $v_p$.

Consider a $d$-dimensional complete embedded Riemannian submanifold $(M,g)$ of a Euclidean space $\mathbb{R}^D$ (The major examples we consider are sphere and Stiefel manifold). Assume the sectional curvatures of $M$ are bounded within $[\kappa_{\min},\kappa_{\max}]$. Let $p,q\in M$ be two points in $M$ and $v_p\in T_pM$ be a tangent vector. Define the following distance: $$ {\rm dist}(p,q,v_p)=\|\Gamma_{p\to q}v_p-P_{p\to q}v_p\|, $$ where $\Gamma_{p\to q}:T_{p}M\to T_{q}M$ is the parallel transport along the shortest geodesic between $p$ and $q$, and $P_{p\to q}:T_pM\subseteq\mathbb{R}^D\to T_qM\subseteq\mathbb{R}^D$ is the projection to $T_qM$ in the ambient space $\mathbb{R}^D$. In other words, $P_{p\to q}v=P_{T_qM}v$ is the orthogonal projection of $v\in\mathbb{R}^{D}$ to $T_qM$. Notice that $\Gamma_{p\to q}$ is an intrinsic concept while $P_{p\to q}$ is an extrinsic concept.

Our question is whether the following inequality holds: $$ {\rm dist}(p,q,v_p)\leq c\|v_p\|d(p,q),\quad \forall p,q\in M,\forall v_p\in T_{p}M, $$ where $c$ is a constant independent of $p,q,$ and $v_p$. Any reference or comment is appreciated.

The intuition of this question is to demonstrate that the parallel transport and the projection mapping are close when $p$ and $q$ are close. We expect that this argument holds in a certain uniform sense so the constant $c$ should be independent of $p,q,$ and $v_p$.

Consider a $d$-dimensional complete embedded Riemannian submanifold $(M,g)$ of a Euclidean space $\mathbb{R}^D$. Assume the sectional curvatures of $M$ are bounded within $[\kappa_{\min},\kappa_{\max}]$. Let $p,q\in M$ be two points in $M$ and $v_p\in T_pM$ be a tangent vector. Define the following distance: $$ {\rm dist}(p,q,v_p)=\|\Gamma_{p\to q}v_p-P_{p\to q}v_p\|, $$ where $\Gamma_{p\to q}:T_{p}M\to T_{q}M$ is the parallel transport along the shortest geodesic between $p$ and $q$, and $P_{p\to q}:T_pM\subseteq\mathbb{R}^D\to T_qM\subseteq\mathbb{R}^D$ is the projection to $T_qM$ in the ambient space $\mathbb{R}^D$. In other words, $P_{p\to q}v=P_{T_qM}v$ is the orthogonal projection of $v\in\mathbb{R}^{D}$ to $T_qM$. Notice that $\Gamma_{p\to q}$ is an intrinsic concept while $P_{p\to q}$ is an extrinsic concept.

Our question is whether the following inequality holds: $$ {\rm dist}(p,q,v_p)\leq c_0\|v_p\|d(p,q),\quad \forall p,q\in M,\forall v_p\in T_{p}M, $$ where $c$ is a constant independent of $p,q,$ and $v_p$.

The intuition of this question is to demonstrate that the parallel transport and the projection mapping are close when $p$ and $q$ are close. We expect that this argument holds in a certain uniform sense so the constant $c$ should be independent of $p,q,$ and $v_p$.

Consider a $d$-dimensional complete embedded Riemannian submanifold $(M,g)$ of a Euclidean space $\mathbb{R}^D$. Assume the sectional curvatures of $M$ are bounded within $[\kappa_{\min},\kappa_{\max}]$. Let $p,q\in M$ be two points in $M$ and $v_p\in T_pM$ be a tangent vector. Define the following distance: $$ {\rm dist}(p,q,v_p)=\|\Gamma_{p\to q}v_p-P_{p\to q}v_p\|, $$ where $\Gamma_{p\to q}:T_{p}M\to T_{q}M$ is the parallel transport along the shortest geodesic between $p$ and $q$, and $P_{p\to q}:T_pM\subseteq\mathbb{R}^D\to T_qM\subseteq\mathbb{R}^D$ is the projection to $T_qM$ in the ambient space $\mathbb{R}^D$. In other words, $P_{p\to q}v=P_{T_qM}v$ is the orthogonal projection of $v\in\mathbb{R}^{D}$ to $T_qM$. Notice that $\Gamma_{p\to q}$ is an intrinsic concept while $P_{p\to q}$ is an extrinsic concept.

Our question is whether the following inequality holds: $$ {\rm dist}(p,q,v_p)\leq c\|v_p\|d(p,q),\quad \forall p,q\in M,\forall v_p\in T_{p}M, $$ where $c$ is a constant independent of $p,q,$ and $v_p$.

The intuition of this question is to demonstrate that the parallel transport and the projection mapping are close when $p$ and $q$ are close. We expect that this argument holds in a certain uniform sense so the constant $c$ should be independent of $p,q,$ and $v_p$.

Let $M$ be a complete Riemannian manifold embedded in an Euclidean space (equipped with Euclidean induced metric). The main examples I'm considering are spheres, Stiefel manifold, etc.

What I'm trying to do is to conduct a certain error analysis for the projectional vector transport. Let's say $\mathbb{S}^{d}\subset\mathbb{R}^{d+1}$ be the $d$-dimensional unit sphere as an example here. Given $p\in\mathbb{S}^{d}$ and $\xi\in T_{p}\mathbb{S}^{d}$, suppose we use the projectional retraction (simply project the vector $p+\xi$ onto $\mathbb{S}^{d}$. For the case of Stiefel manifold, see [1]) and obtain a point $q=\text{retr}_{p}(\xi)=\frac{p+\xi}{\|p+\xi\|}\in\mathbb{S}^{d}$.

Now I'm considering the ways to transport vector $\xi$ from $T_p \mathbb{S}^{d}$ to $T_q \mathbb{S}^{d}$. Projectional vector transport (to project the vector $\xi$ onto the tangent space of $q$) yields $\eta=\xi-\langle\xi, q\rangle q\in T_q \mathbb{S}^{d}$. Another way to obtain a transported vector is to parallel transport $\xi$ onto $T_q \mathbb{S}^{d}$, yielding $P_{p\rightarrow q}\xi\in T_{q}\mathbb{S}^{d}$ (here I'm think of transporting along the minimal geodesic, basically yielding a vector with the same direction as $\eta$ and the length is the same as $\|\xi\|$, see [2] Chap 5).

Now a simple calculation shows that $$ \|P_{p\rightarrow q}\xi - \eta\|\leq \|\xi\| d(p,q) $$ where the left-hand side is the error between the parallel transported vector and the projected vector-transported vector (both are in $T_q \mathbb{S}^{d}$), and the right-hand side are the length of the vector $\xi$ and the geodesic distance from $p$ to $q$.

Question: Is this bound true only for the sphere ? Or it holds for other manifolds mentioned in [1], particularly, the Stiefel manifold?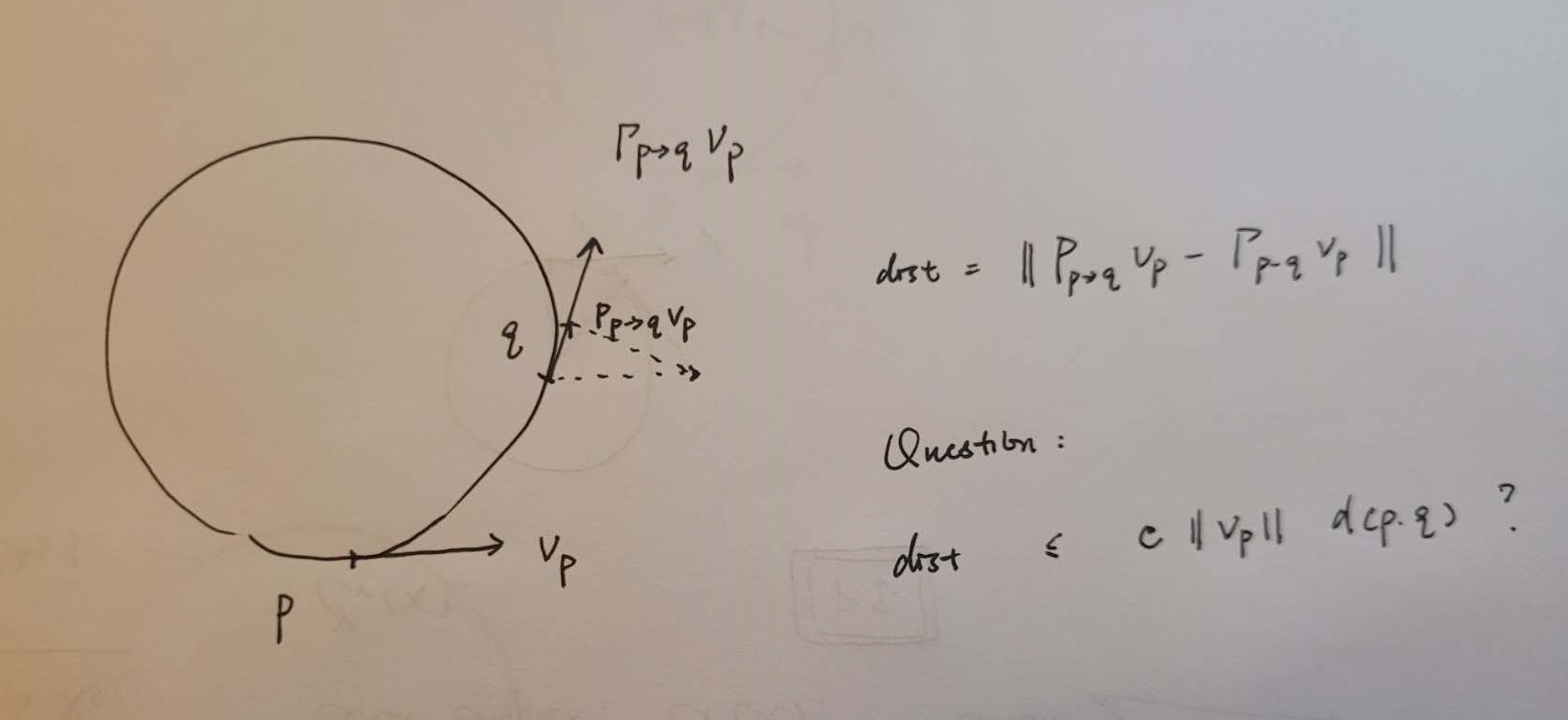

The difficulty seems to be in the lack of closed form solution for a parallel transport on Sitefel manifold (or other such matrix manifolds). Any help or pointers to related references are much appreciated.

References:

[1] Absil, P-A., and Jérôme Malick. "Projection-like retractions on matrix manifolds." SIAM Journal on Optimization 22.1 (2012): 135-158.

[2] Absil, P-A., Robert Mahony, and Rodolphe Sepulchre. Optimization algorithms on matrix manifolds. Princeton University Press, 2008.

Consider a $d$-dimensional complete embedded Riemannian submanifold $(M,g)$ of a Euclidean space $\mathbb{R}^D$. Assume the sectional curvatures of $M$ are bounded within $[\kappa_{\min},\kappa_{\max}]$. Let $p,q\in M$ be two points in $M$ and $v_p\in T_pM$ be a tangent vector. Define the following distance: $$ {\rm dist}(p,q,v_p)=\|\Gamma_{p\to q}v_p-P_{p\to q}v_p\|, $$ where $\Gamma_{p\to q}:T_{p}M\to T_{q}M$ is the parallel transport along the shortest geodesic between $p$ and $q$, and $P_{p\to q}:T_pM\subseteq\mathbb{R}^D\to T_qM\subseteq\mathbb{R}^D$ is the projection to $T_qM$ in the ambient space $\mathbb{R}^D$. In other words, $P_{p\to q}v=P_{T_qM}v$ is the orthogonal projection of $v\in\mathbb{R}^{D}$ to $T_qM$. Notice that $\Gamma_{p\to q}$ is an intrinsic concept while $P_{p\to q}$ is an extrinsic concept.

Our question is whether the following inequality holds: $$ {\rm dist}(p,q,v_p)\leq c_0\|v_p\|d(p,q),\quad \forall p,q\in M,\forall v_p\in T_{p}M, $$ where $c$ is a constant independent of $p,q,$ and $v_p$.

The intuition of this question is to demonstrate that the parallel transport and the projection mapping are close when $p$ and $q$ are close. We expect that this argument holds in a certain uniform sense so the constant $c$ should be independent of $p,q,$ and $v_p$.