Suppose $a,b$ are two positive-definite linear operators on (say) $\mathbb R^n$. For $p\in(0,1)$, do we then have $(a+b)^p\leq a^p+b^p$ (with respect to the Loewner order)?
-
1$\begingroup$ What do you mean by 'operator' here? And then what does the power mean? $\endgroup$– David Roberts ♦Commented Nov 1, 2022 at 12:02
-
2$\begingroup$ @DavidRoberts : I think "operator" here is supposed to mean "linear operator". The matrix functions (here, matrix power functions) can then be defined in a standard manner -- cf. e.g. nhigham.com/2020/06/09/what-is-a-matrix-function $\endgroup$– Iosif PinelisCommented Nov 1, 2022 at 13:45
-
2$\begingroup$ I am also curious what is meant by $\le$ relation on operators. Is it supposed to be a lexicographic order on matrices with respect to a certain fixed basis? $\endgroup$– Grisha TaroyanCommented Nov 1, 2022 at 13:46
-
1$\begingroup$ Please clarify your specific problem or provide additional details to highlight exactly what you need. As it's currently written, it's hard to tell exactly what you're asking. $\endgroup$– Community BotCommented Nov 1, 2022 at 13:47
-
2$\begingroup$ @GrishaTaroyan : Since positive operators were mentioned, it appears that the order in question is the Loewner order. I have taken the liberty to edit the post accordingly. $\endgroup$– Iosif PinelisCommented Nov 1, 2022 at 13:53
2 Answers
Iosif's example can be given a more conceptual description. Take $a=P$, $b=Q$ as projections. Then $P^p=P$, $Q^p=Q$, so the desired inequality becomes $$ (P+Q)^p \le P+Q . $$ Now $T^p\le T$, for $0<p<1$, is equivalent to $T$ having no eigenvalues in $(0,1)$. However, it's easy to give $P+Q$ an eigenvalue in this range.
For example, if $R(P),R(Q)$ span the whole space and there is a $v$ with $Pv=0$, $Qv\not= v$, then the smallest eigenvalue lies in $(0,1)$, by min-max.
-
2
-
3$\begingroup$ @IosifPinelis: Thank you, and sorry for misspelling your name (fixed now). $\endgroup$ Commented Nov 1, 2022 at 20:53
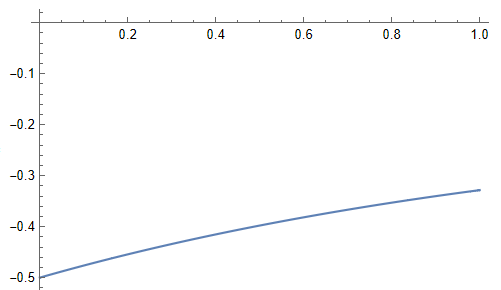
No. E.g., (identifying linear operators with matrices in a standard manner) let $$a=\left( \begin{array}{cc} 1 & 0 \\ 0 & 0 \\ \end{array} \right),\quad b=\frac12 \left( \begin{array}{cc} 1 & 1 \\ 1 & 1 \\ \end{array} \right). $$ Then (by straightforward but somewhat tedious calculations) for all $p\in(0,1)$ $$d(p):=\det(a^p+b^p-(a+b)^p) \\ =2^{-p-3/2} \\ \times\left(\sqrt{2} \left(2^p+2\right)-2 \left(\sqrt{2}-1\right) \left(2+\sqrt{2}\right)^p-2 \left(1+\sqrt{2}\right) \left(2-\sqrt{2}\right)^p\right)<0 \tag{1}\label{1},$$ and hence $(a+b)^p\not\le a^p+b^p$.
To prove the inequality in \eqref{1}, let
$$d_0(p):=d(p)2^{p+3/2},\quad
d_1(p):=\frac{d_0'(p)}{(2+\sqrt2)^p},\\
d_2(p):=d_1'(p)(1+1/\sqrt2)^p.
$$
Then
$$d_2'(p)=\left(1+\sqrt{2}\right) 2^{1-p} \left(2-\sqrt{2}\right)^p \ln\left(3-2
\sqrt{2}\right) \ln\left(2-\sqrt{2}\right) \ln\left(2+\sqrt{2}\right)>0,$$
$d_2$ is increasing (on $(0,1)$), $d_2(1)=\sqrt{2} \ln\left(2-\sqrt{2}\right) \ln\left(6+4 \sqrt{2}\right)<0$, $d_2<0$, $d_1$ is decreasing, $d_1(1)=0$, $d_1>0$, $d_0$ is increasing, $d_0(1)=0$, $d_0<0$, $d<0$. $\quad\Box$
Here is the graph $\{(p,d(p)(1-p)^{-2})\colon0<p<1\}$: